A uniform rope of length L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
√m1m2
√m1+m2m2
√m2m1
√m1+m2m1
The correct Answer is:B
To solve the problem, we need to find the ratio of the wavelengths of a transverse pulse at the bottom and top of a vertically hanging rope. Here’s a step-by-step breakdown of the solution:
Step 1: Understanding the System
We have a uniform rope of length L and mass m1 hanging vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. When a transverse pulse is generated at the lower end of the rope, it travels upwards.
Step 2: Analyzing Tension in the Rope
The tension in the rope varies along its length due to the weight of the rope and the block.
- At the bottom of the rope (where the pulse is generated), the tension T1 is due only to the weight of the block:
T1=m2⋅g
- At the top of the rope (where the rope is attached to the support), the tension T2 is due to the weight of both the rope and the block:
T2=(m1+m2)⋅g
Step 3: Relating Wavelength to Tension
The wavelength of a wave on a string is related to the tension in the string. Specifically, the wavelength λ is proportional to the square root of the tension T:
λ∝√T
Step 4: Setting Up the Ratio of Wavelengths
From the relationship above, we can express the ratio of the wavelengths at the top and bottom of the rope:
λ1λ2=√T1T2
Rearranging gives us:
λ2λ1=√T2T1
Step 5: Substituting the Tension Values
Now we substitute the expressions for T1 and T2:
λ2λ1=√(m1+m2)⋅gm2⋅g
The g cancels out:
λ2λ1=√m1+m2m2
Final Result
Thus, the ratio of the wavelengths is:
λ2λ1=√m1+m2m2
We have a uniform rope of length L and mass m1 hanging vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. When a transverse pulse is generated at the lower end of the rope, it travels upwards.
The tension in the rope varies along its length due to the weight of the rope and the block.
- At the bottom of the rope (where the pulse is generated), the tension T1 is due only to the weight of the block:
T1=m2⋅g
- At the top of the rope (where the rope is attached to the support), the tension T2 is due to the weight of both the rope and the block:
T2=(m1+m2)⋅g
The wavelength of a wave on a string is related to the tension in the string. Specifically, the wavelength λ is proportional to the square root of the tension T:
λ∝√T
From the relationship above, we can express the ratio of the wavelengths at the top and bottom of the rope:
λ1λ2=√T1T2
Rearranging gives us:
λ2λ1=√T2T1
Now we substitute the expressions for T1 and T2:
λ2λ1=√(m1+m2)⋅gm2⋅g
The g cancels out:
λ2λ1=√m1+m2m2
Thus, the ratio of the wavelengths is:
λ2λ1=√m1+m2m2
Topper's Solved these Questions
- WAVES AND ACOUSTICSBOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - Chapter Test
30 Videos
- WAVES AND ACOUSTICSBOOK - A2ZCHAPTER - WAVES AND ACOUSTICSEXERCISE - AIIMS Questions
55 Videos
- VECTORSBOOK - A2ZCHAPTER - VECTORSEXERCISE - Chapter Test
29 Videos
- WORK, ENERGY, POWER AND COLLISIONBOOK - A2ZCHAPTER - WORK, ENERGY, POWER AND COLLISIONEXERCISE - Chapter Test
29 Videos
Similar Questions
A uniform rope of elngth l and mass m hangs vertically from a rigid support. A block of mass m is attached to the free end of the rope. A transverse pulse of wavelength λ is produced at the lower end of the rope. The wavelength of the pulse, when it reaches the top of the rope. is aλ. Find a2

View SolutionA uniform rope of length 12m and mass 6kg hangs vertically from a rigid support. A block of mass 2kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06m is produced at the lower end of the rope. What is the wavelength of the pulse whwn it reaches the top of the rope?
View Solution
Knowledge Check
A uniform rope of legnth L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
A√m1+m2m1B√m1m2C√m1+m2m2D√m2m1A uniform rope of legnth L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
A√m1m2B√m1+m2m2C√m2m1D√m1+m2m1A uniform rope of length 10 m and mass 3 kg hangs vertically from a rigid support. A block of mass 1 kg is attached to the free end of the rople. A transverse pulse of wavelength 0.05 m is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is
A0.10 mB0.12 mC0.16 mD0.18 m
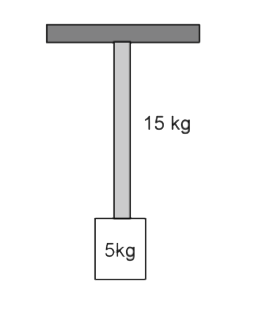
A uniform rope of length 10 m and mass 15 kg hangs vertically from a rigid support. A block of mass 5 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.08 m is produced at the lower end of the 3 rope. The wavelength of the pulse when it reaches the top of the rope will be-
View SolutionA uniform rope of length 20m and mass 8kg hangs vertically froma rigid support. A block of mass 2kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top os the rope?
View SolutionA uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 10 cm and mass 4 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope, what is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 12m and mass 6kg hangs vertically from a rigid support . A block of mass 2kg is attached to the free end of the rope . A transverse pulse of wavelengths 0.06m is produced at the lower end of the rope . What is the wavelength of the pulse when it reaches the top of the rope?
View Solution
