A uniform slab of dimension 10cm×10cm×1cm is kept between two heat reservoire at temperatures 10∘Cand90^(@)CThelar≥rsurfaceareas→uchthereservoirs.Thethermalcondutivityofthematerialis0.80Wm^(-1)^(∘)C−1 . Find the amount of heat flowing through the slab per minute.
The correct Answer is:C, D
(T1)=90∘C,(T2)=(10∘C)
l=1cm=0.01m<br>=0.1xx0.1(m^2)=1xx(10^(-2)m^2)<br>k=0.80W//(m-^(@)C)<br>(Q)/(T)=(kA(T_1-T_2))/(l)<br>(8xx10^(-1)xx10^(-2)xx(90-10))/(1xx10^(-2))<br>=64J//s=3840 J//min.
Topper's Solved these Questions
- HEAT TRANSFERBOOK - HC VERMACHAPTER - HEAT TRANSFEREXERCISE - EXERCIESE
1 Videos
- HEAT TRANSFERBOOK - HC VERMACHAPTER - HEAT TRANSFEREXERCISE - Objective 2
6 Videos
- HEAT AND TEMPERATUREBOOK - HC VERMACHAPTER - HEAT AND TEMPERATUREEXERCISE - Exercise
4 Videos
- INTRODUCTION TO PHYSICSBOOK - HC VERMACHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Exercises
19 Videos
Similar Questions
A uniform slab of dimension 10cm×10cm×1cm is kept between two heat reservoirs at temperatures 10°C and 90°C. The larger surface areas touch the reservoirs. The thermal conductivity of the material is 0.80 W/m–°C. Find the amount of heat flowing through the slab per second.
View SolutionA closed cubical box is made of perfectly insulating material and the only way for heat to enter or leave the box is through two solid cylindrical metal plugs, each of cross sectional area 12cm2 and length 8cm fixed in the opposite walls of the box. The outer surface of one plug is kept at a temperature of 100∘C . while the outer surface of the plug is maintained at a temperature of 4(∘)C . The thermal conductivity of the material of the plug is 2.0Wm−1^(@)C^(-1) . A source of energy generating 13W is enclosed inside the box. Find the equilibrium temperature of the inner surface of the box assuming that it is the same at all points on the inner surface.
View Solution
Knowledge Check
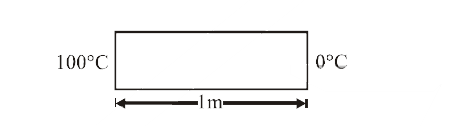
A rod of 1m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperature 100∘C and 0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod =0.09kilocalm−1s−1('∘C)]
 A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/sec
A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/secA slab of stone area 3600cm2 and thickness 10cm is exposed on the lower surface to steam at 100∘C A block of ice at 0∘C rest on upper surface of the slab. In one hour 4.8kg of ice is melted. The thermal conductivity of the stone in Js−1m−1k−1 is (latent heat of ice =3.36×105J/Kg .
A12.0B10.5C1.02D1.24A heat source at T = 500 k is connected to another heat reservoir at t = 100 k by a copper slab which is 1 m long. Given that the thermal conductivity of copper is 0.1Wk−1m−1 , the energy flux through it in the steady state is:
A4000Wm−2B400Wm−2C40Wm−2D4Wm−2
A rod of 1 m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperatures 100∘Cand0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod = 0.09 kilocal m−1s−1(∘C)]
View SolutionA hollow spherical conducting sheel of inner radius R1=0.25m and outer radius R2=0.50m is placed inside a heat reservoir of temperature T0=1000∘C. The shell is initially filled with water at 0∘C. The thermal conductivity of the material is k=1024πW/m−K and its heat capacity is negligible. The time required to raise the temperature of water to 100∘Cis1100ln.109sec. Find K. Take specific heat of water s=4.2kJ/kg.∘C, density of water dw=1000kg/m3π=227

View SolutionA heat source at T = 103 K is connected to another heat reservoir at T = 102 K by a copper slab which is 1 m thick. Given that the thermal conductivity of copper is 0.1 WK−1m−1, the energy flux through it in steady state is :
View SolutionA metal rod of cross sectional area 1.0cm2 is being heated at one end. At one time , the temperature gradient is 5.0∘Ccm−1 at cross section A and is 2.5∘Ccm−1 at cross section B. calculate the rate at which the temperature is increasing in the part AB of the rod. The heat capacity of the part AB=0.40J∘C−1 , thermal conductivity of the material of the rod. K=200Wm−1C−1 . Neglect any loss of heat to the atmosphere.
View SolutionAn icebox made of 1.5cm thick stuyrofoam has dimensions 60cm×60cm×30cm.Itconta∈siceatwhichtheiceisme<∈g.Latentheatoffusionofice=3.36xx10^(5)J kg^(-1).andthermalconductivityofstyrofoam=0.04Wm^(-1)^(∘)C−1 .
View Solution
