A uniform square plate and a disc having same mass per unit area are kept in contact as shown in Fig. The side of square and diameter of circle are both equal to L. Locate the position of centre of mass of the system w.r.t. the centre of the square.
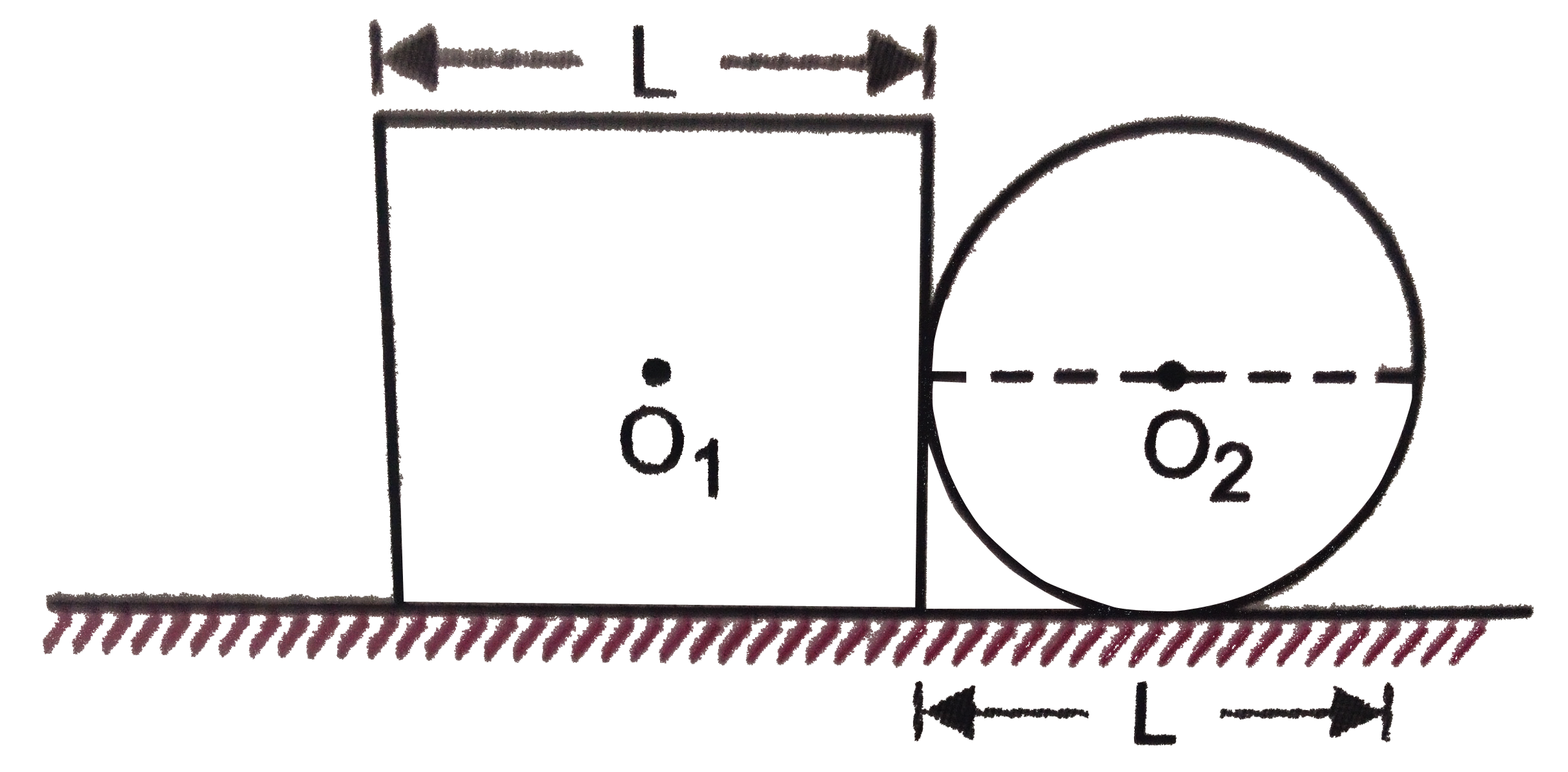
Let mass per unit area of the square plate = mass per unit area of disc =σ
∴ Mass of square plate
m1=σL2, and mass of disc
m2=(πr2)σ=(πL24)σ
Centre of square is O1, where m1 is concentrated and centre of disc is O2, where m2 is concentrated. If x is distance of c.m of the combination from O1, then
xcm=m1x1+m2x2m1+m2=m1×0+m2Lm1+m2
=(πL24)σ×LσL2+πL2σ4=σπL2/4×LσL2(1+π/4)
xcm=π/4×L(4+π)/4=(πL4+π)
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - NCERT
21 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - ADDITIONAL EXERCISES
11 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE E
1 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Knowledge Check
The side of a square is equal to the diameter of a circle. If the side and radius change at the same rate, then the ratio of the change of their areas, is
A1:πBπ:1C2:πD1:2Four particles each of mass m are placed at the vertices of a square of side l. the potential at the centre of square is
A−2√2GmlB−3√2GmlC−2GmlD−4√2GmlA circular plate of diameter d is kept in contact with a square plate of edge d as shown in figure. The densities of the material and the thickness are same
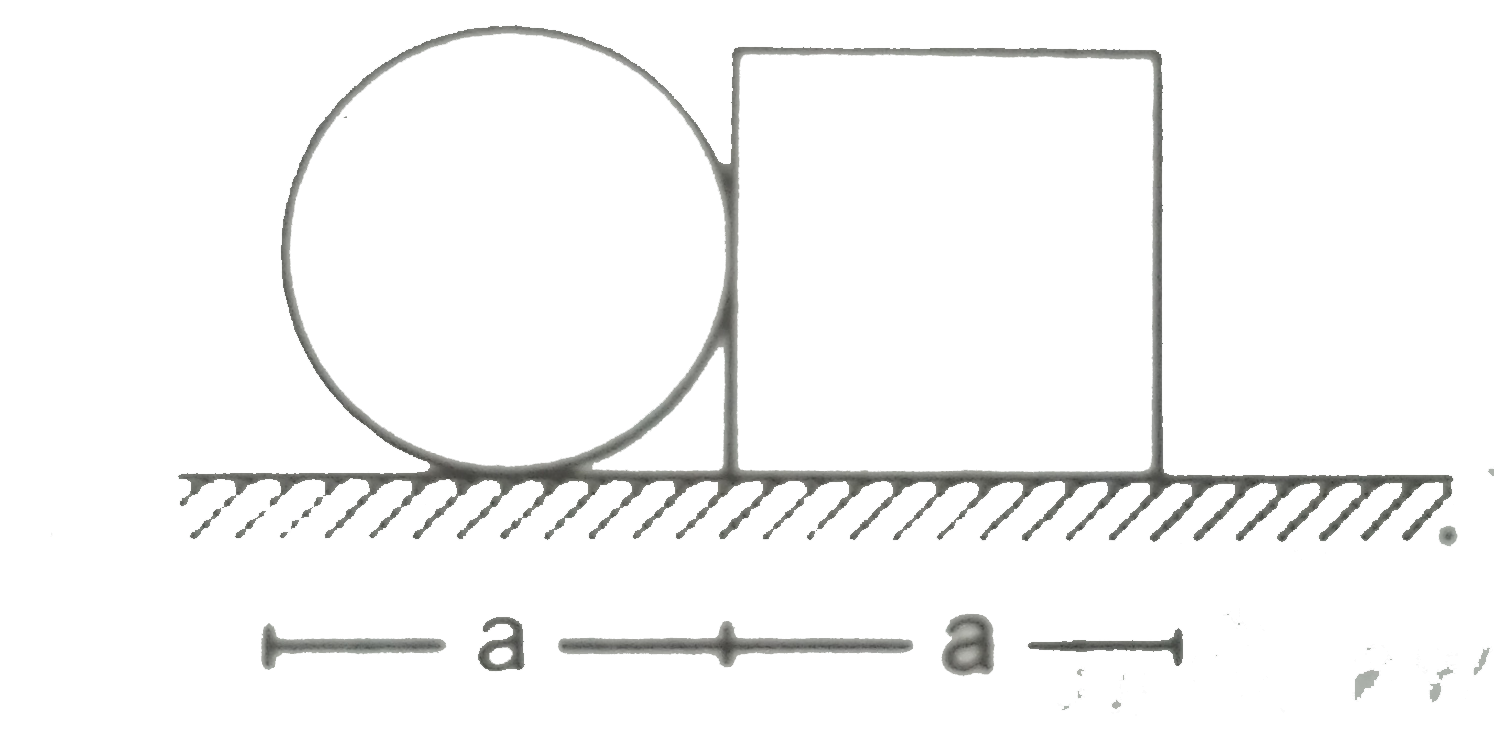 . Everywhere. The centre of mass of the composite system will be Ainside the circular plateBinside hte square plateCat the point of contactDoutside the system
. Everywhere. The centre of mass of the composite system will be Ainside the circular plateBinside hte square plateCat the point of contactDoutside the system
Centre of mass of the system lies inside disc or square plate and why ?
View SolutionA square of side 4m having uniform thickness is divided into four equal squares as shown in Fig. If one of the squares is cut off, find
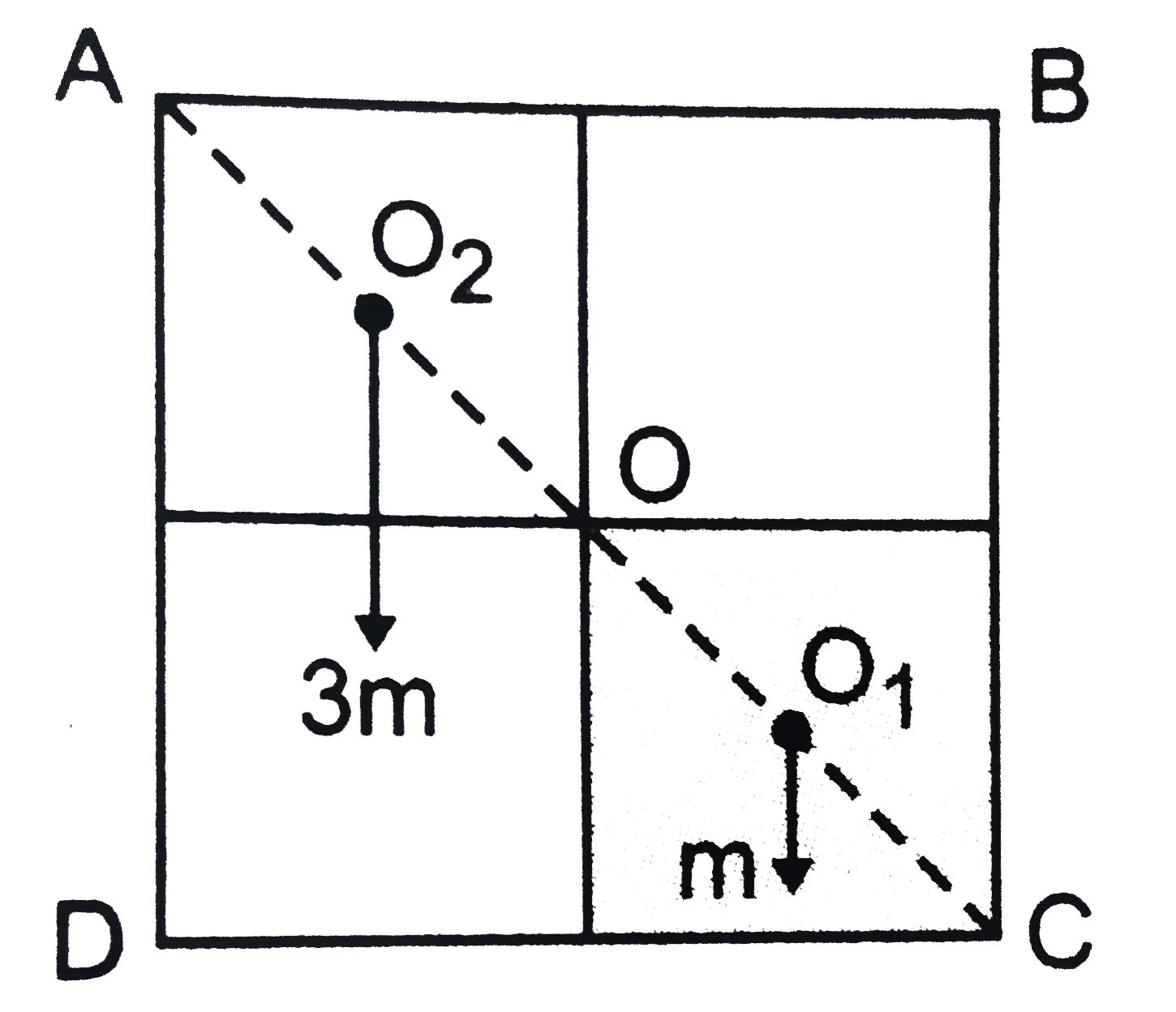
the position of centre of mass of the remaining portion from the centre O.
View SolutionA disc of radius R is placed on a square plate of edge 4R made up of the same sheet with their planes parallel such that any two adjacent sides ofsquare touch the disc. Find the distance of the centre of mass of the system from the centre of square plate?
View SolutionFour masses, each equal to M are placed at the four corners of a square of side a. Calculate the force of attraction on another mass m, kept at the centre of the square.
View SolutionThe object shown in figure is constructed of uniform rods of same material. Find the position of centre of mass w.r.t. its corner O.

View Solution


