A very small solid sphere is set rolling on a rough horizontal track which becomes circular after certain distance. Radius of the circular portion of the track is R which is large compared to radius r of the sphere. Ifaim is that sphere should complete the circle successfully then minimum required speed at the bottom is found to be √27gRn Calculate .
The correct Answer is:7
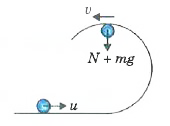
Situation described in the question is as shown in the figure. When sphere reaches the top point of circle then normal reaction and weight P of the body acta vertically downwards assuming v to be the speed at the highest point we can write the following equation:
N+Mg=mv2R
Here note that radius of circular motion corresponding to centre of sphere is taken to be R because it is large in comparison to radius of sphere. To calculate minimum speed at the bottom we can assume critical most situation at the top point when normal reaction becomes zero (N=0)
⇒0+mg+mv2R⇒v=√gR ...(i)
Let us assume that corresponding speed at the bottom is w. We can apply conservation of mechanical energy because sphere performs pure rolling
Loss in K.E.Gain in gravitational potential energy
710mu2−710mv2=mg(2R)
Substituting from equation (1) we get the following
710mu2−710m(√gR)2=mg(2R)
u=√27gR7
On comparing with the given result we get n=7.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Chapter Practice Test (for Board Examination)16videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS (MATRIX MATCH TYPE QUESTIONS) )1videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)6videos
PHYSICAL WORLD
- BOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST15videos
THERMAL PROPERTIES OF MATTER
Similar Questions
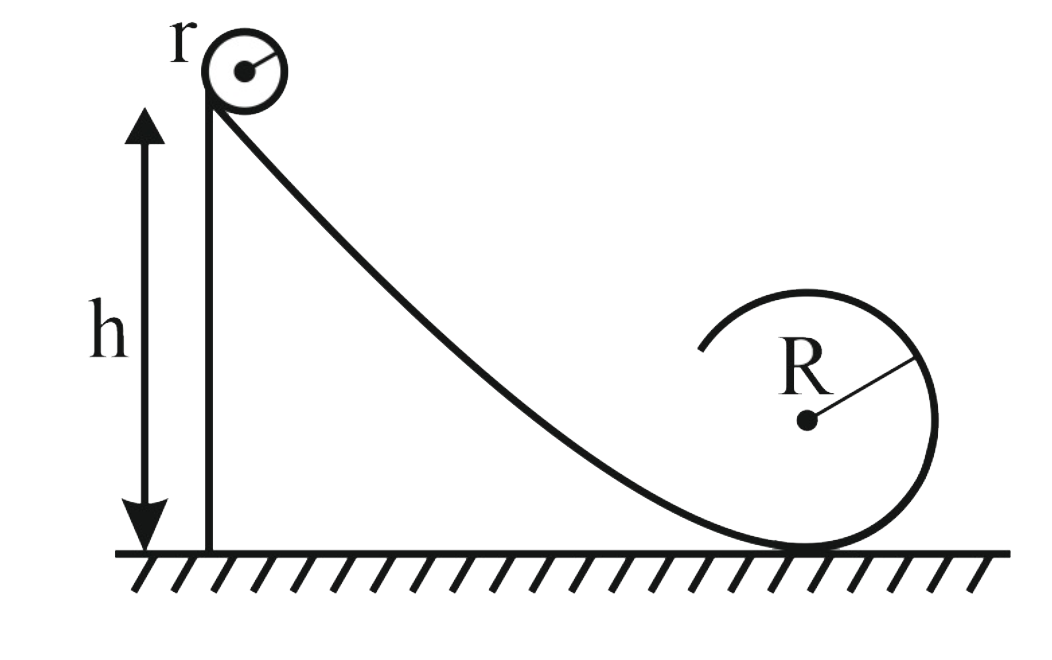
A small solid sphere rolls without slipping along the track shown in figure. The sphere starts from rest from a height h above the bottom of a loop of radius R which is much larger than the radius of the sphere r. The minimum value of h for the sphere to complete the loop is:
 View Solution
View SolutionA solid sphere rolls without slipping along the track shown in the figure. The sphere starts from rest from a height h above the bottom of the loop of radius R which is much larger than the radius of the sphere r. The minimum value of h for the sphere to complete the loop is
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
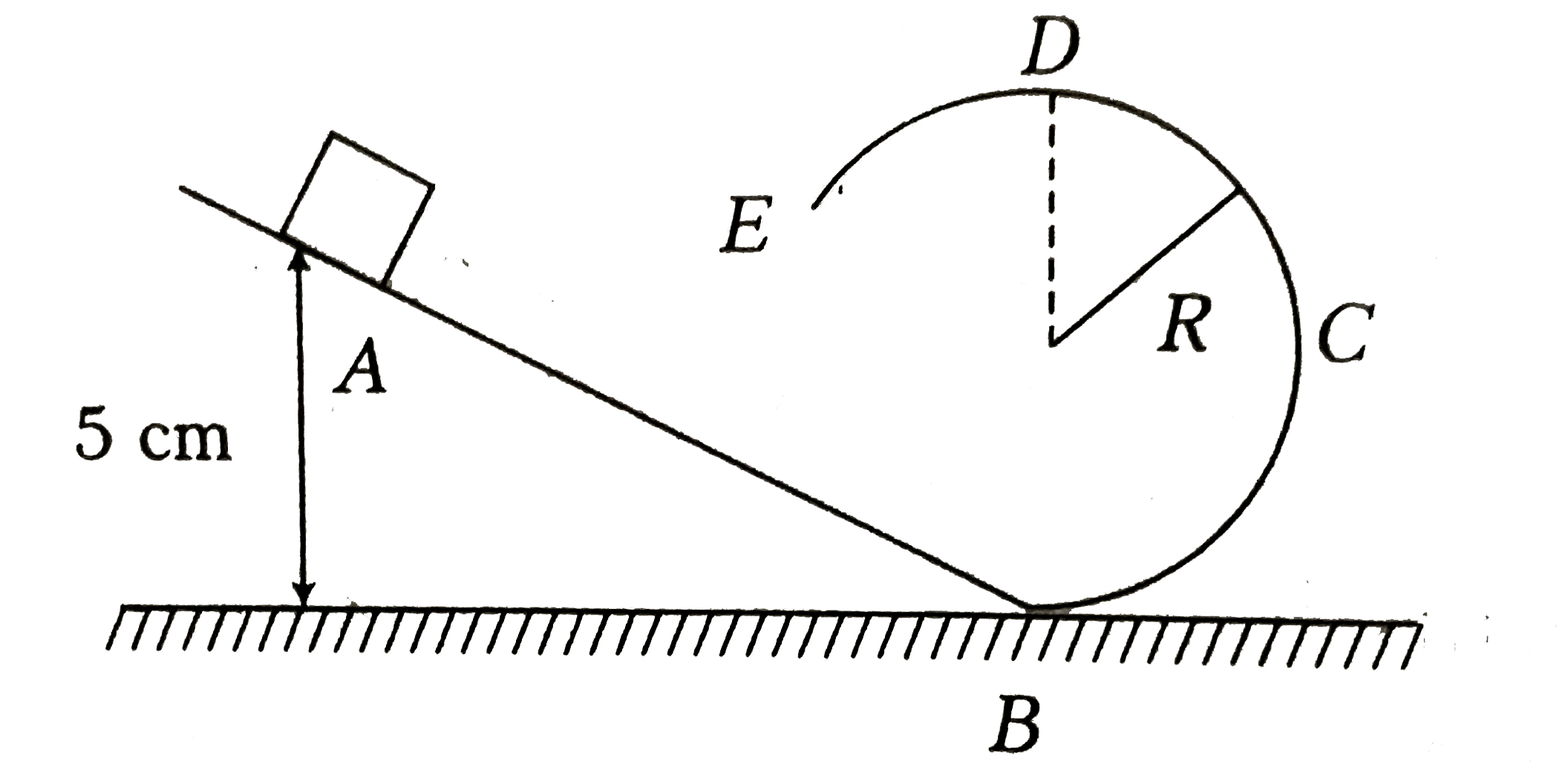
A frictionless track ABCDE ends in a circular loop of radius R. A body slides down the track from point A which is at height h = 5cm. Maximum value of R for a body to complete the loop successfully is
 A2 cmB103cmC154cmD183cm
A2 cmB103cmC154cmD183cm - Question 2 - Select One
A small solid sphere of radius r rolls down an incline without slipping which ends into a vertical loop of radius R. Find the height above the base so that it just loops the loop
A52RB52(R−r)C2510(R−r)D2710R−17r10
Find the radius of the circular section in which the sphere |→r|=5 is cut by the plane →r.ˆi+ˆj+ˆk=3√3.
View SolutionA frictionless track ABCDE ends in a circular loop of radius R. A body slides down the track from point A which is at height h = 5cm. Maximum value of R for a body to complete the loop successfully is
 View Solution
View SolutionA solid sphere is rolling on a horizontal surface such that speed of the centre of sphere is u. Mase of the sphere is m and radius R. Magnitude of angular momentum about the point of contact is found to be 7nmvR/. Find the value of n
View SolutionFigure shows a rough track a portion of which is in the form of a cylinder of radius R. With what minimum linear speed should as sphere of radius r be set rolling on the horizontal part so that it completely goes round the circle on the cylindrical part.
 View Solution
View SolutionFigure shows a rough track a portion of which is in the form of a cylinder of radius R. With what minimum linear speed should as sphere of radius r be set rolling on the horizontal part so that it completely goes round the circle on the cylindrical part.
 View Solution
View SolutionA small solid marble of mass M and radius r rolls down along the loop track, without slipping. Find the height h above the base, from where it has to start rolling down the incline such that the sphere just completes the vertical circular loop of radius R.
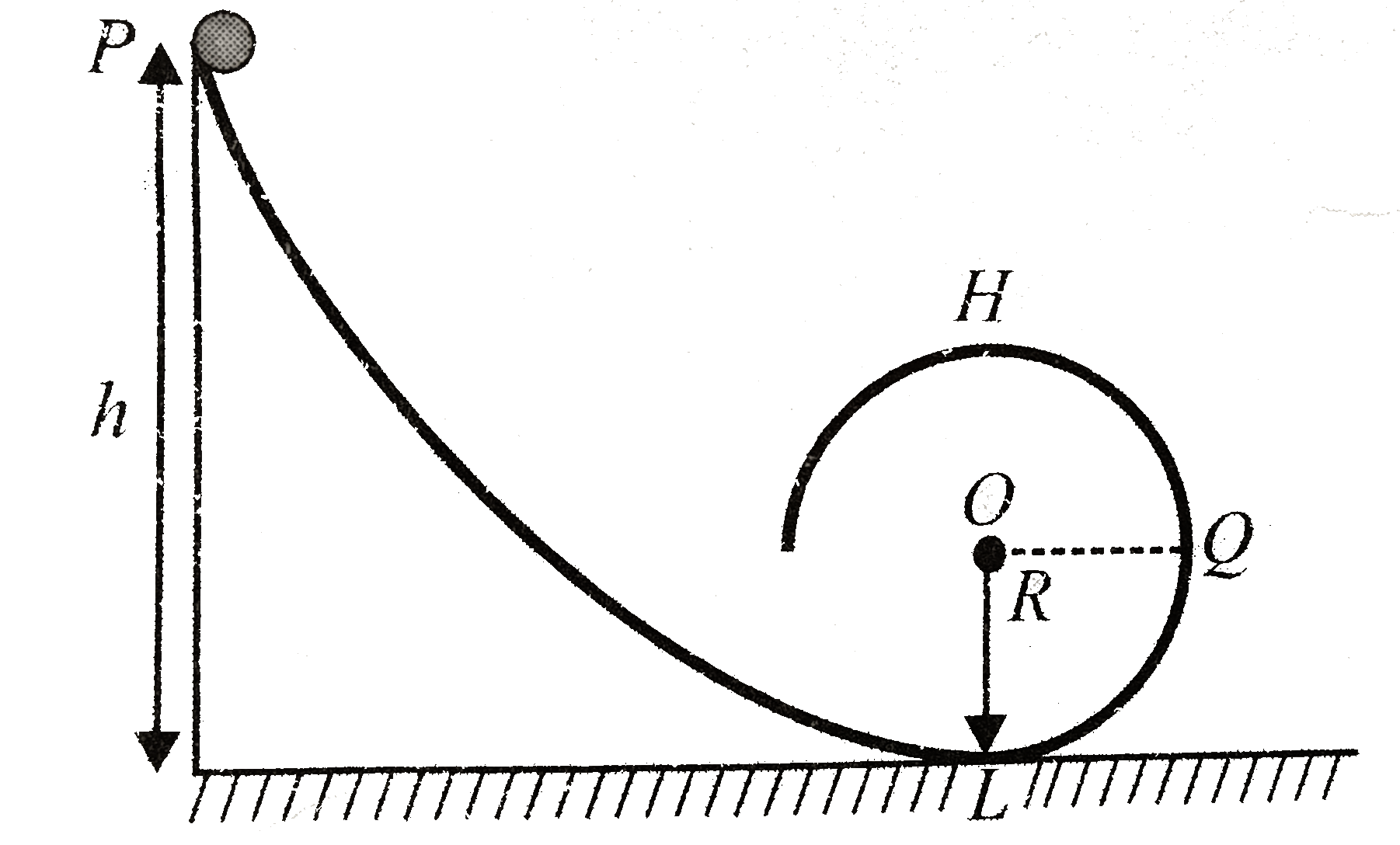 View Solution
View Solution

