Question

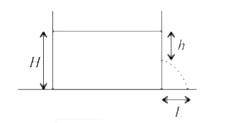
A water barrel stands on a table of height $h$. If a small holes is punched in the side of the barrel at its base, it is found that the resultant stream of water strikes the ground at a horizontal distance $R$ from the table. What is the depth of water in the barrel?

Options

A. `(R^(2))/(h)`
B. `(R^(2))/(2h)`
C. `(R^(2))/(4h)`
D. `(4R^(2))/(h)`

Answer

C

Explanation

To find the depth of water in the barrel when a small hole is punched in the side at the base, we can follow these steps:

1. Identify Variables:
- Let \( H \) be the depth of water in the barrel.
- Let \( h \) be the height of the table.
- Let \( R \) be the horizontal distance from the table where the water strikes the ground.

2. Determine the Velocity of Water:
- According to Torricelli's theorem, the velocity \( V \) of water flowing out of the hole at the base of the barrel is given by:
\( V = \sqrt{2gH} \)
where \( g \) is the acceleration due to gravity.

3. Calculate the Time of Flight:
- The water falls a vertical distance of \( h \) (the height of the table). The time \( t \) taken to fall this distance can be calculated using the equation of motion:
\( h = \frac{1}{2} g t^2 \)
Rearranging gives:
\( t = \sqrt{\frac{2h}{g}} \)

4. Calculate the Horizontal Range:
- The horizontal range \( R \) can be expressed as:
\( R = V \cdot t \)
Substituting the expressions for \( V \) and \( t \):
\( R = \sqrt{2gH} \cdot \sqrt{\frac{2h}{g}} = \sqrt{4hH} \)

5. Square Both Sides:
- Squaring both sides of the equation gives:
\( R^2 = 4hH \)

6. Solve for Depth \( H \):
- Rearranging the equation to find \( H \):
\( H = \frac{R^2}{4h} \)

Final Answer:
The depth of water in the barrel is:
\( H = \frac{R^2}{4h} \)