A tank of height H is fully filled with water. If the water rushing from a hole made in the tank below the free surface , strikes the floor at a maximum horizontal distance , then the depth of the hole from the free surface must be
34H
23H
14H
12H
The correct Answer is:D
To solve the problem, we need to determine the depth of the hole from the free surface of the water in the tank, such that the water strikes the floor at the maximum horizontal distance. Here’s a step-by-step solution:
Step 1: Understanding the Problem
We have a tank of height H filled with water. A hole is made at a depth h from the free surface. The water will exit the hole and fall to the ground, and we want to find the value of h that maximizes the horizontal distance R that the water travels before hitting the ground.
Step 2: Determine the Efflux Velocity
The velocity of water exiting the hole can be determined using Torricelli's Law, which states:
v=√2gh
where h is the depth of the hole from the free surface.
Step 3: Calculate the Time of Flight
The water will fall a vertical distance of H−h before hitting the ground. We can use the second equation of motion to find the time t it takes to fall this distance:
s=ut+12gt2
Here, s=H−h, u=0 (initial velocity), and g is the acceleration due to gravity. Thus, the equation simplifies to:
H−h=12gt2
Rearranging gives:
t2=2(H−h)g
Taking the square root:
t=√2(H−h)g
Step 4: Calculate the Horizontal Distance
The horizontal distance R traveled by the water can be expressed as:
R=v⋅t
Substituting the values of v and t:
R=√2gh⋅√2(H−h)g
Simplifying this expression:
R=√2gh⋅√2(H−h)g=√4h(H−h)g
Thus, we have:
R=2√h(H−h)√g
Step 5: Maximize the Horizontal Distance
To find the maximum distance, we need to take the derivative of R with respect to h and set it to zero:
dRdh=2√g⋅ddh(√h(H−h))
Using the product rule:
ddh(√h(H−h))=12√h(H−h)⋅((H−h)−h)=H−2h2√h(H−h)
Setting this equal to zero for maximization:
H−2h=0⟹h=H2
Conclusion
The depth of the hole from the free surface that maximizes the horizontal distance is:
h=H2
We have a tank of height H filled with water. A hole is made at a depth h from the free surface. The water will exit the hole and fall to the ground, and we want to find the value of h that maximizes the horizontal distance R that the water travels before hitting the ground.
The velocity of water exiting the hole can be determined using Torricelli's Law, which states:
v=√2gh
where h is the depth of the hole from the free surface.
The water will fall a vertical distance of H−h before hitting the ground. We can use the second equation of motion to find the time t it takes to fall this distance:
s=ut+12gt2
Here, s=H−h, u=0 (initial velocity), and g is the acceleration due to gravity. Thus, the equation simplifies to:
H−h=12gt2
Rearranging gives:
t2=2(H−h)g
Taking the square root:
t=√2(H−h)g
The horizontal distance R traveled by the water can be expressed as:
R=v⋅t
Substituting the values of v and t:
R=√2gh⋅√2(H−h)g
Simplifying this expression:
R=√2gh⋅√2(H−h)g=√4h(H−h)g
Thus, we have:
R=2√h(H−h)√g
To find the maximum distance, we need to take the derivative of R with respect to h and set it to zero:
dRdh=2√g⋅ddh(√h(H−h))
Using the product rule:
ddh(√h(H−h))=12√h(H−h)⋅((H−h)−h)=H−2h2√h(H−h)
Setting this equal to zero for maximization:
H−2h=0⟹h=H2
The depth of the hole from the free surface that maximizes the horizontal distance is:
h=H2
Similar Questions
A tank if filled with water upto height H. When a hole is made at a distance h below the level of water. What will be the horizontal range of water jet ?
View SolutionA liquid is coming out from the orifice of tank and falls upto a maximum horizontal distance of 6 m. The height h is equal to
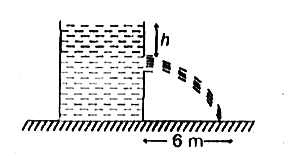
View Solution
Knowledge Check
A water barrel stands on a table of height h. If a small holes is punched in the side of the barrel at its base, it is found that the resultant stream of water strikes the ground at a horizontal distance R from the table. What is the depth of water in the barrel?
AR2hBR22hCR24hD4R2h
A tank is filled to a height H. The range of water coming out of a hole which is a depth H/4 from the surface of water level is
View SolutionA liquid is filled in a vessel and a hole is made at a depth h below the free surface of the liquid.
Statement-1 : Greater is the distance of the hole form the free surface of liquid, greater will be the velocity of efflux.
And
Statement-2 The speed of the liquid coming out of the orifice depends on the quantity of liquid in the vessel.
View SolutionA tank is filled with water upto a height H. A hole is punched in one of the walls at a depth h1 below the water surface. (a) Find the distance from the foot of the wall at which the stream strikes the floor. (6) Is it possible to make second hole at another depth so that this second stream also has the same range? If so find its depth ?
View SolutionWater level is maintained ina cylindrical vessel up to a fixed height H. The vessel is kept on a horizontal plane. At what height above the bottom should a hole be made in the vessel so that the water stream coming out of the hole strikes the horizontal plane at the greatest distance from the vessel.

View SolutionA rectangular tank is placed on a horizontal ground and is filled with water to a height H above the base. A small hole is made on one vertical side at a depth D below the level of the water in the tank. The distance x from the bottom of the tank at which the water jet from the tank will hit the ground is
View SolutionA tank is filled by water (ρ=103kg/m3). A small hole is made in the side wall of the tank at depth 10 m below water surface. A water jet emerges horizontal from the hole and falls at horizontal distance R from it. The amount of extra pressure (in terms of atmospheric pressure) that must be applied on the water surface, so that range becomes 3R on the on the ground will be (cross section area of hole is negligible and 1 atm = 105 Pa, g = 10 m/s2)
View SolutionA squre hole of side length l is made at a depth of h and a circular hole is made at a depht of 4 h from the surface of water in a water tank kept on a horizontal surface (where l ltlt h). Find the radius r of the circular hole if equal amount of water comes out of the vessel through the holes per second.
View Solution
