A wave travels along the positive x-direction with a speed of 20ms−1. The amplitude of the wave is 0.20 cm and the wavelength 2.0 cm. (a) Write a suitable wave equation which describes this wave. (b) What is the displacement and velocity of the particle at x = 2.0 cm at time t = 0 according to the wave equation written ?
The correct Answer is:A, B, C, D
To solve the problem step by step, we will address both parts of the question.
Part (a): Writing the Wave Equation
1. Identify Given Values:
- Speed of the wave, V=20m/s
- Amplitude, A=0.20cm=0.20×10−2m
- Wavelength, λ=2.0cm=2.0×10−2m
2. Calculate the Wave Number k:
k=2πλ=2π2.0×10−2=2π0.02=100πm−1
3. Calculate the Angular Frequency ω:
- First, find the frequency ν:
ν=Vλ=20m/s2.0×10−2m=1000Hz
- Now calculate ω:
ω=2πν=2π×1000s−1=2000πs−1
4. Write the Wave Equation:
The general form of the wave equation traveling in the positive x-direction is:
y(x,t)=Asin(kx−ωt)
Substituting the values of A, k, and ω:
y(x,t)=(0.20×10−2)sin(100πx−2000πt)
Part (b): Finding Displacement and Velocity at x=2.0cm and t=0
1. Substituting Values into the Wave Equation:
- Convert x=2.0cm to meters:
x=2.0cm=2.0×10−2m
- Substitute x and t=0 into the wave equation:
y(2.0×10−2,0)=(0.20×10−2)sin(100π×2.0×10−2−2000π×0)
=(0.20×10−2)sin(2π)=(0.20×10−2)×0=0
2. Finding the Velocity of the Particle:
- The velocity v is given by:
v=dydt=−Aωcos(kx−ωt)
- Substitute A, ω, k, x, and t=0:
v=−(0.20×10−2)(2000π)cos(100π×2.0×10−2−2000π×0)
=−(0.20×10−2)(2000π)cos(2π)
=−(0.20×10−2)(2000π)×1=−0.20×2000π×10−2
=−4πm/s
Final Answers:
- (a) The wave equation is:
y(x,t)=(0.20×10−2)sin(100πx−2000πt)
- (b) The displacement at x=2.0cm and t=0 is 0m and the velocity is −4πm/s.
- Speed of the wave, V=20m/s
- Amplitude, A=0.20cm=0.20×10−2m
- Wavelength, λ=2.0cm=2.0×10−2m
k=2πλ=2π2.0×10−2=2π0.02=100πm−1
- First, find the frequency ν:
ν=Vλ=20m/s2.0×10−2m=1000Hz
- Now calculate ω:
ω=2πν=2π×1000s−1=2000πs−1
The general form of the wave equation traveling in the positive x-direction is:
y(x,t)=Asin(kx−ωt)
Substituting the values of A, k, and ω:
y(x,t)=(0.20×10−2)sin(100πx−2000πt)
- Convert x=2.0cm to meters:
x=2.0cm=2.0×10−2m
- Substitute x and t=0 into the wave equation:
y(2.0×10−2,0)=(0.20×10−2)sin(100π×2.0×10−2−2000π×0)
=(0.20×10−2)sin(2π)=(0.20×10−2)×0=0
- The velocity v is given by:
v=dydt=−Aωcos(kx−ωt)
- Substitute A, ω, k, x, and t=0:
v=−(0.20×10−2)(2000π)cos(100π×2.0×10−2−2000π×0)
=−(0.20×10−2)(2000π)cos(2π)
=−(0.20×10−2)(2000π)×1=−0.20×2000π×10−2
=−4πm/s
- (a) The wave equation is:
y(x,t)=(0.20×10−2)sin(100πx−2000πt)
- (b) The displacement at x=2.0cm and t=0 is 0m and the velocity is −4πm/s.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer7videos
WAVE MOTION AND WAVES ON A STRING
- BOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Objective -29videos
WAVE MOTION AND WAVES ON A STRING
- BOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Questions for short Answer9videos
THE FORCES
- BOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer16videos
WORK AND ENERGY
Similar Questions
Transverse waves on a srting have speed 12.0m/s, amplitude 0.05m and wavelength 0.4m. The waves travel in the +x−direction and at t=0 the x=0 end of the string has zero displacement and is moving upwards.
(a) Write a wave function describing the wave.
(b) Find the transverse displacement of a point at x=0.25m at time t=0.15s.
( c ) How much time must elapse from the instant in part (b) until the point at x=0.25m has zero dispacement?View SolutionA wave propagates in a string in the positive x-direction with velocity v. The shape of the string at t=t0 is given by
f(x,t0)=Asin(x2a2). Then the wave equation at any instant t is given byView SolutionA wave travelling on a string at a speed of 10ms−1 causes each particle of the string to oscillate with a time period of 20 ms. (a) What is the wavelength of the wave ? (b) If the displacement of a particle is 1.5 mm at a certain instant, what will be the displacement of a particle 10 cm away from it at the same instant ?
View SolutionA wave in a string has an amplitude of 2cm. The wave travels in the +ve direction of x axis with a speed of 128ms−1 and it is noted that 5 complete waves fit in 4m length of the string. The equation describing the wave is
View SolutionA certain transverse sinusoidal wave of wavelength 20 cm is moving in the positive x direction. The transverse velocity of the particle at x=0 as a function of time is shown. The amplitude of the motion is:
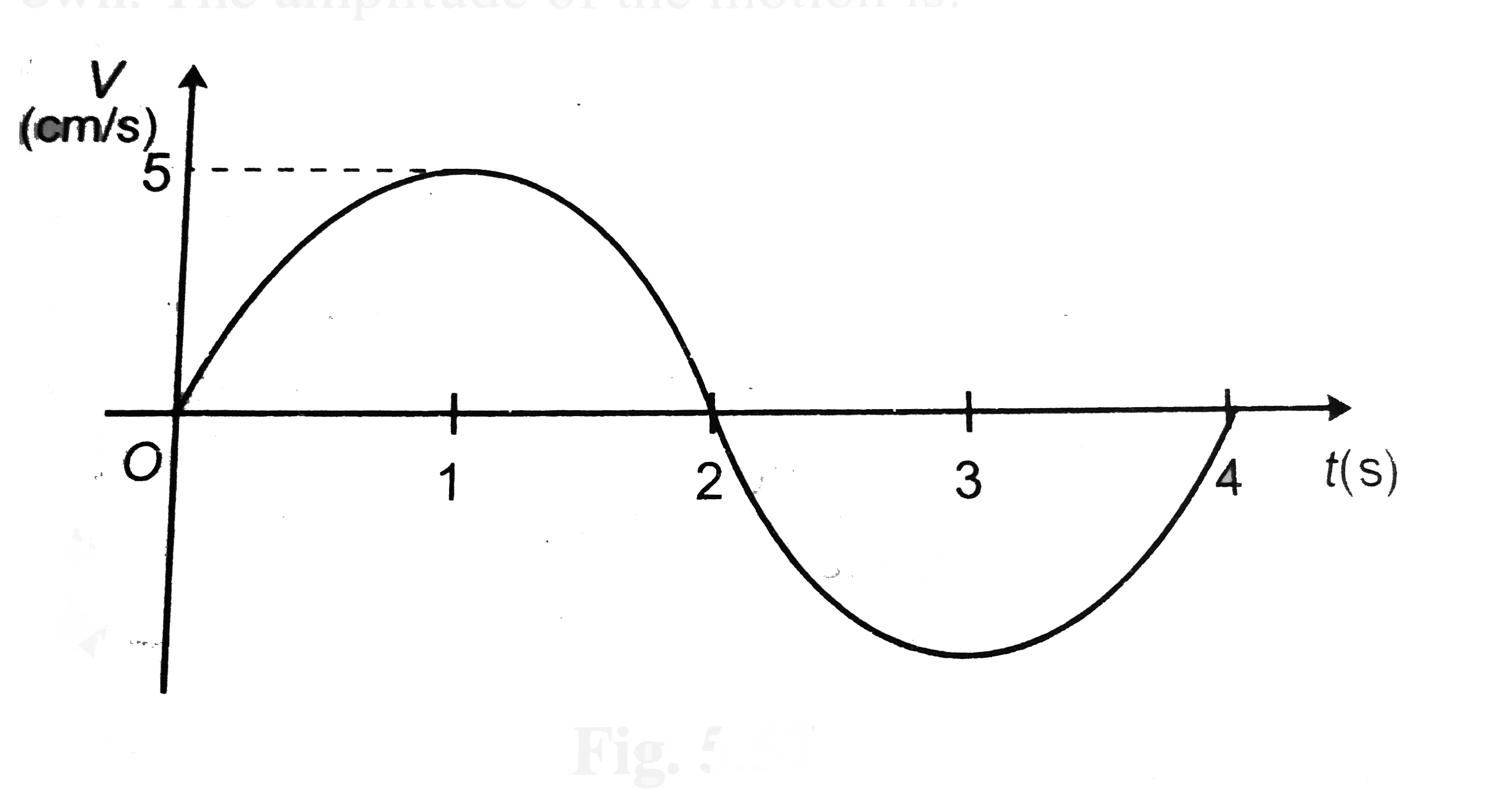 View Solution
View SolutionA certain transverse sinusoidal wave of wavelength 20 cm is moving in the positive x direction. The transverse velocity of the particle at x=0 as a function of time is shown. The amplitude of the motion is:
 View Solution
View SolutionA sinusoidal wave trsvelling in the positive direction on a stretched string has amplitude 2.0cm, wavelength 1.0m and velocity 5.0m/s. At x=0 and t=0 it is given that y=0 and ∂y∂t<0. Find the wave function y(x,t).
View SolutionA transverse sinusoidal wave of wavelength 20 cm is moving along a string towards increasing x. the transverse displacement of the string particle at x=0 as a function of time is shown in figure.
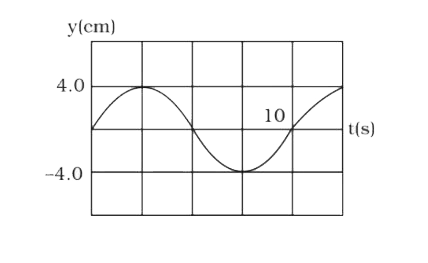
Q. The velocity of propagation of the wave is ?View SolutionA wave of wavelength 0.60 cm is produced in air and it travels at a speed of 300 ms^-1. Will it be audible?
View SolutionA sinusoidal wave travelling in the positive x-direction has an amplitude of 15.0cm, a wavelength of 40.0cm and a frequency of 8.00Hz.Thevertic≤displacementofthemediumatt = 0 and x = 0isalso15.0 cmasshown∈figure.<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/DCP_V03_C17_E01_054_Q01.pngwh=80%mx-autosty≤=background:white;><br>(a)F∈dtheanga––rwaveνmberk,periodT,anga––requencyomegaandspeedv of the wave.
(b) Write a general expression for wave function.View Solution

