A wooden block of mass 0.5 kg and density 800 kgm−3 is fastened to the free end of a vetical spring of spring cosntant 50 Nm−1 fixed at the bottom. If the entire system is completely immersed in water, find a. the elongation (or compresion) of the spring in equilibrium and b. the time period of vertical oscillations of het block when it is slightly depressed and released.
The correct Answer is:A, B, C
Given m = 5kg, P=800kgm3,k=50Nm
a. From the free body diagram
[hereVρg>mg. So, elongation will be there]
mg=kX+Vρug
→(0.5)x(10+50x(X))
=(0.5500)×103×(10)
→50x=(0.5)×(10)×((108)−1)
→50x=(54)
→x=(5200)
=x=2.5cm
b. since the system is inside water only kx unbalance force will be the driving force for SHM. There is no change in buoyant force
So, ma=kx
→a=kxm
→w2x=kxm
→(2pT)2=km
→T=2π√mk
=2π×√(0.550)=π5sec
Topper's Solved these Questions
Similar Questions
A block of mass m and relative density y(<1) si attached to an ideal spring of constant K. The system is initially at rest and at equilibrium. If the container acceleration upwards with a0, find the increase in the elongation of the spring in equilibrium.
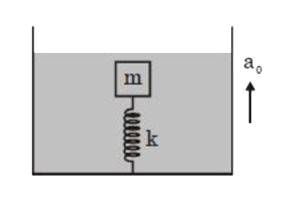
View SolutionSpring mass system is shown in figure. Find the elastic potential energy stored in each spring when block is at its mean position. Also find the time period of vertical oscillations. The system is in vertical plane.

View Solution
Knowledge Check
Two blocks of masses 6 kg and 3 kg are attached to the two ends of a massless spring of spring constant 2π2N/m. If spring is compressed and released on a smooth horizontal surface then find the time period (in seconds) of each block.
A1B2C3D4Figure shows a system consisting of a massless pulley, a spring of force constant k and a block of mass m. If the block is slightly displaced vertically down from is equilibrium position and then released, the period of its vertical oscillation is
 A2π√mKBπ√m4KCπ√mKD4π√mK
A2π√mKBπ√m4KCπ√mKD4π√mKIn the figure, a block of mass m is rigidly attached to two identical springs of stiffness k each. The other ends of the springs are connected to the fixed wall. When the block is in equilibrium, length of each spring is b, which is greater than the natural length of the spring. The time period of the oscillation of the block if it is displaced by small distance perpendicular to the length of the springs and released. Space is gravity free.
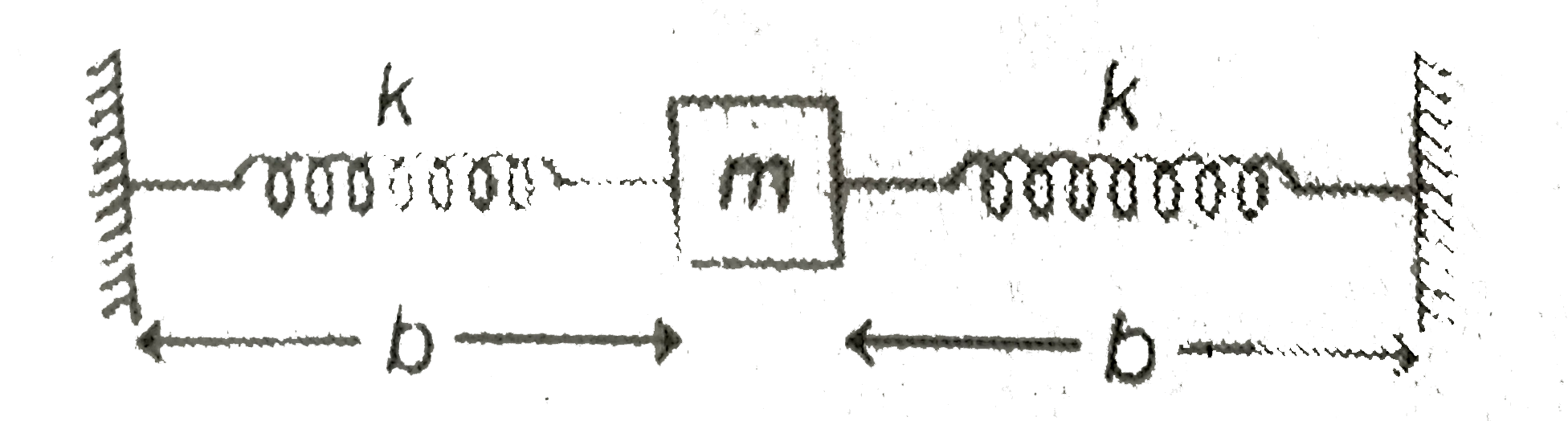 A2π√mbk(b−1)B2π√mb2k(b−l)C2π√(mb−lkb)D2π√(mb−l2kb)
A2π√mbk(b−1)B2π√mb2k(b−l)C2π√(mb−lkb)D2π√(mb−l2kb)
Spring mass system is shown in figure. Find the elastic potential energy stored in each spring when block is at its mean position. Also find the time period of vertical oscillations. The system is in vertical plane.

View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View SolutionTwo blocks of masses m1 and m2 are attached to the lower end of a light vertical spring of force constant k. The upper end of the spring is fixed. When the system is in equilibrium, the lower block (m2) drops off. The other block (m1) will

View SolutionA cubical block of wood of edge a and density ρ floats in water of density 2ρ. The lower surface of the cube just touches the free end of a mass less spring of force constant K fixed at the bottom of the vessel. The weight W put over the block so that it is completely immersed in water without wetting the weight is
View SolutionA body of mass m is attached to the lower end of a spring whose upper end is fixed. The spring has negligible mass. When the mass m is slightly pulled down and released, it oscillates with a time period of 3s. When the mass m is increased by 1kg, the time period of oscillations becomes 5s. The value of m in kg is
View Solution
