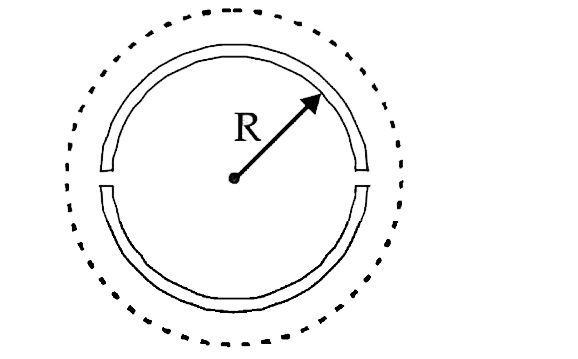
A wooden wheel of radius R is made of two semicircular part . The two parts are held together by a ring made of a metal strip of cross sectional area S and length L. L is slightly less than $2 π R$ . To fit the ring on the wheel, it is heated so that its temperature rises by $Δ T$ and it just steps over the wheel. As it cools down to surrounding temperature, it process the semicircle parts together. If the coefficient of linear expansion of the metal is $α$ , and it Young's modulus is Y, the force that one part of the wheel applies on the other part is :

A

$2 S Y α Δ T$

B

$2 S Y α Δ T$

C

$2 π S Y Δ T$

D

$S Y α Δ T$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

If temperature rises by $Δ T$ , then increase in length, $Δ L = L α Δ T$
Let tension developed in the ring be $T_{1}$ .
Stress developed in string
$Y = \frac{\frac{T}{S}}{\frac{Δ L}{L}} = \frac{T}{S α Δ T} \Rightarrow T = S Y α Δ T$
For one part of the wheel, (as shown in figure)
We can say, F= 2T
Here F is the force that one part of the wheel applies on the other part.
$∴ F = 2 S Y α Δ T$

|

Updated on:21/07/2023

Class 11 PHYSICS PROPERTIES OF SOLIDS AND FLUIDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The diameter of a fly wheel is $R$ . Its coefficient of linear expansion is $α$ . If its temperature is increased by $δ T$ the percentage increase in its moment of inertia is
A $200 \times α \times Δ T$
B $100 \times α \times Δ T$
C $50 \times α \times Δ T$
D $150 \times α \times Δ T$
Question 2 - Select One
A metal ring of initial radius r and cross-sectional area A is fitted onto a wooden disc of radius R > r. If Youngs modules of metal is Y then tension in the ring is
A $\frac{A Y R}{r}$
B $\frac{A Y (R - r)}{r}$
C $\frac{Y}{A} (\frac{R - r}{r})$
D $\frac{Y r}{A R}$
Question 3 - Select One
The length of an iron wire is L and area of cross-section is A. The increase in length is $l$ on applying the force F on its two ends. Which of the statement is correct
AIncrease in length is inversely proportional to its length L
BIncrease in length is proportional to area of cross-section A
CIncrease in length is inversely proportional to A
DIncrease in length is proportional to Yound's modulus.

MODERN PUBLICATION-THERMAL PROPERTIES OF MATTER-COMPETITION FILE (OBJECTIVE TYPE QUESTION ((B) MULTIPLE CHOICE QUESTION FROM CPMPETITIVE EXAMINATION ) JEE MAIN AND OTHER STATE BOARDS FOR ENGINEERING ENTRANCE)

Home
Ask Doubt
Profile