An airplane flies northward from town A and B and then back again. There is a steady wind blowing towards the north so that for the first state of the trip, the airplane is flying in the same direction as the wind and for the return trip of the journey, the airplane is flying opposite of the wind. The total trip time Tω as compared to the total trip time in the absence of any winds T0 is:
Tw=T0
Tw>T0
Tw<T0
Data is insufficient
The correct Answer is:A
To solve the problem, we need to analyze the airplane's journey in the presence of wind and compare it to the journey in the absence of wind. Let's break it down step by step.
Step 1: Define Variables
Let:
- VA = speed of the airplane in still air
- VW = speed of the wind
- d = distance between towns A and B
- T0 = total trip time in the absence of wind
- Tω = total trip time in the presence of wind
Step 2: Calculate Time from A to B
When the airplane flies from A to B, it is flying in the same direction as the wind. Therefore, the effective speed of the airplane is:
VAB=VA+VW
The time taken to travel from A to B, denoted as t1, is given by:
t1=dVAB=dVA+VW
Step 3: Calculate Time from B to A
When the airplane returns from B to A, it is flying against the wind. Therefore, the effective speed of the airplane is:
VBA=VA−VW
The time taken to travel from B to A, denoted as t2, is given by:
t2=dVBA=dVA−VW
Step 4: Calculate Total Time in Presence of Wind
The total time Tω for the round trip in the presence of wind is:
Tω=t1+t2=dVA+VW+dVA−VW
Factoring out d:
Tω=d(1VA+VW+1VA−VW)
Step 5: Calculate Total Time in Absence of Wind
In the absence of wind, the time T0 for the round trip is simply:
T0=dVA+dVA=2dVA
Step 6: Compare Tω and T0
To compare Tω with T0, we can express Tω in terms of T0:
Tω=d(1VA+VW+1VA−VW)
This can be simplified further:
Tω=d((VA−VW)+(VA+VW)(VA+VW)(VA−VW))=d(2VAV2A−V2W)
Thus,
Tω=2dVA⋅VAV2A−V2W=T0⋅11−(VWVA)2
Conclusion
Since VW is positive, Tω>T0. Therefore, the total trip time Tω in the presence of wind is greater than the total trip time T0 in the absence of wind.
Final Answer
The total trip time Tω is greater than T0.
---
Let:
- VA = speed of the airplane in still air
- VW = speed of the wind
- d = distance between towns A and B
- T0 = total trip time in the absence of wind
- Tω = total trip time in the presence of wind
When the airplane flies from A to B, it is flying in the same direction as the wind. Therefore, the effective speed of the airplane is:
VAB=VA+VW
The time taken to travel from A to B, denoted as t1, is given by:
t1=dVAB=dVA+VW
When the airplane returns from B to A, it is flying against the wind. Therefore, the effective speed of the airplane is:
VBA=VA−VW
The time taken to travel from B to A, denoted as t2, is given by:
t2=dVBA=dVA−VW
The total time Tω for the round trip in the presence of wind is:
Tω=t1+t2=dVA+VW+dVA−VW
Factoring out d:
Tω=d(1VA+VW+1VA−VW)
In the absence of wind, the time T0 for the round trip is simply:
T0=dVA+dVA=2dVA
To compare Tω with T0, we can express Tω in terms of T0:
Tω=d(1VA+VW+1VA−VW)
This can be simplified further:
Tω=d((VA−VW)+(VA+VW)(VA+VW)(VA−VW))=d(2VAV2A−V2W)
Thus,
Tω=2dVA⋅VAV2A−V2W=T0⋅11−(VWVA)2
Since VW is positive, Tω>T0. Therefore, the total trip time Tω in the presence of wind is greater than the total trip time T0 in the absence of wind.
The total trip time Tω is greater than T0.
Topper's Solved these Questions
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - MCQ_TYPE
27 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - COMPREHENSION_TYPE
19 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
Similar Questions
High speed wind blows over a house. The force on the roof is
View SolutionAn aeroplane flies along a straight path AandB and returns back again. The distance between AandB is l and the aeroplane maintains the constant speed v w.r.t. wind.
There is a steady wind with a speed u at an angle θ with line AB. Determine the expression for the total time of the trip.
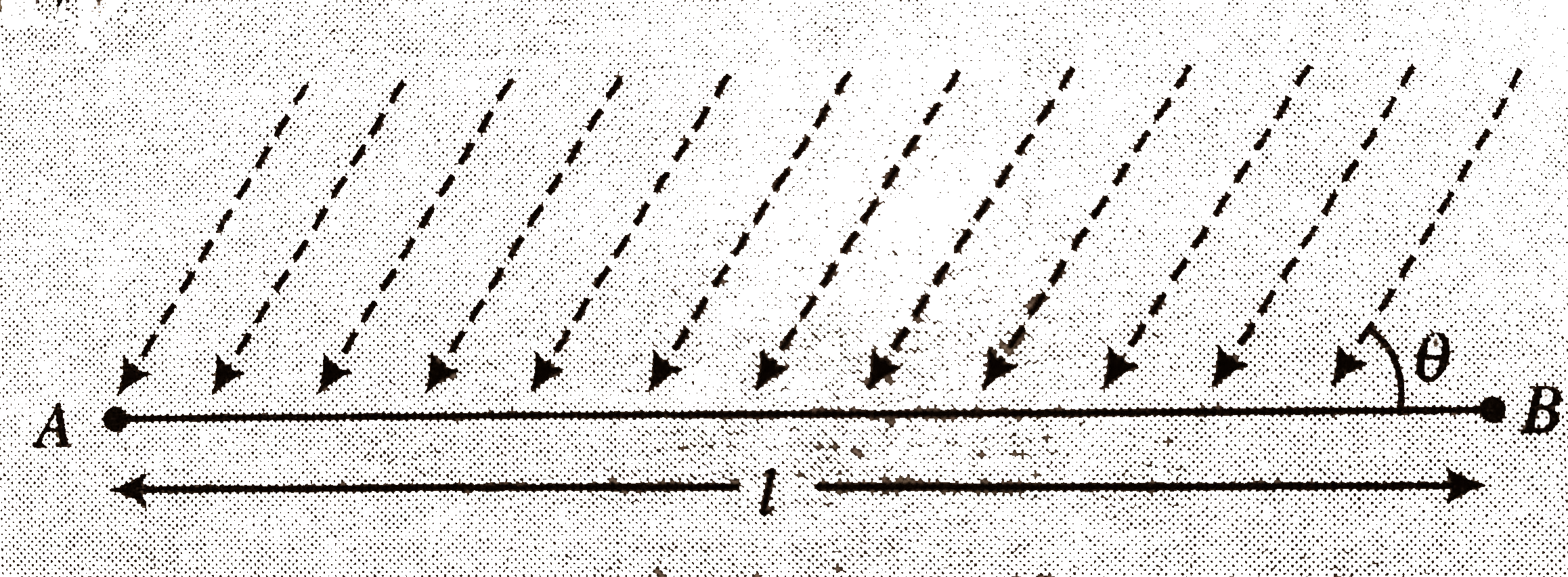 .
.
View Solution
Knowledge Check
A car traveled at 80 mph for the first half of the time of a trip and at 40 mph for the second half of the trip. What is the average speed of the car during the entire trip?
A20B40C50D60A car traveled 65% of the way from Towm A to Town B at an average speed of 65 mph. The car traveled at an average speed of v mph for the remaining part of the trip. The average speed for the entire trip was 50 mph. What is v is mph?
A65B50C45D35
The wheels of an airplane are set into rotation just before landing so that the wheels do not slip on the ground. If the airplane is travelling in the east direction, what should be the direction of angular velocity vector of the wheels?
View SolutionAn aeroplane is to go along straight line from A to B, and back again. The relative speed with respect to wind is V. The wind blows perpendicular to line AB with speed v. The distance between A and B is l. The total time for the round trip is:
View SolutionAn eaeroplane is to go along straight line from A to B, and back again. The relative speed with respect to wind is V. The wind blows perpendicular to line AB with speed v. The distance between A and B is l. The total time for the round trip is:
View SolutionA man travelling towards east at 8km/h finds that the wind seems to blow directly from the north On doubling the speed, he finds that it appears to come from the north-east. Find the velocity of the wind.
View SolutionA sound source and listener are both stationary and a strong wind is blowing. Is there a Doppler effect?
View SolutionA boat is moving towards east velocity 4 m/s w.r.t still water and river is flowing towards north with velocity 2 m/s and the wind blowing towards north with velocity 6 m/s. the direction of the flag blown over by the wind hpisted on the boat is
View Solution
