An alternating voltage (in volts) given by V=200√2sin(100t) is connected to 1μF capacitor through an ideal ac ammeter in series. The reading of the ammeter and the average power consumed in the circuit shall be
20 mA, 0
20 mA, 4W
20√2mA,8W
20√2mA,4√2W
The correct Answer is:A
To solve the problem, we need to find the reading of the ammeter and the average power consumed in the circuit when an alternating voltage is applied to a capacitor. Let's break it down step by step.
Step 1: Identify the given values
The alternating voltage is given by:
V=200√2sin(100t)
The capacitance of the capacitor is:
C=1μF=1×10−6F
Step 2: Determine the peak voltage (V0) and angular frequency (ω)
From the voltage equation, we can identify:
- Peak voltage V0=200√2 volts
- Angular frequency ω=100 rad/s
Step 3: Calculate the RMS voltage (Vrms)
The RMS voltage can be calculated using the formula:
Vrms=V0√2
Substituting the value of V0:
Vrms=200√2√2=200 volts
Step 4: Calculate the capacitive reactance (Xc)
The capacitive reactance is given by the formula:
Xc=1ωC
Substituting the values of ω and C:
Xc=1100×1×10−6=110−4=104 ohms
Step 5: Calculate the RMS current (Irms)
Using the formula for current in an AC circuit:
Irms=VrmsXc
Substituting the values:
Irms=200104=20×10−3 A=20 mA
Step 6: Calculate the average power consumed (P)
The average power consumed in a capacitor circuit is given by:
P=Vrms⋅Irms⋅cos(ϕ)
In a purely capacitive circuit, the phase difference ϕ is 90∘, so:
cos(90∘)=0
Thus, the average power consumed is:
P=200⋅20×10−3⋅0=0 watts
Final Answers:
- The reading of the ammeter is 20 mA.
- The average power consumed in the circuit is 0 watts.
The alternating voltage is given by:
V=200√2sin(100t)
The capacitance of the capacitor is:
C=1μF=1×10−6F
From the voltage equation, we can identify:
- Peak voltage V0=200√2 volts
- Angular frequency ω=100 rad/s
The RMS voltage can be calculated using the formula:
Vrms=V0√2
Substituting the value of V0:
Vrms=200√2√2=200 volts
The capacitive reactance is given by the formula:
Xc=1ωC
Substituting the values of ω and C:
Xc=1100×1×10−6=110−4=104 ohms
Using the formula for current in an AC circuit:
Irms=VrmsXc
Substituting the values:
Irms=200104=20×10−3 A=20 mA
The average power consumed in a capacitor circuit is given by:
P=Vrms⋅Irms⋅cos(ϕ)
In a purely capacitive circuit, the phase difference ϕ is 90∘, so:
cos(90∘)=0
Thus, the average power consumed is:
P=200⋅20×10−3⋅0=0 watts
- The reading of the ammeter is 20 mA.
- The average power consumed in the circuit is 0 watts.
Topper's Solved these Questions
Similar Questions
An alternating voltage E=200√2sin(100t) is connected to a 1 microfarad capacitor through an AC ammeter. The reading of the ammeter shall be
View SolutionAn alternating voltage E=200√2sin(100t) is connected to a 1 microfarad capacitor through an AC ammeter. The reading of the ammeter shall be
View SolutionAn alternating voltage given by V=300√2sin(50t) (in volts) is connected across a 1μF capacitor through an AC ammeter. The reading of the ammeter will be
View SolutionWhen a voltage Vs=200√2sin(100t)V is applied to an ac circuit the current in the circuit is found to be i=2sin[ωt+(π/4)]A. Find the average power consumed in the circuit.
View SolutionWhen a voltage Vs=200√2sin(100t)V is applied to an ac circuit the current in the circuit is found to be i=2sin[ωt+(π/4)]A. Find the average power consumed in the circuit.
View SolutionAn alternating voltage given as, V=100√2sin100t V is applied to a capacitor of 1μF. The current reading of the ammeter will be equal to ................ mA.
View SolutionIn an ac circuit an alternating voltage e=200√2sin100t volts is connected to a capacitor of capacity 1 μF. The rms.value of the current in the circuit is
View SolutionIn an ac circuit , an alternating voltage e=200√2sin100t volts is connected to a capacitor of capacitance 1μF. The rms value of the current in the circuit is :
View SolutionIn an AC circuit , an alternating voltage e = 200√2 sin 100t V is connected to a capacitor of capacity 1μF . The rms value of the current in the circuit is
View SolutionFigure below shows a capacitor C, an inductor L and a resistor R, connected in series to an a.c. supply of 220 V.
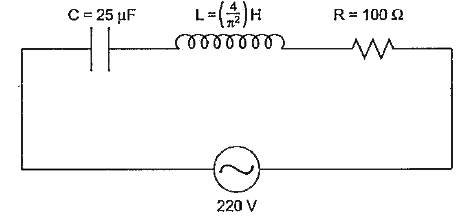
Average power consumed by the circuit.
View Solution
