An electric dipole of momentum →p is placed in a uniform electric field. The dipole is rotated through a very small angle θ from equilibrium and is released. It executes simple harmonic motion with frequency f=12π√pEI where, I= moment of interia of the dipole.
 Solution in Gujrati
Solution in Gujrati
A dipole makes an angle 9 with an electric field from its equilibrium position. So, torque acting on it,
→τ=→p×→E
∴τ=pEsinθ
This torque rotates the dipole in clockwise direction
∴τ=−pEsinθ
Here θ is very small.
∴sinθ=θ
∴τ=−pEθ
but, τ=1α and α=−ω2θ
1α=−pEθ, where α is the angular acceleration in S.H.M.
∴1(−ω2θ)=−pEθ
∴ω=√pE1
∴2πf=√pE1(∴ω=2πf)
∴f=12π√pE1
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION C NCERT EXEMPLAR SOLUTION (MULTIPLE CHOICE QUESTIONS (MCQs))
7 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION C NCERT EXEMPLAR SOLUTION (MULTIPLE CHOICE QUESTIONS (MORE THAN ONE OPTION))
6 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION B NUMERICALS (ADDITONAL EXERCISE)
10 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - KUMAR PRAKASHANCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section-D (MCQs asked in GUJCET/Board Exam)
1 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section D MCQs (MCQs asked in Competitive Exams )
38 Videos
Similar Questions
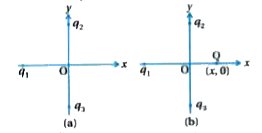
In figure, two positive charges q2 and q3 fixed along the y-axis, exert a net electric force in the + x direction on a charge q1 fixed along the x-axis. If a positive charge Q is added at (x, 0), the force on q1
View SolutionIf ∮s→E.→dS=0 over a surface, then
View SolutionAn oil drop of 12 excess electrons is held stationary under a constant electric field of 2.55×104NC−1. (Millikan's oil drop experiment). The density of the oil is 1.26gcm−3. Estimate the radius of the drop, (g=9.81ms−2,e=1.60×10−19C).
View SolutionMass of photon in motion is ………
View SolutionThree pure inductance each of 3 H are connected as shown in figure. The equivalent inductance of this connection between points A and B is ......

View SolutionAn electric dipole of moment p is placed in the positive of stable equilibrium in uniform electric field of intensity E . It is rotated through an angle theta from the initial position. The potential energy of electric dipole in the final position is
View SolutionProve that the frequency of oscillation of an electric dipole of moment p and rotational inertia I for small amplitudes about its equilibrium position in a uniform electric field strength E is 12π√(pEI)
View SolutionAn electric dipole of moment →p is placed in a uniform electric field →E, with →p parallel to →E. It is then rotated by an angle θ. The work done is
View SolutionAn electric dipole is placed in an uniform electric field with the dipole axis making an angle θ with the direction of the electric field. The orientation of the dipole for stable equilibrium is
View SolutionAn electric dipole is situated in an electric field of uniform intensity E whose dipole moment is P and moment of inertial is I . IF the dipole is displaced by small angle θ then the angular frequency of its oscillation is
View SolutionAn electric dipole of moment p is placed in equilibrium position in a uniform electric field of intensity E. to rotate the dipole through an angle θ from its initial position, the required moment of the torque is _____________. [fill in the blank]
View SolutionAn electric dipole of dipole moment →P is placed parallel to the uniform electric field of intensity →E. On rotating it through 180∘ the amount of work done is ..... .
View SolutionAn electric dipole of moment P is placed in the position of stable equilibrium in uniform electric field of intensity E. It is rotated through an angle θ from the initial position. The potential energy of electric dipole in the position is
View SolutionAn electric dipole is placed in an uniform electric field with the dipole axis making an angle theta with the direction of the electric field. The orientation of the dipole for stable equilibrium is
View SolutionAn electric dipole of dipole moment vecP is placed in a uniform electric field vecE such that vecP is perpendicular to vecE . The work done to turn the dipole through an angle of 180^(@) is -
View Solution
