An inductor (L=20mH), a resistor (R=100Ω) and a battery (ε=10V) are connected in series. After along time the circuit is short- circuited and then the battery is disconnected. Find the current in the circuit 1ms after short- circuiting.
To solve the problem step by step, we will follow the process of finding the initial current I0, the time constant τ, and then using these values to find the current I after 1 ms.
Step 1: Determine the Initial Current I0
The initial current I0 in the circuit when the battery is connected can be calculated using Ohm's Law:
I0=ϵR
Where:
- ϵ=10V (voltage of the battery)
- R=100Ω (resistance)
Substituting the values:
I0=10V100Ω=0.1A
Step 2: Calculate the Time Constant τ
The time constant τ for an RL circuit is given by:
τ=LR
Where:
- L=20mH=20×10−3H (inductance)
- R=100Ω (resistance)
Substituting the values:
τ=20×10−3H100Ω=0.2ms
Step 3: Find the Current I After Short-Circuiting
After the circuit is short-circuited, the current I at time t can be calculated using the formula:
I=I0e−tτ
Where:
- t=1ms=0.001s
- τ=0.2ms=0.0002s
Substituting the values into the equation:
I=0.1A⋅e−0.0010.0002
Calculating the exponent:
−0.0010.0002=−5
Now substituting back into the equation:
I=0.1A⋅e−5
Using the value of e−5≈0.006737:
I≈0.1A⋅0.006737≈0.0006737A=0.6737mA
Final Answer
The current in the circuit 1 ms after short-circuiting is approximately:
I≈0.673mA
- ϵ=10V (voltage of the battery)
- R=100Ω (resistance)
- L=20mH=20×10−3H (inductance)
- R=100Ω (resistance)
- t=1ms=0.001s
- τ=0.2ms=0.0002s
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Worked out examples
29 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 1
11 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 2
7 Videos
- ELECTROMAGNETIC WAVESBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise
8 Videos
Similar Questions
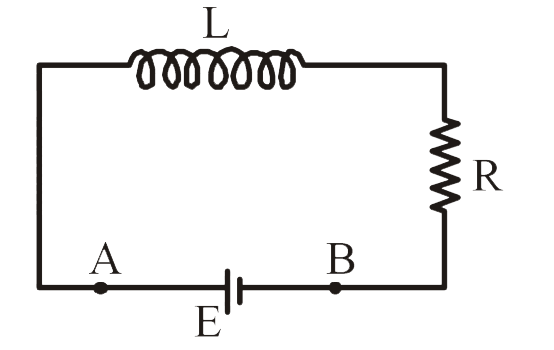
An inductor (L = 100 mH), a resistor (R=100Ω) and a battery (E = 100 V) are initially connected in series as shown in the figure. After a long time the battery is disconnected after short circuiting the points A and B. The current in the circuit 1 ms after the short circuit is
View SolutionAn inductor (L=20mH), a resistor (R=100Ω) and a battery(ɛ=10V) are connected in series. Find (a) the time constant, (b) the maximum current and (c ) the time elapsed before the current reaches 99% of the maximum value.
View SolutionAn inductor of 10mH is connected to a 18V battery through a resistor of 10kΩ and a switch. After a long time, when the maximum current is set up in the circuit, the battery is disconnected from the circuit. Calculate the current in the circuit after 15μs
View SolutionAn AC circuit has R= 100Ω , C= 2μ and L= 80 mH, connected in series. The quality factor of the circuit is :
View SolutionA satellite is launched into a circular orbit of radius R around the earth while a second satellite is launched into an orbit of radius 1.02R. The percentage difference in the time period is:
View SolutionAn inductor 20mH, a capacitor 50μF and a resistor 40Ωare connected in series across of emf V=10sin340t. The power loss in A.C. circuit is
View SolutionAn inductor of 10mH is connected to a 18V battery through a resistor of 10kΩ and a switch After a long time, when the maximum current is set up in the circuit, the current is switched off. Calculate the cur- rent in the circuit after 2 μs.
View SolutionThree resistors are connected to a 6 V battery as shown in the figure
Calculate:
total current in the circuit.
View SolutionFor the given circuit, the total energy stored in the circuit when a 12V battery is connected.(C=10μF)
_PHY_E05_125 _Q01.png)
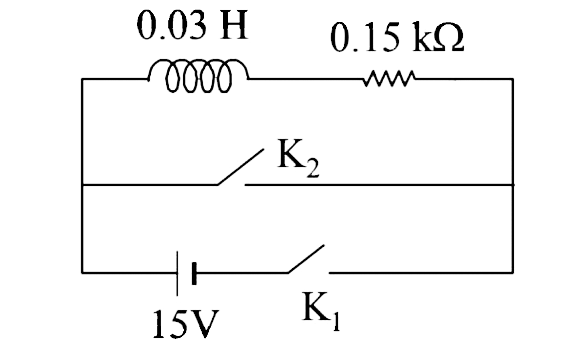
View SolutionAn inductor (L =0.03 H) and a resistor (R=0.15k(Ω)) are connected in series to a battery of 15 V EMF in a circuit shown below. The key K1 is opened and Key K2 is closed simultaneously. At t =1 ms, the current in the circuit will be (e5=150)
View Solution
