The battery discussed in the previous question is suddenly disconnected. Is a current induced in the other loop? If yes, when does it start and when does it end? Do the loops attract each other or repel?
To solve the question, we will analyze the situation step by step:
Step 1: Understanding the Initial Condition
Initially, we have two loops, and one of them is connected to a battery, allowing current to flow through it. This current creates a magnetic field around the loop.
Hint: Remember that a current-carrying conductor produces a magnetic field around it.
Step 2: Disconnecting the Battery
When the battery is suddenly disconnected from the first loop, the current in that loop will start to decrease. This change in current leads to a change in the magnetic field associated with that loop.
Hint: Consider how a change in current affects the magnetic field produced by the loop.
Step 3: Induction of Current in the Second Loop
According to Faraday's law of electromagnetic induction, a change in magnetic flux through a loop induces an electromotive force (emf) in that loop. Since the magnetic field from the first loop is decreasing when the battery is disconnected, this change will induce a current in the second loop.
Hint: Recall Faraday's law: A change in magnetic flux induces an emf in a closed loop.
Step 4: Direction of the Induced Current
Using Lenz's law, we can determine the direction of the induced current. Lenz's law states that the induced current will flow in a direction that opposes the change in magnetic flux. Since the magnetic field is decreasing, the induced current in the second loop will flow in the same direction as the original current in the first loop to try to maintain the magnetic field.
Hint: Lenz's law helps us find the direction of the induced current based on the change in magnetic flux.
Step 5: Duration of the Induced Current
The induced current will start flowing in the second loop the moment the battery is disconnected and the magnetic field begins to decrease. This induced current will last only for a brief moment until the magnetic field completely dissipates, which occurs shortly after the disconnection.
Hint: Think about how quickly the magnetic field can change and how that affects the duration of the induced current.
Step 6: Interaction Between the Loops
Since the induced current in the second loop flows in the same direction as the current in the first loop (which was clockwise), the two loops will attract each other. This is because parallel currents attract.
Hint: Remember the rule: like currents attract, and opposite currents repel.
Final Summary
- Yes, a current is induced in the second loop when the first loop's battery is disconnected.
- The induced current starts as soon as the magnetic field begins to decrease and ends shortly after the magnetic field dissipates.
- The loops will attract each other since the induced current in the second loop flows in the same direction as the original current in the first loop.
Initially, we have two loops, and one of them is connected to a battery, allowing current to flow through it. This current creates a magnetic field around the loop.
When the battery is suddenly disconnected from the first loop, the current in that loop will start to decrease. This change in current leads to a change in the magnetic field associated with that loop.
According to Faraday's law of electromagnetic induction, a change in magnetic flux through a loop induces an electromotive force (emf) in that loop. Since the magnetic field from the first loop is decreasing when the battery is disconnected, this change will induce a current in the second loop.
Using Lenz's law, we can determine the direction of the induced current. Lenz's law states that the induced current will flow in a direction that opposes the change in magnetic flux. Since the magnetic field is decreasing, the induced current in the second loop will flow in the same direction as the original current in the first loop to try to maintain the magnetic field.
The induced current will start flowing in the second loop the moment the battery is disconnected and the magnetic field begins to decrease. This induced current will last only for a brief moment until the magnetic field completely dissipates, which occurs shortly after the disconnection.
Since the induced current in the second loop flows in the same direction as the current in the first loop (which was clockwise), the two loops will attract each other. This is because parallel currents attract.
- Yes, a current is induced in the second loop when the first loop's battery is disconnected.
- The induced current starts as soon as the magnetic field begins to decrease and ends shortly after the magnetic field dissipates.
- The loops will attract each other since the induced current in the second loop flows in the same direction as the original current in the first loop.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE
98 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 2
7 Videos
- ELECTROMAGNETIC WAVESBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise
8 Videos
Similar Questions
Which one of the following pairs of reactantas does not form oxygen when they react with each other ?
View Solution12 cells each having the same emf are connected in series and are kept in a closed box. Some of the cells are wrongly connected. This battery is connected in series with an ammeter and two similar cells . The current is 3A when the two cells aid the battery and is 2A when the cells and the battery oppose each other. How many cells in the battery are wrongly connected?
View SolutionA pilot does not fall down when his aeroplane loops the loop. Why ?
View Solution(a) A closed loop is held stationary in the magnetic field between the north and south poles of two permanent magnets held fixed. Can we hope to generate current in the loop by using very strong magnets?
(b) A closed loop moves normal to the constant electric field between the plates of a large capacitor. Is a current induced in the loop (i) when it is wholly inside the region between the capacitor plates (ii) when it is partially outside the plates of the capacitor? The electric field is normal to the plane of the loop.
(c) A rectangular loop and a circular loop are moving out of a uniform magnetic field region (Fig. 6.8) to a field-free region with a constant velocity v. In which loop do you expect the induced emf to be constant during the passage out of the field region? The field is normal to the loops.

(d) Predict the polarity of the capacitor in the situation described by Fig. 6.9.

View SolutionTwo loops are facing each other as shown in Figure. State whether the loops will attract each other or repel each other if current I1 is increased
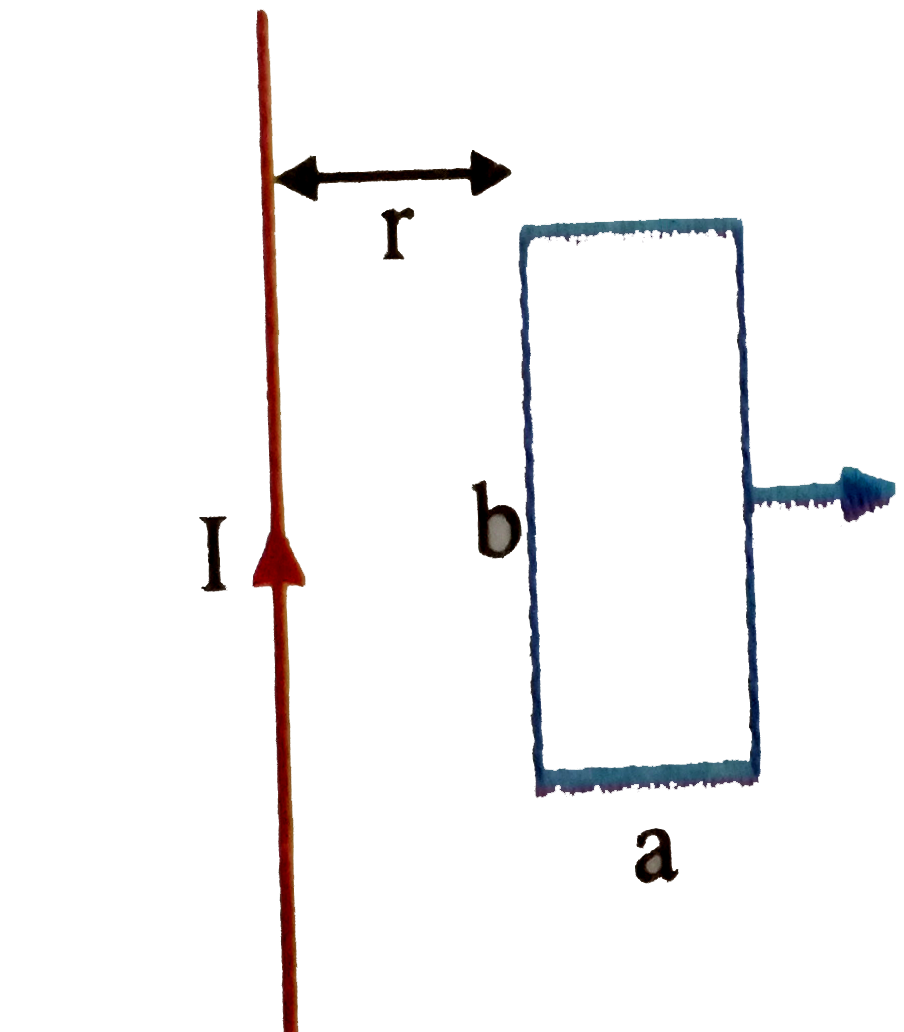
View SolutionA rectangular loop of wire with dimensions shown in figure is coplanar with a long wire carrying current 'I'. The distance between the wire and the left side of the loop is r. The loop is pulled to the right as indicated. What are the directions of the induced current in the loop and the magnetic forces on the left and right sides of the loop when the loop is pulled ?
View SolutionWhen air is blown In between two balls suspended close to each other they are attracted towards each other why?
View SolutionA square metallic loop of side l is placed near a fixed long wire carrying a current i(figure).The loop is moved towards right perpendicular to the wire with a speed v in the plane containing the wire and the loop.The emf induced in the loop when the rear end of the loop is at a distance a=2l from the wire is μ0ivxπ.Find out value of x.

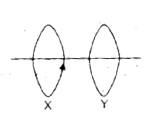
View SolutionThe two loops shown in the figure have their planes parallel to each other . A clockwise current flows in the loop X as viewed from X towards Y. The two coils will repel each other if the current in the loop X is :
View SolutionTwo proton beams going in the same direction repel each
other whereas two wires carrying currents in the same
direction attract each other. Explain.
View Solution
