An inductor of inductance L and another resistor of resistance R are connected in series with a battery of emf E and a switch. Switch is closed at t = 0. How much charge will pass through the battery in one time constant? Internal resistance of the battery is negligible.
ELeR2
ELeR
2ELeR2
ELR2
The correct Answer is:A
To solve the problem of how much charge will pass through the battery in one time constant in an LR circuit, we can follow these steps:
1. Understand the Circuit:
We have an inductor (L) and a resistor (R) connected in series with a battery of emf (E) and a switch. When the switch is closed at time t=0, current starts to flow through the circuit.
2. Current in the Circuit:
The current I(t) in an LR circuit can be expressed as:
I(t)=ER(1−e−tτ)
where τ=LR is the time constant of the circuit.
3. Charge Flow:
The charge Q that passes through the circuit in a time interval can be found by integrating the current over that time interval. The relationship between charge and current is given by:
Q=∫t0I(t′)dt′
4. Substituting Current:
Substitute the expression for I(t) into the integral:
Q=∫τ0ER(1−e−t′τ)dt′
5. Integrate:
We can split the integral into two parts:
Q=ER∫τ0dt′−ER∫τ0e−t′τdt′
The first integral evaluates to:
∫τ0dt′=τ
The second integral can be evaluated using the formula for the integral of an exponential:
∫e−axdx=−1ae−ax
Thus,
∫τ0e−t′τdt′=−τe−t′τ∣∣∣τ0=−τ(e−1−1)=τ(1−e−1)
6. Final Charge Calculation:
Putting it all together:
Q=ER(τ−τ(1−e−1))
Simplifying gives:
Q=ERτe−1
Since τ=LR:
Q=ELR2e−1
Conclusion:
The charge that passes through the battery in one time constant is:
Q=ELR2(1−e−1)
We have an inductor (L) and a resistor (R) connected in series with a battery of emf (E) and a switch. When the switch is closed at time t=0, current starts to flow through the circuit.
The current I(t) in an LR circuit can be expressed as:
I(t)=ER(1−e−tτ)
where τ=LR is the time constant of the circuit.
The charge Q that passes through the circuit in a time interval can be found by integrating the current over that time interval. The relationship between charge and current is given by:
Q=∫t0I(t′)dt′
Substitute the expression for I(t) into the integral:
Q=∫τ0ER(1−e−t′τ)dt′
We can split the integral into two parts:
Q=ER∫τ0dt′−ER∫τ0e−t′τdt′
∫τ0dt′=τ
∫e−axdx=−1ae−ax
Thus,
∫τ0e−t′τdt′=−τe−t′τ∣∣∣τ0=−τ(e−1−1)=τ(1−e−1)
Putting it all together:
Q=ER(τ−τ(1−e−1))
Simplifying gives:
Q=ERτe−1
Since τ=LR:
Q=ELR2e−1
The charge that passes through the battery in one time constant is:
Q=ELR2(1−e−1)
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITION FILE (MULTIPLE CHOICE QUESTIONS) (AIPMT/NEET & Other State Boards for Medical Entrance)8videos
ELECTROMAGNETIC INDUCTION
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITION FILE (MULTIPLE CHOICE QUESTIONS) (JEE (Main) & Other State Boards for Engineering Entrance)38videos
ELECTROMAGNETIC INDUCTION
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Numerical Problems)16videos
ELECTROMAGNETIC INDUCTION
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test15videos
ELECTRIC CHARGES AND FIELDS
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST14videos
ELECTROMAGNETIC WAVES
Similar Questions
An inductor of self-inductance L and resistor of resistance R are connected in series to a battery of emf E and negligible resistance.Calculate the maximum rate at which energy is stored in the inductor.
View SolutionA coil of inductance L and resistance R is connected to a battery of emf E. The final current in the coil is ______.
View Solution
Knowledge Check
- Question 1 - Select One
In the circuit shown in figure switch S is closed at time t=0. The charge which passes through the battery in one time constant is
 AeR2ELBE(LR)CELeR2DeLER
AeR2ELBE(LR)CELeR2DeLER - Question 2 - Select One
An inductance L and and a resistance R are connected in series to a battery of voltage V and negligible internal resistance through a switch. The switch is closed at t=0. The charge that passes through the battery in one time constant is [e is base of natural logarithms]
AeR2VLBVLRCVLeR2DeLVR - Question 3 - Select One or More
An inductor L and a resistance R are connected in series with a battery of emf E and a switch. Initially the switch is open. The switch is closed at an instant t = 0. Select the correct alternatives
AAt t = 0, induced emf in inductor is zeroBAt t=LR, energy stored in the inductor is (1−1e)2(LE22R2)CAt t=LR, induced emf in the inductor is EeDAt t=LR, voltage drop across the resistor is E(1−12)
An inductance L and a resistance R are connected in series with a battery of emf ε. The maximum rate at which the energy is stored in the magnetic field is :
View SolutionAn inductance L and a resistance R are connected in series with a battery of emf ε. The maximum rate at which the energy is stored in the magnetic field is :
View SolutionSolenoid of self-inductance L is connected in series with a resistor of resistance R with a battery and switch. Switch is closed at t = 0. How much time will be taken by inductor to acquire one-fourth of its maximum energy?
View SolutionA cricuite contains an inductance L, a resistance R and a battery of emf E. The circulit is switched on at t=0 . The change flows through the battery in one time constant (τ) is-
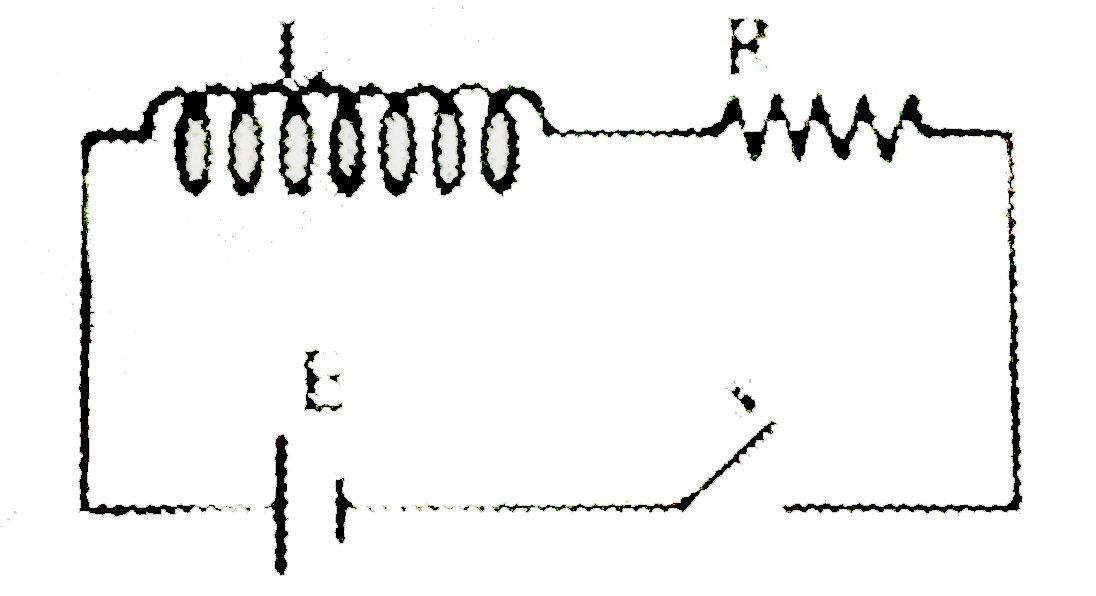 View Solution
View SolutionThe switch shown in figure is closed at t=0 find the charge throuh battery till time t=LR
_2019_CBT8_E01_016_Q01.png) View Solution
View Solution

