An isosceles triangular plates of base 3 m and altitude 3 m is immersed in oil vertically with its base coinciding with the free surface of the oil of relative density 0.8. Determine the total thrust.
To determine the total thrust exerted by the oil on the isosceles triangular plate, we can follow these steps:
Step 1: Determine the density of the oil
The relative density of the oil is given as 0.8. The density of water is approximately 1000kg/m3. Therefore, the density of the oil can be calculated as:
ρoil=Relative Density×Density of Water=0.8×1000kg/m3=800kg/m3
Step 2: Find the depth of the center of mass of the triangular plate
The height of the triangular plate is given as 3 m. For an isosceles triangle, the center of mass is located at a distance of h3 from the base. Thus, the depth of the center of mass from the free surface of the oil is:
Depth of center of mass=3m3=1m
Step 3: Calculate the pressure at the center of mass
The pressure exerted by the fluid at a certain depth is given by the formula:
P=ρgh
Where:
- ρ is the density of the fluid (oil).
- g is the acceleration due to gravity (approximately 10m/s2).
- h is the depth of the center of mass.
Substituting the values:
P=800kg/m3×10m/s2×1m=8000Pa
Step 4: Calculate the area of the triangular plate
The area A of the triangular plate can be calculated using the formula for the area of a triangle:
A=12×base×height=12×3m×3m=92m2=4.5m2
Step 5: Calculate the total thrust
The total thrust F exerted by the fluid on the plate can be calculated using the formula:
F=P×A
Substituting the values we found:
F=8000Pa×4.5m2=36000N
Final Result
Thus, the total thrust exerted by the oil on the triangular plate is:
F=36000Nor3.6×104N
---
The relative density of the oil is given as 0.8. The density of water is approximately 1000kg/m3. Therefore, the density of the oil can be calculated as:
The height of the triangular plate is given as 3 m. For an isosceles triangle, the center of mass is located at a distance of h3 from the base. Thus, the depth of the center of mass from the free surface of the oil is:
The pressure exerted by the fluid at a certain depth is given by the formula:
- ρ is the density of the fluid (oil).
- g is the acceleration due to gravity (approximately 10m/s2).
- h is the depth of the center of mass.
The area A of the triangular plate can be calculated using the formula for the area of a triangle:
The total thrust F exerted by the fluid on the plate can be calculated using the formula:
Thus, the total thrust exerted by the oil on the triangular plate is:
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.2
11 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.3
10 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Solved Examples
7 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
An isosceles triangle made of wood of base 10 feet and height 8 feet is placed vertically with its base on the ground and vertex directly above it. If the triangle faces the sun whose altitude is 30∘, then the tangent of the angle at the apex of the shadow is
View SolutionAn isosceles triangle of wood of base 10 feet and height 8√3 feet is placed vertically with its base on the ground and vertex directly above. The triangle faces the sun whose altitude is 30∘. Then, the tangent of the angle at the apex of the shadow is
View SolutionConstruct an isosceles triangle whose base is 8 cm and altitude 4 cm and then another triangle whose sides are 34 times the corresponding sides of the isosceles triangle.
View SolutionConstruct an isosceles triangle whose base is 8cm and altitude 4cm and then another triangle whose sides are 3/2 times the corresponding sides of the isosceles triangle.
View SolutionConstruct an isosceles triangle whose base is 8cm and altitude 4cm and then construct another triangle whose sides are 32 times the corresponding sides of the isosceles triangle.
View SolutionThe figure shows an isosceles triangle plate of mass M and base L.
The angle at the apex is 90∘. The apex lies at the origin and the base is parallel to X−axis.
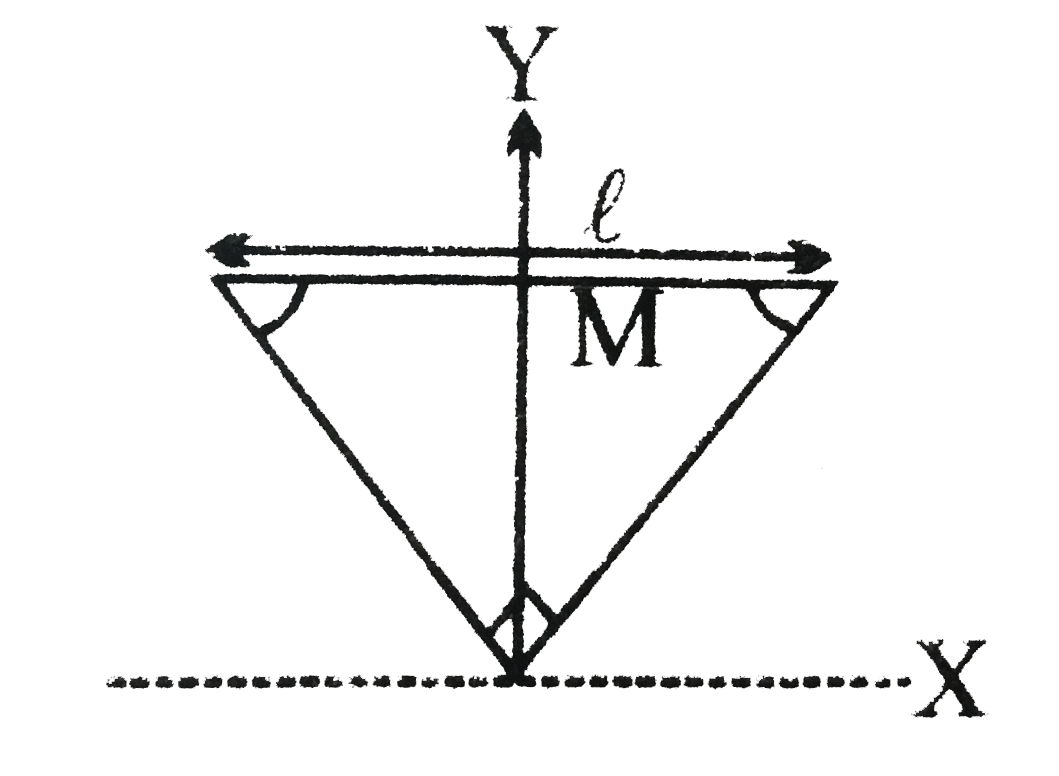
The moment of inertia of the plate about its base parallel to the x−axis is
View SolutionThe area of a triangle is 50 cm2 If the altitude is 8 cm, what is its base?
View SolutionCurved surface area of a cone is 308 cm2 and its slant height is 14cm. Find the radius of the base and total surface area of the cone.
View SolutionCurved surface area of a cone is 308 cm2 and its slant height is 14cm. Find the radius of the base and total surface area of the cone.
View Solution_S01_015_Q01.png)
A vertical U-tube of uniform cross-section contains mercury in both arms A glycerine (relative density =1.3) column of length 10 cm is introduced into one of the arms. Oil of density 800 gm m−3 is poured into the other arm until the upper surface of the oil and hlycerine are at the same horizontal level, find the length of the column. Density of mercury is 13.6×103kgm−3
View Solution
