Figure shows a semi-cylindrical massless gate pivoted at the point O holding a stationary liquid of density ρ. The length of the cylinder is l. Calculate, the horizontal force exerted by the liquid on the gate.
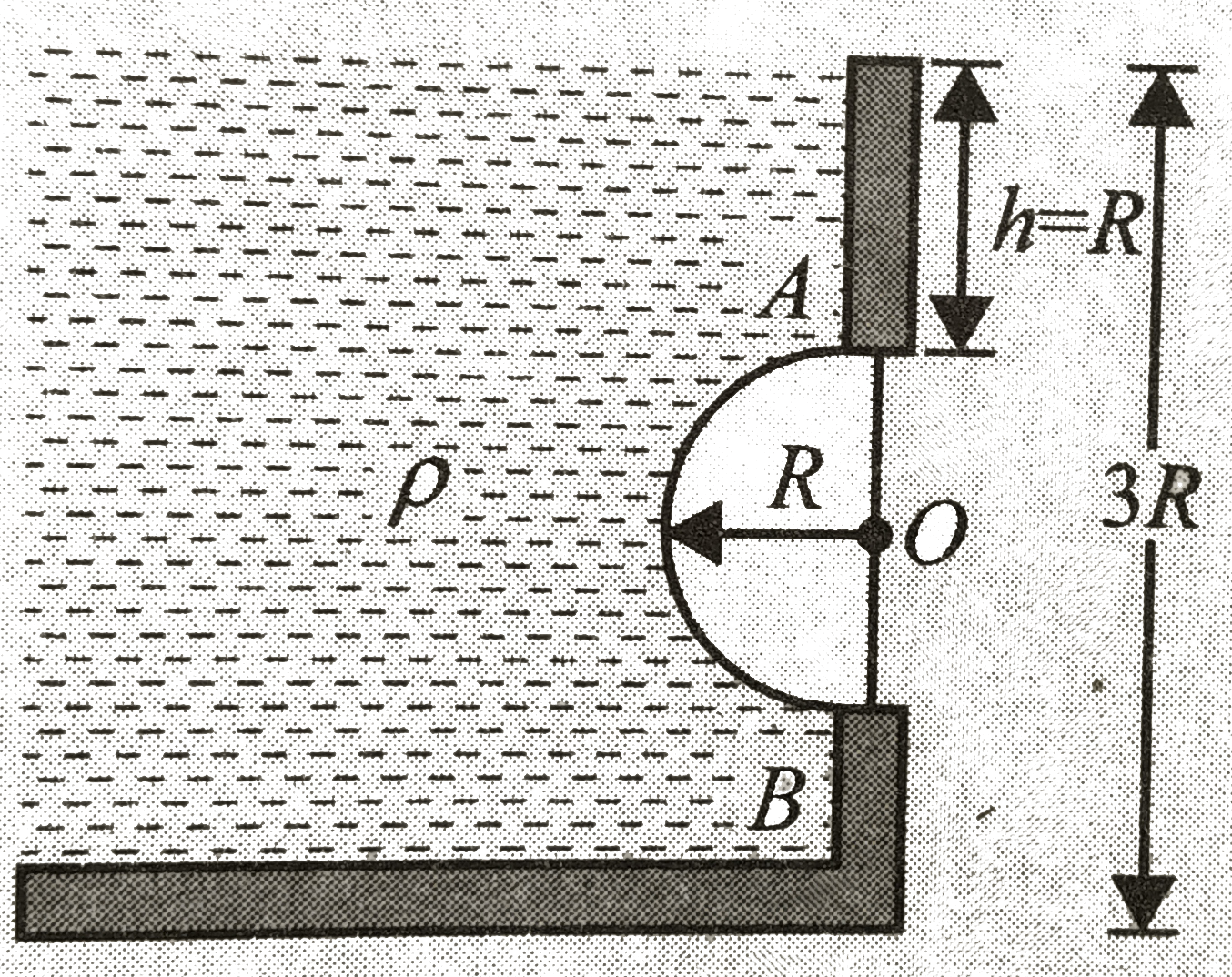
The force exerted by liquid on the gate is
fH=Pav×vertical projection area of the gate
=(PA−PB2)×(2Rl)
=(Rρg+3Rρg2)×2Rl=4ρgR2l
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.2
11 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.3
10 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Solved Examples
7 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A light semi cylindrical gate of radius R is pivoted aat its mid point O, of radius R as shown in the figure holding liquid of density ρ. The force F required to prevent the rotation of the gate is equal to

View SolutionThe force F needed to support the liquid of density ρ and the vessel on it, in figure, is
View Solution
Knowledge Check
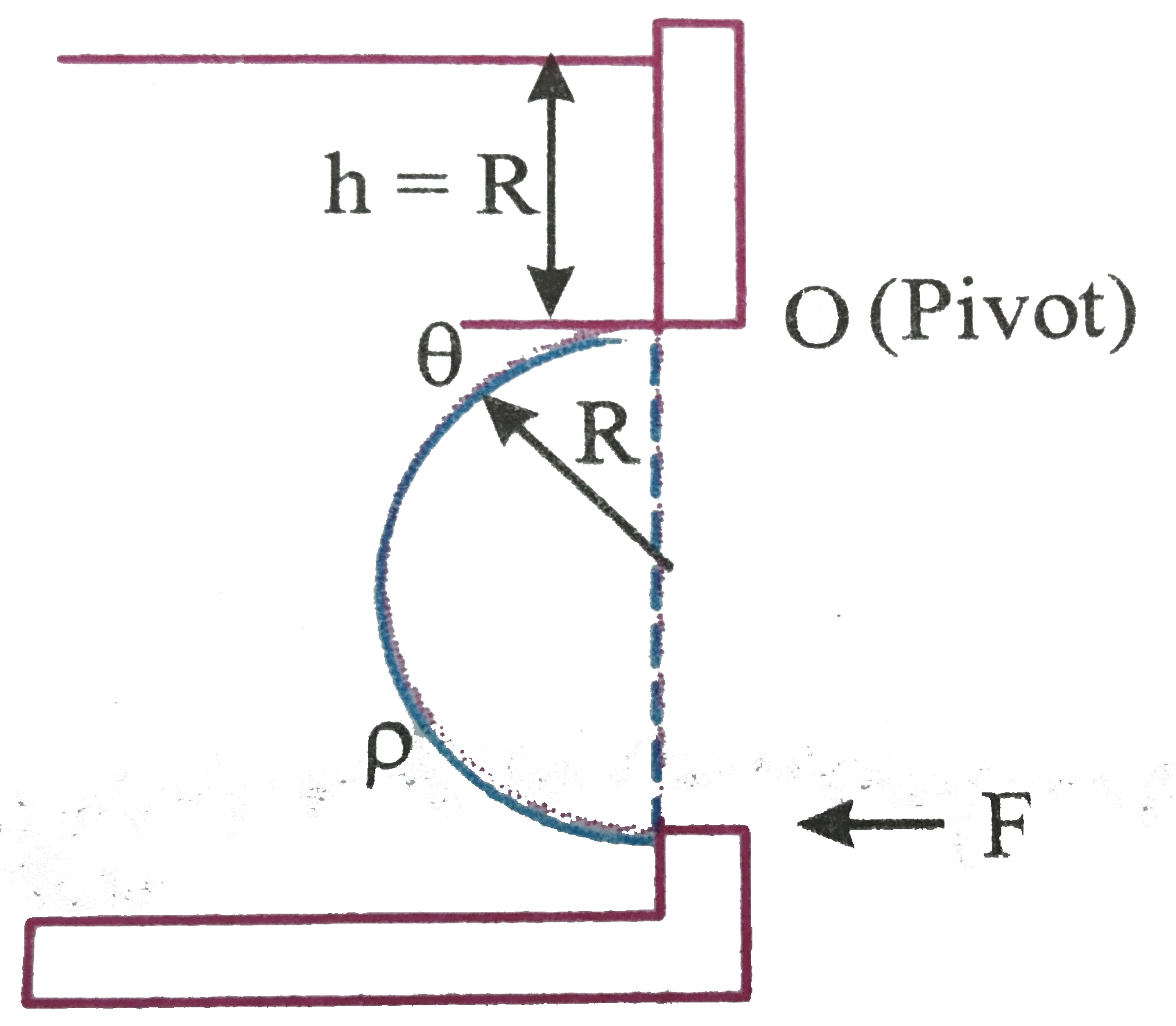
The fig shows a semo-cylinderical massless gate of unit length perpendicular to the plane of the page and is pivoted at the point O holding a stationary liquid of density ρ. A horizontal foce F is applied at its lowest position to keep it stationary. The magnitude of the force is:A32ρgR2B92ρgR2CρgR2D2ρgR2A light semi cylindrical gate of radius R is pivoted aat its mid point O, of radius R as shown in the figure holding liquid of density ρ. The force F required to prevent the rotation of the gate is equal to
 A2πR3ρgB2ρgR3C2R2ρg3DNone of these
A2πR3ρgB2ρgR3C2R2ρg3DNone of theseA liquid of density ρ comes out with a velocity v from a horizontal tube of area of cross-section A. The reaction force exerted by the liquid on the tube is F :
AF∝vBF∝v2CF∝ADF∝ρ
A square gate of size 1m×1m is hinged at its mid point. A fluid of density ρ fill the space to the left of the gate. The force F required to hold the gate stationary is
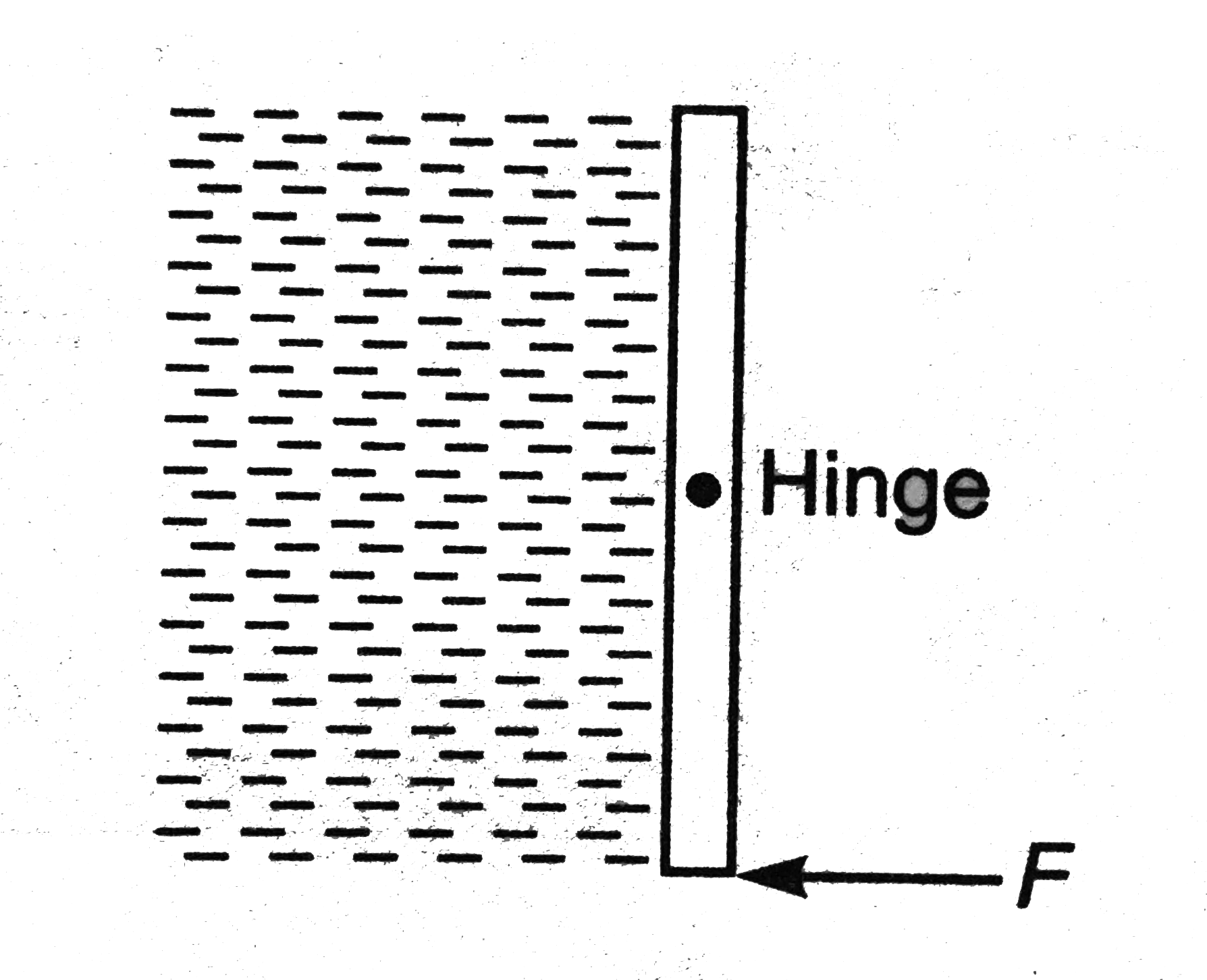
View SolutionA cylindrical vessel of radius r is filled with a homogeneous liquid upto a height h. It is found that the force exerted by the liquid column on the bottom of the cylinder is equal to the force exerted by the liquid on the sides of the cylinder. What is the relation between r and h?
View SolutionA light semi cylindrical gate of radius R is pivoted aat its mid point O, of radius R as shown in the figure holding liquid of density ρ. The force F required to prevent the rotation of the gate is equal to

View SolutionA square gate of size 1m×1m is hinged at its mid point. A fluid of density ρ fill the space to the left of the gate. The force F required to hold the gate stationary is

View SolutionA square gate of size 1m×1m is hinged at its mid point. A fluid of density ρ fill the space to the left of the gate. The force F required to hold the gate stationary is

View Solution
