An LR circuit having L=4.0H,R=1.0Ωandε=6.0V is switched on at t=0. find the power dissipated in joule heating at t=4.0s.
To find the power dissipated in an LR circuit at a given time, we can follow these steps:
Step 1: Identify the given values
We have the following values from the problem:
- Inductance, L=4.0H
- Resistance, R=1.0Ω
- Voltage, ϵ=6.0V
- Time, t=4.0s
Step 2: Calculate the steady-state current (I0)
The steady-state current (I0) can be calculated using Ohm's law:
I0=ϵR
Substituting the values:
I0=6.0V1.0Ω=6.0A
Step 3: Calculate the time constant (τ)
The time constant (τ) for an LR circuit is given by:
τ=LR
Substituting the values:
τ=4.0H1.0Ω=4.0s
Step 4: Calculate the current at t=4.0s
The current I(t) at any time t in an LR circuit is given by:
I(t)=I0(1−e−tτ)
Substituting the values:
I(4)=6.0(1−e−4.04.0)=6.0(1−e−1)
Using the approximation e−1≈0.3679:
I(4)=6.0(1−0.3679)≈6.0×0.6321≈3.7926A
Rounding off, we get:
I(4)≈3.8A
Step 5: Calculate the power dissipated
The power dissipated in the resistor can be calculated using the formula:
P=I2R
Substituting the values:
P=(3.8A)2×1.0Ω=14.44W
Final Answer
The power dissipated in joule heating at t=4.0s is approximately:
P≈14.44W
We have the following values from the problem:
- Inductance, L=4.0H
- Resistance, R=1.0Ω
- Voltage, ϵ=6.0V
- Time, t=4.0s
The steady-state current (I0) can be calculated using Ohm's law:
I0=ϵR
Substituting the values:
I0=6.0V1.0Ω=6.0A
The time constant (τ) for an LR circuit is given by:
τ=LR
Substituting the values:
τ=4.0H1.0Ω=4.0s
The current I(t) at any time t in an LR circuit is given by:
I(t)=I0(1−e−tτ)
Substituting the values:
I(4)=6.0(1−e−4.04.0)=6.0(1−e−1)
Using the approximation e−1≈0.3679:
I(4)=6.0(1−0.3679)≈6.0×0.6321≈3.7926A
Rounding off, we get:
I(4)≈3.8A
The power dissipated in the resistor can be calculated using the formula:
P=I2R
Substituting the values:
P=(3.8A)2×1.0Ω=14.44W
The power dissipated in joule heating at t=4.0s is approximately:
P≈14.44W
Topper's Solved these Questions
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Short Answer13videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 112videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE9videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises75videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercises9videos
ELECTROMAGNETIC WAVES
Similar Questions
Knowledge Check
- Question 1 - Select One
In a series LR circuit (L=3H,R=1.5Ω) and DC voltage =1V. Find current at T=2 seconds.
A0.1AB2AC3AD0.4A - Question 2 - Select One
In the circuit shown in figure L=10H,R=5Ω,E=15V. The switch S is closed at t=0. At t=2s the current in the circuit is
 A3(1−1e)AB3(1−1e2)AC3(1e)AD3(1e2)A
A3(1−1e)AB3(1−1e2)AC3(1e)AD3(1e2)A - Question 3 - Select One
In LR circuit, f = 50 Hz, L= 2 H, ε=5V,R=1Ω, then the energy stored in the inductor is:
A25 JB50 JC100 JDNone of these
A resistor of resistance 100Ω is connected to an AC source ε=(12V)sin(250πs−1)t. Find the energy dissipated as heat during t=0 to t=1.0ms.
View SolutionIn a series RC circuit with an AC source, R=300Ω,C=25μF,ε050V and v=50(Π)Hz. Find the peak current and the average power dissipated in the circuit.
View SolutionAn LR combination is connected to an idal battery. If l = 10 mH, R = 2.0 Omega and epsilon = 2.0 V, how much time will it take for the current ot reach 0.63 A?
View SolutionIn the circuit shown in figure, E = 10 V, r = 1Ω,
R = 4Ω and L = 5H. The circuit is closed at time t = 0.
Then, match the following two columns.
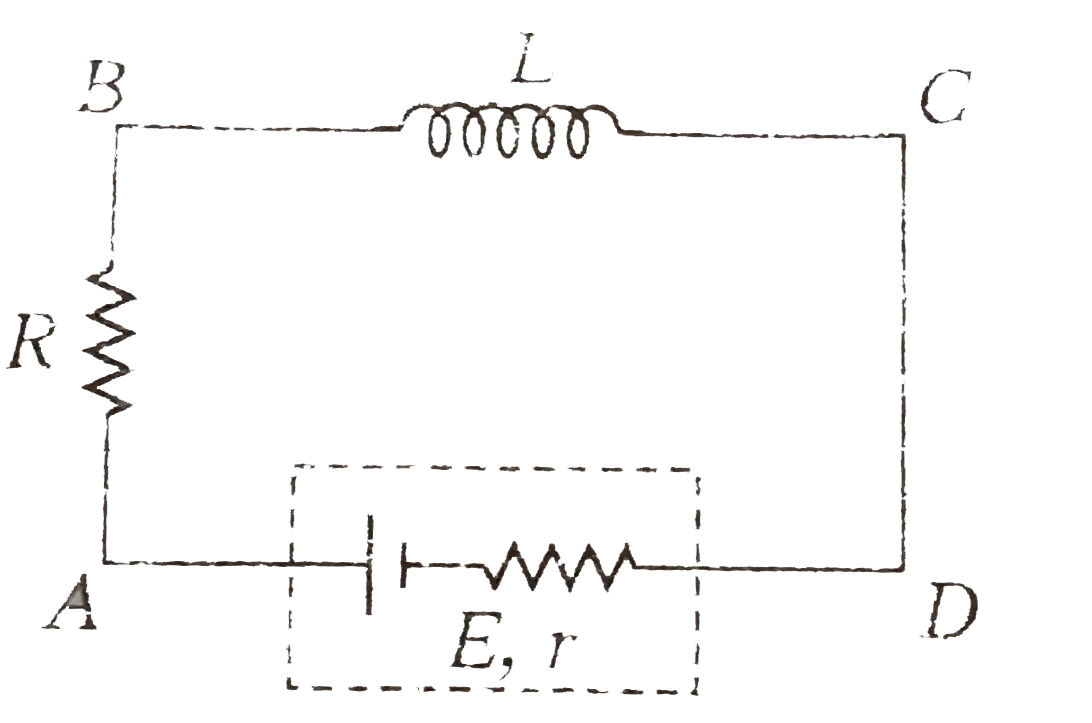
Column IColumn IIA.VAD at t = 0p4VB.VBC at t = 0q8VC.VAB at t =∞r.0VD.VAD at t=∞s.10VView SolutionSwitch is closed at t=0 then the current in the circuit at t=L2R is
_PHY_E06_157_Q01.png) View Solution
View Solution

