Question

An open lift is moving upward with velocity $10m/s.$ It has an upward acceleration of $2m/s^2.$ A ball is projected upwards with velocity $20m/s$ relative to ground. Find
(a) time when ball again meets the lift
(b) displacement of lift and ball at that instant.
Take $g=10m/s^2$

Explanation

(i) At the time when ball again meets the lift, `s_(L)=s_(B)`
`therefore 10 t+(1)/(2)xx2xxt^(2)=20t-(1)/(2)xx10t^(2)`
Solving this equation, we get

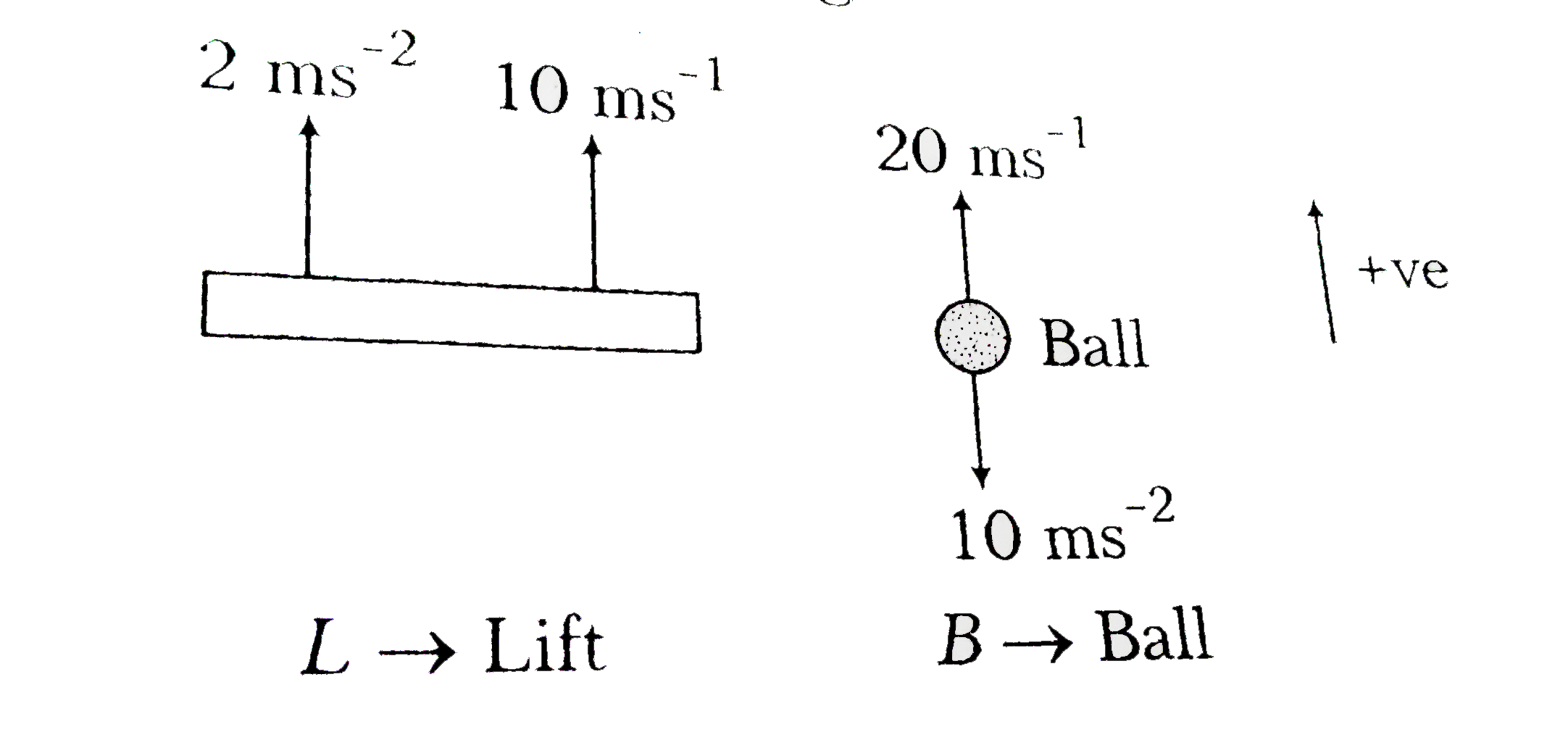
`therefore` Ball will again meet the lift after `(5)/(3)s`.
(ii) At this instant `s_(L)=s_(B)=10xx(5)/(3)+(1)/(2)xx2xx((5)/(3))^(2)`
`=(175)/(9)m=19.4 m`
(iii) For the ball `u uarr darr a`. Therefore we will first find `t_(0)`, the time when its velocity becomes zero.
`t_(0)=|(u)/(a)|=(20)/(10)=2s`
As `t(=(5)/(3)s)lt t_(0)`, distance and displacement are equal
or `d = 19.4 m`
Concept of relative motions is more useful in two body problem in two (or three) dimensional motion. This can be understood by the following example.