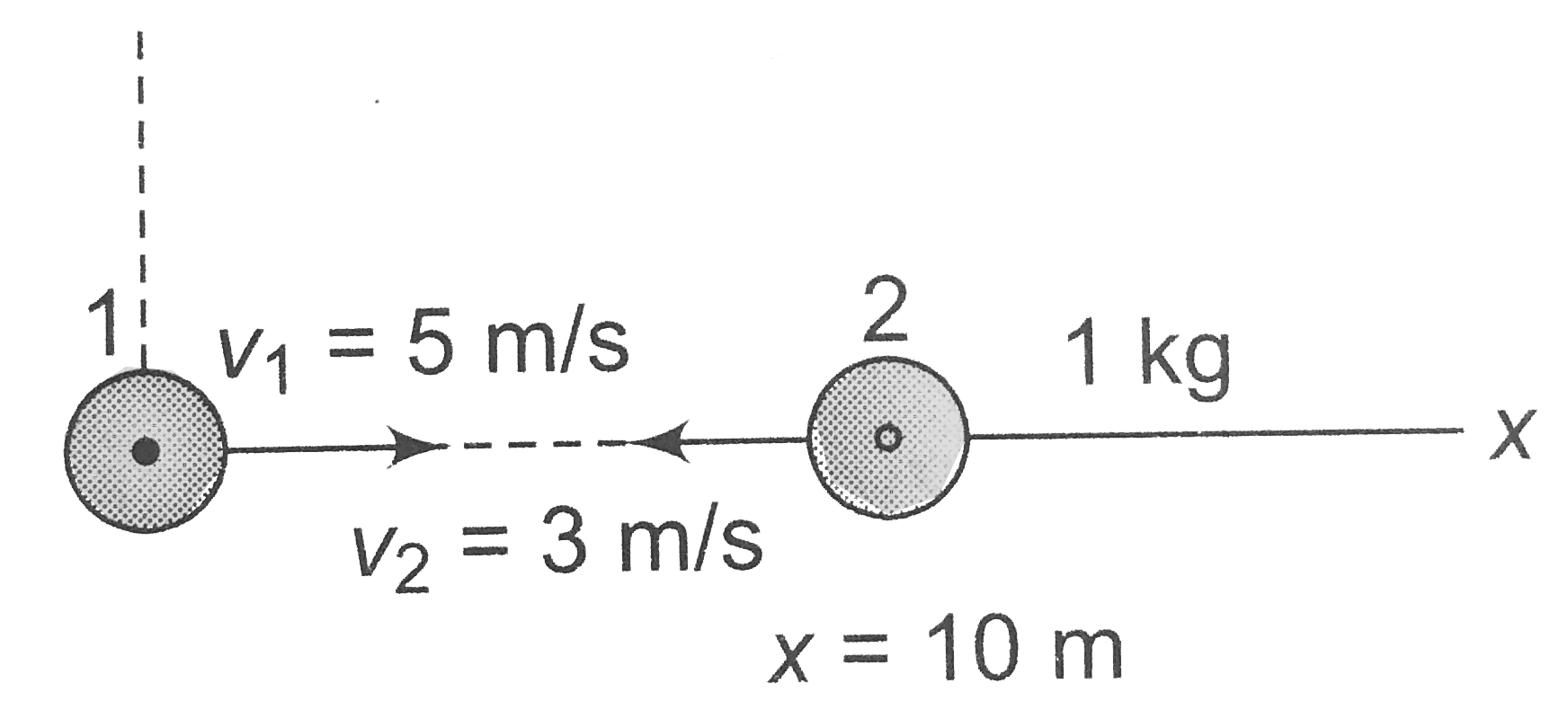
At , the positions and velocities of two particles are as shown in the figure. They are kept on a smooth surface and being mutually attracted by gravitational force. Find the position of centre of mass at .
 .
.
A
B
C
D
Video Solution
Text Solution
The correct Answer is:B
As no external force is acting on the system. The velocity of centre of the mass will be constant.
Displacement of the centre of mass
Hence, position of the centre of mass
|
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - MCQ_TYPE
20 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - LC_TYPE
34 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
Similar Questions
Knowledge Check
The position-time graph of a particle of mass 0.1 kg is shown. The impulse at t = 2s is :
 A0.2kgms−1B0.02kgms−1C0.1kgms−1D0.4kgms−1
A0.2kgms−1B0.02kgms−1C0.1kgms−1D0.4kgms−1The figure shows the positions and velocity of two particle. If the paritcle move under the mutual attraction of each other, then the position of centre of mass at t=1s is :-
 Ax=5mBx=6mCx=3mDx=2m
Ax=5mBx=6mCx=3mDx=2m
Find the position of centre of mass of the section shown in figure

View SolutionFind out the position of centre of mass of the figure shown below.
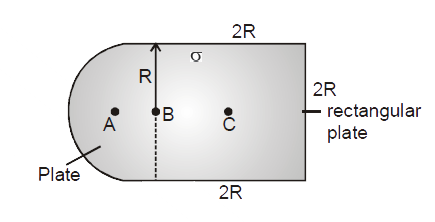
View SolutionFind out the position of centre of mass of the uniform lamina as shown in figure.
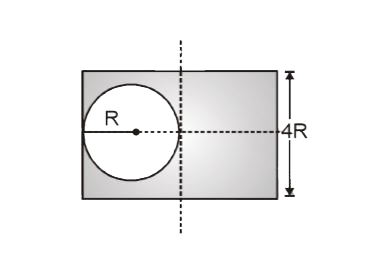
View SolutionFind the position of centre of mass of the uniform lamina shown in figure.

View SolutionFind the position of centre of mass of the T-shaped plate from O, in Fig.
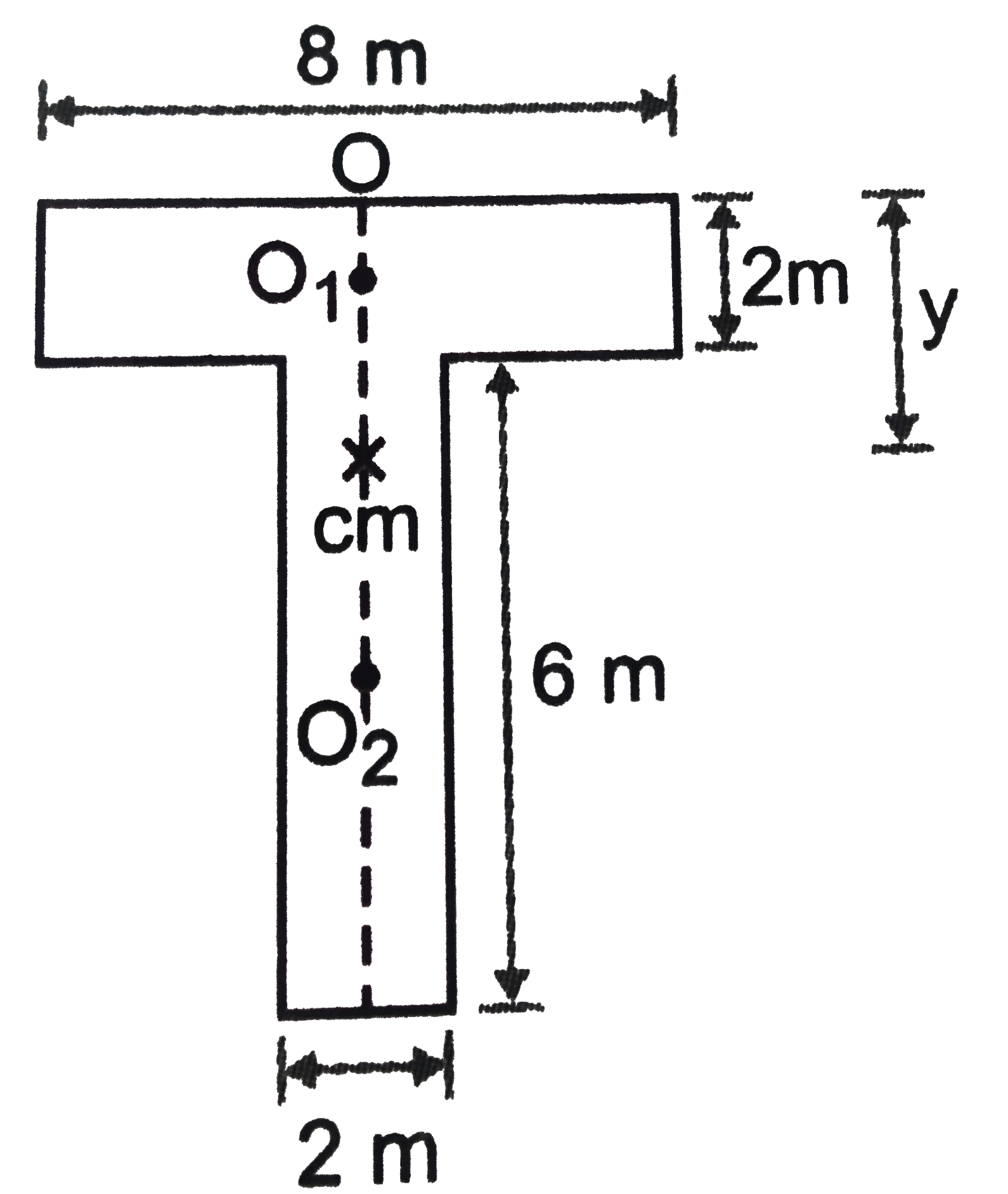
View SolutionFigure shows position and velocities of two particles moving under mutual gravitational attraction in space at time t=0. The position of centre of mass after one second is (in meters)
.

View Solution
