At t=0,a transverse wave pulse travelling in the positive x direction with a speed of 2m/s in a wire is described by the function y=6/x2 given that x≠0. Transverse velocity of a particle at x=2 m and t= 2 s is
3m/s
−3m/s
8m/s
−8m/s
The correct Answer is:b
To solve the problem step by step, we will follow these instructions:
Step 1: Understand the wave function
The wave function at time t=0 is given by:
y=6x2
This represents a transverse wave pulse traveling in the positive x-direction.
Step 2: Write the general wave equation
Since the wave is traveling in the positive x-direction, we can express the wave function at any time t as:
y=6(x−vt)2
where v is the speed of the wave. Given that the speed v=2m/s, we can substitute this into the equation:
y=6(x−2t)2
Step 3: Differentiate the wave function with respect to time
To find the transverse velocity of a particle, we need to differentiate y with respect to t:
dydt=ddt(6(x−2t)2)
Using the chain rule, we get:
dydt=6⋅ddt((x−2t)−2)=6⋅(−2)(x−2t)−3⋅(−2)=24(x−2t)3
Step 4: Substitute the values of x and t
We need to find the transverse velocity at x=2m and t=2s:
dydt=24(2−2⋅2)3=24(2−4)3=24(−2)3=24−8=−3m/s
Step 5: Conclusion
The transverse velocity of the particle at x=2m and t=2s is:
dydt=−3m/s
---
The wave function at time t=0 is given by:
y=6x2
This represents a transverse wave pulse traveling in the positive x-direction.
Since the wave is traveling in the positive x-direction, we can express the wave function at any time t as:
y=6(x−vt)2
where v is the speed of the wave. Given that the speed v=2m/s, we can substitute this into the equation:
y=6(x−2t)2
To find the transverse velocity of a particle, we need to differentiate y with respect to t:
dydt=ddt(6(x−2t)2)
Using the chain rule, we get:
dydt=6⋅ddt((x−2t)−2)=6⋅(−2)(x−2t)−3⋅(−2)=24(x−2t)3
We need to find the transverse velocity at x=2m and t=2s:
dydt=24(2−2⋅2)3=24(2−4)3=24(−2)3=24−8=−3m/s
The transverse velocity of the particle at x=2m and t=2s is:
dydt=−3m/s
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer1videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Multiple Correct25videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRAVELLING WAVESEXERCISE - Subjective16videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct9videos
TRANSMISSION OF HEAT
- BOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A wave pulse is travelling on a string at 2m/s along positive x-directrion. Displacement y of the particle at x = 0 at any time t is given by
y=2t2+1 Find
Shape of the pulse at t = 0 and t = 1s.View SolutionA transverse sinusoidal wave is moving along a string in the opposite direction of an x axis with a speed of 70m/s. At t=0 the string particle at x=0 has a transverse displacement of 4.0 cm and is not moving. The maximum transverse speed of the string particle at x=0 is 16m/s. (a) What is the frequency of the wave? (b) What is the wavelength of the wave? If y(x,t) =ymsin(kx±ωt+ϕ) is the form of the wave equation, what are (c) ym,(d)k,(e)ω(f)ϕ, and (g) the correct of sign in front of ω?
View Solution
Knowledge Check
- Question 1 - Select One
A wave travelling in positive x-direction with a=0.2 m/s. Velocity = 360 m/s. and λ = 60 m, then correct expression for the wave is
Ay=0.2sin[2π(6t+x60)]By=0.2sin[π(6t+x60)]Cy=0.2sin[2π(6t−x60)]Dy=0.2sin[π(6t−x60)] - Question 2 - Select One
A wave travelling in positive X-direction with A=0.2m has a velocity of 360m/sec . If λ=60m , then correct expression for the wave is
Ay=0.2sin[2π(6t+x60)]By=0.2sin[π(6t+x60)]Cy=0.2sin[2π(6t−x60)]Dy=0.2sin[π(6t−x60)] - Question 3 - Select One
A wave propagates in a string in the positive x-direction with velocity v. The shape of the string at t=t0 is given by
f(x,t0)=Asin(x2a2). Then the wave equation at any instant t is given byAg(x,t)=Asin[x−v(t−t0)]2a2Bg(x,t)=Asin[x+v(t−t0)]2a2Cg(x,t)=Asin[x−v(t+t0)]2a2Dg(x,t)=Asin[x+v(t+t0)]2a2
A symmetrical triangular pulse of maximum height 0.4m and total length 1 m is moving in the positive x-direction on a string on which the wave speed is 24 m/s. At t = 0 the pulse is entirely located between x = 0 and x = 1 m. Draw a graph of the transverse velocity of particle of string versus time at x = +1m.
View SolutionA wave represented by the given equationY=Asin(10πx+15πt+π3) , where x is in meter and t is in second. The expression represents
(1) A wave travelling in the negative X direction with a velocity of 1.5 m/sec
(2) A wave travelling in the negative X direction with a wavelength of 0.2 m
(3) A wave travelling in the positive X direction with a velocity of 1.5 m/sec.
(4) A wave travelling in the positive X direction with a wavelength of 0.2 mView SolutionA transverse wave travelling in a string produce maximum transverse velocity of 3m/s and maximum transverse acceleration 90m/s2 in a particle. If the velocity of wave in the string is 20m/s. Datermine the equation of the wave ?
View SolutionAt t=0, a transverse wave pulse in a wire is described by the function y=6/(x2−3) where x and y are in metres. The function y(x,t) that describes this wave equation if it is travelling in the positive x direction with a speed of 4.5m/s is
View SolutionA transverse sinusoidal wave of wavelength 20 cm is moving along a string towards increasing x. the transverse displacement of the string particle at x=0 as a function of time is shown in figure.
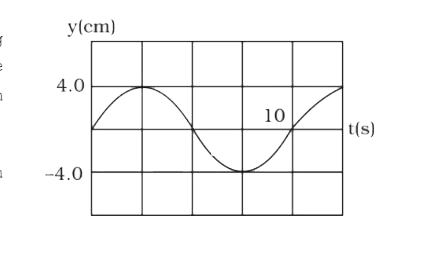
Q. The transverse velocity of the particle at x=0 at t=5.0 s is ?View Solution

