black colored solid sphere of radius "R" and mass "M" is inside a cavity with vacuum inside.The walls of the cavity are maintained at temperature T0 .The initial temperature of the sphere is 3T0 .If the specific heat of the material of the sphere varies as αT3 per unit mass with the temperature of the sphere,where α is a constant,then the time taken for the sphere to cool down to temperature 2T0 will be ( σ Stefan Boltzmann constant)
Mα4πR2σln(32)
Mα4πR2σln(163)
Mα16πR2σln(163)
Mα16πR2σln(32)
The correct Answer is:C
In the given problem, fall in temperature of sphere,
dT=(3T0−2T0)=T0
Tsurr=T0
Tinitial=3T0
Specific heat of the material of the sphere varies as,
C=αT3 per unit mass (α = constant)
Applying formula,
dTdt=−σAMC(T4−T4surr)
dTdt=−σ4πR2Mα(T)3(T4−T4surr)
∫2T03T0T3dT(T4−T4surr)=−σ4πR2Mα∫t0dt
Let (T4−T4surr)=x
Differentiating w.r.t. x
⇒4T3dTdx−0=1
⇒4T3dT=dx
T3dT=dx4
when , T=3T0⇒x=(3T0)4−(T0)4=80T40
when T=2T0⇒x=(2T0)4−(T0)4=15T40
Now , substituting in integral
∫15T4080T40dx4x=−σ4πR2Mα∫t0dt
14[lnx]15T4080T40=−σ4πR2Mα[t]t0
14{ln(15T40)−ln(80T40)}=−σ4πR2Mα(t)
−14ln(1580)=σ4πR2Mα(t)
t=Mα16πR2σln(163)
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBook:MODERN PUBLICATIONChapter:THERMAL PROPERTIES OF MATTERExercise:COMPETITION FILE (OBJECTIVE TYPE QUESTION ((B) MULTIPLE CHOICE QUESTION FROM CPMPETITIVE EXAMINATION ) JEE ADVANCED FOR IIT ENTRANCE )
Explore 9 Videos
- THERMAL PROPERTIES OF MATTERBook:MODERN PUBLICATIONChapter:THERMAL PROPERTIES OF MATTERExercise:COMPETITION FILE (OBJECTIVE TYPE QUESTION ((C ) MULTIPLE CHOICE QUESTION WITH MORE THAN ONE CORRECT ANSWER)
Explore 11 Videos
- THERMAL PROPERTIES OF MATTERBook:MODERN PUBLICATIONChapter:THERMAL PROPERTIES OF MATTERExercise:COMPETITION FILE (OBJECTIVE TYPE QUESTION ((B) MULTIPLE CHOICE QUESTION FROM CPMPETITIVE EXAMINATION ) AIMPMT/NEET AND OTHER STATE BOARDS FOR MEDICAL ENTRANCE)
Explore 18 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBook:MODERN PUBLICATIONChapter:SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONExercise:Chapter Practice Test (for Board Examination)
Explore 16 Videos
- UNITS AND MEASUREMENTBook:MODERN PUBLICATIONChapter:UNITS AND MEASUREMENTExercise:CHAPTER PRACTICE TEST
Explore 15 Videos
Similar Questions
A solid sphere of radius R has a concentric cavity of radius R3 inside it. The sphere is found to just float in water with the highest point of it touching the water surface. Find the specific gravity of the material of the sphere.
View SolutionFind the moment of inertia of a sphere about a tangent to the sphere, while the mass of the sphere is M and the radius of the sphere is R.
View Solution
Knowledge Check
The power P is received by a surface at temperature T0K from a small sphere at temperature
AT(T>>T0) and at a distance 'd'. If both 'T' and 'd' are doubled, then power received by surface will becomeBPC2PD4PA solid sphere of radius R has a concentric cavity of radius 'R/2' inside it. The sphere is found to just float in water with the highest point of it touching the water surface. The specific gravity of the material of the sphere is
A1B78C87D89A uniform solid sphere of radius R has a cavity of radius 1m cut from it if centre of mass of the system lies at the periphery of the cavity then
 A(R2+R+1)(2–R)=1B(R2–R–1)(2–R)=1C(R2–R+1)(2–R)=1D(R2+R–1)(2–R)=1
A(R2+R+1)(2–R)=1B(R2–R–1)(2–R)=1C(R2–R+1)(2–R)=1D(R2+R–1)(2–R)=1
A sphere of material of relative density 8 has a concentric spherical cavity and just sinks in water.If the radius of the sphere is 2cm then the volume of the cavity is
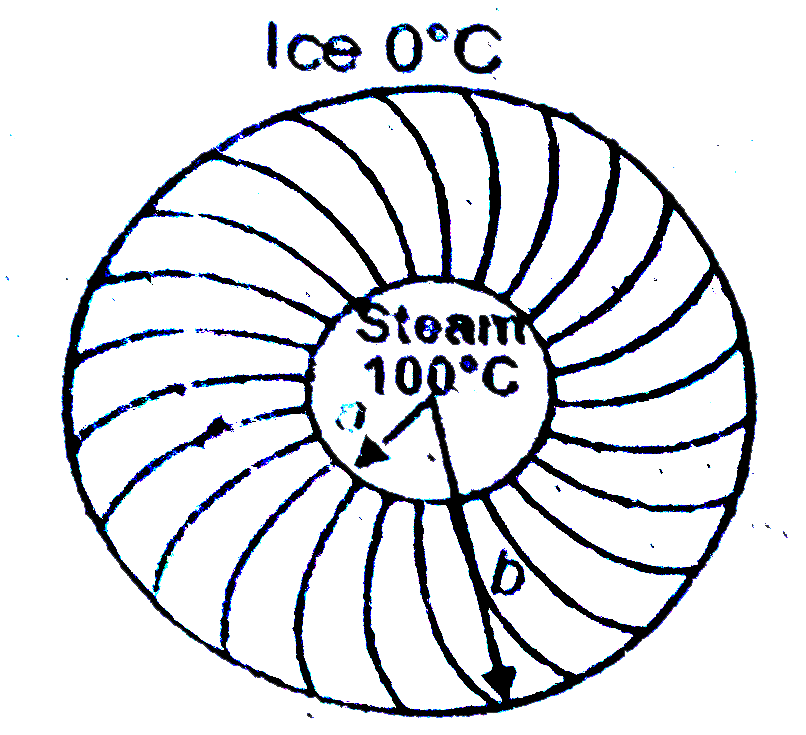
View SolutionA solid sphere of radius b=4cm has a cavity of radius a=2cm. The cavity is filled with steam at 100∘C. The solid sphere is placed inside the ice at 0∘C. Thermal conductivity of material of sphere is 0.5 W/m.∘C. If ther ate of flow of heat through the sphere radially is xπJ/s then find the value of x.
View SolutionThe specific heat of many solids at low temperatures varies with absolute temperature T according to the relation S=AT3, where A is a constant. The heat energy required to raise the temperature of a mass m of such a solid from T = 0 to T = 20 K is
View SolutionThe radius of metal sphere at room temperature T is R and the coefficient of linear expansion of the metal is α. The sphere is heated a little by a temperature T, so that new temperature is T+ΔT. The increase in volume of sphere is approximately
View SolutionA solid sphere and a hollow sphere of the same material and of equal radii are heated to the same temperature.
View Solution
