Calculate the acceleration of block A and B in cases (a),(b), and (c ).
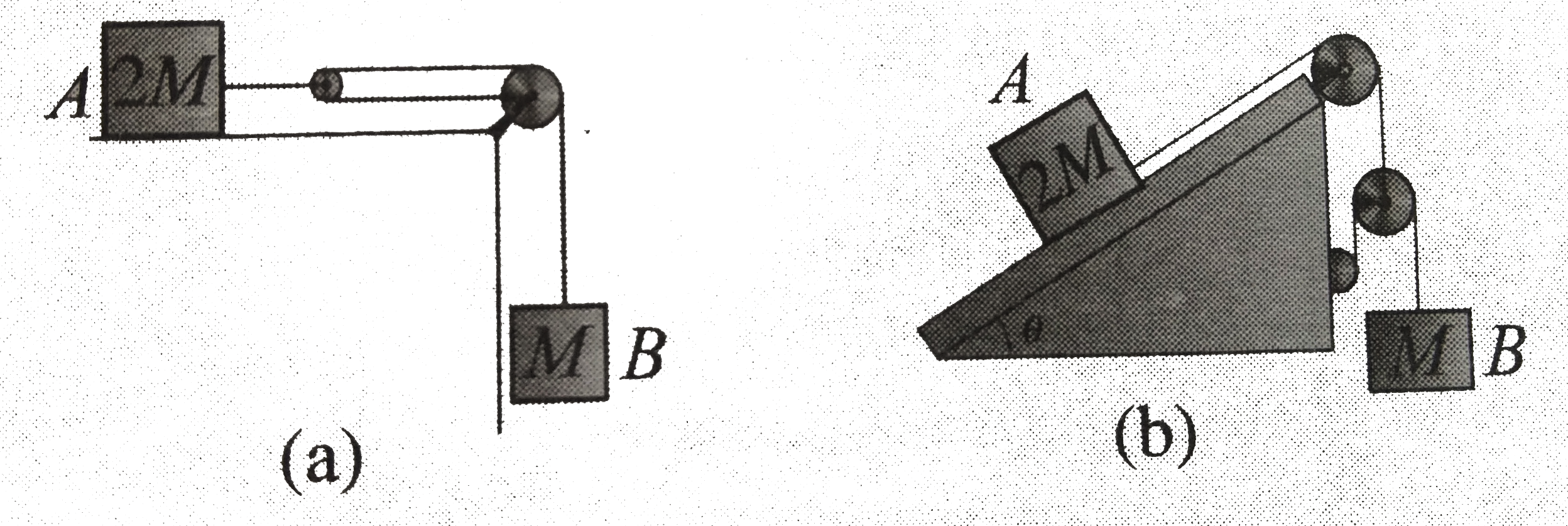


(a) For block 2M,2T=2Ma …(i)
For block M,Mg−t=M2a …(ii)
Solving equation (i) and (ii), we get a=g/3
(b) For block 2M,
2Mgsinθ−T'=2Ma...(i)
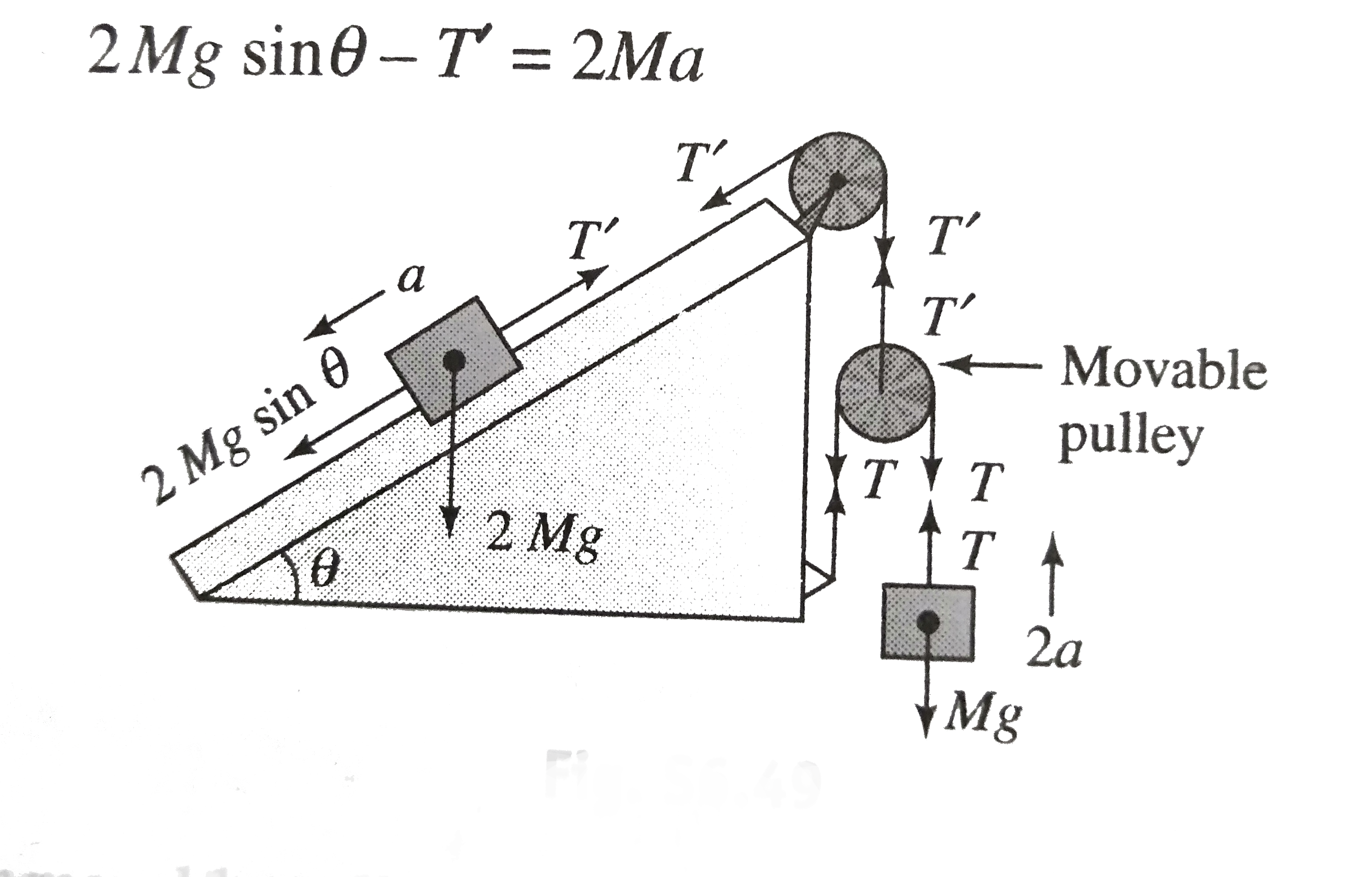
For movable pulley,
T'−2T=0×a⇒T'=2T ...(ii)
For block M,T−Mg=M2a ...(iii)
Solving eq, (i), (ii) , and (iii), we get a=g[sinθ−1]3
This is negative, hence direction of accelerations will be opposite to those shown in fig.
Note: Here we assume that the itblock 2M is moving down the plane. You may assume that it is moving up the plane. The magnitude of the acceleration found will remain the same.
(c) For block 2M,
2Mgsinθ−T=2M(2a) ...(i)

For block M,2T−Mg=Ma ...(ii)
Solving Eqs (i) and (ii), we get a=g[4sinθ−1]9.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.4
13 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Subjective
15 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.2
35 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
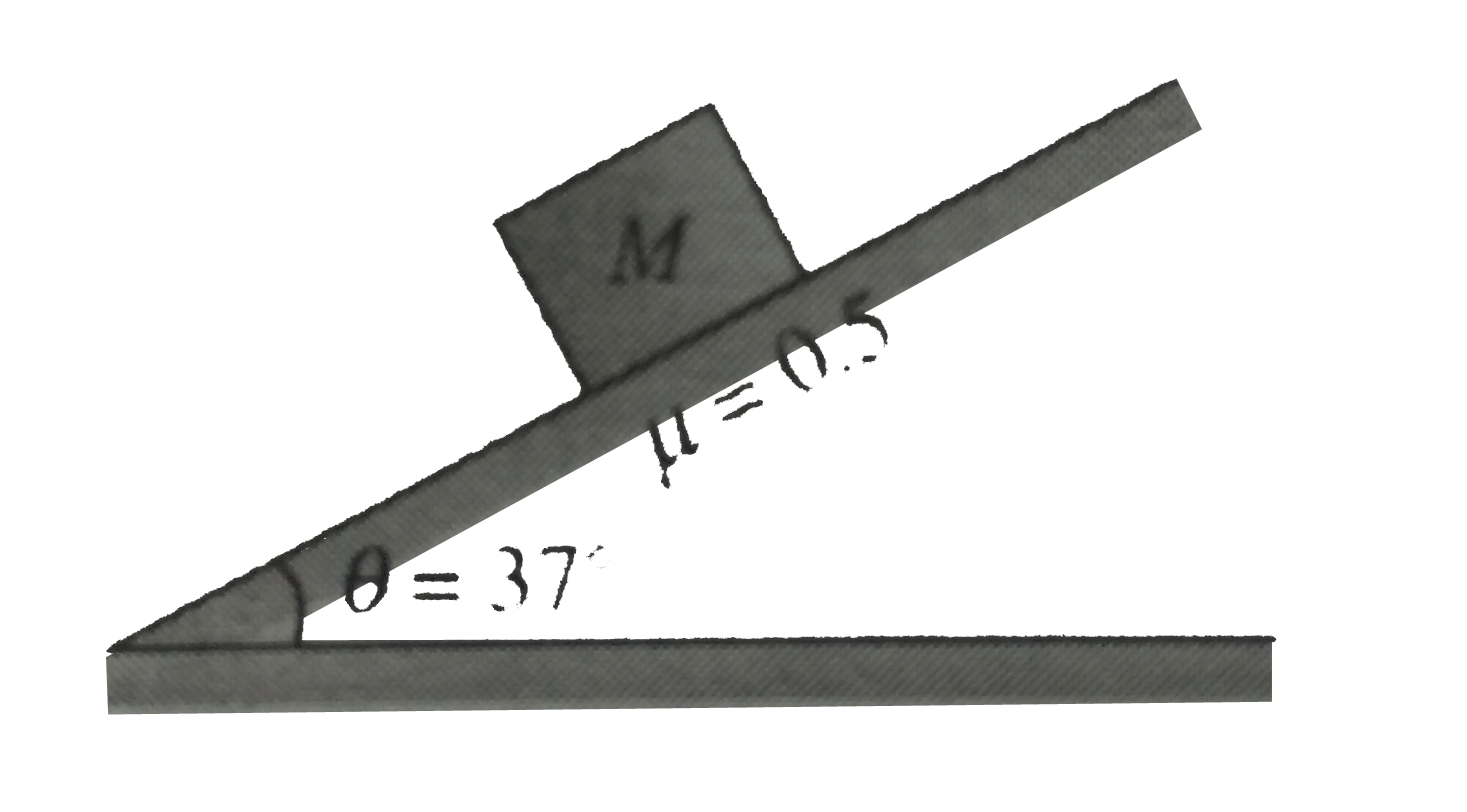
A block of mass M=10kg is placed inclined plane , inclined at angle θ=37∘ with horizontal The coefficient of friction between the block and inclined is μ=0.5
a. Calculate the acceleration of the block when it is released
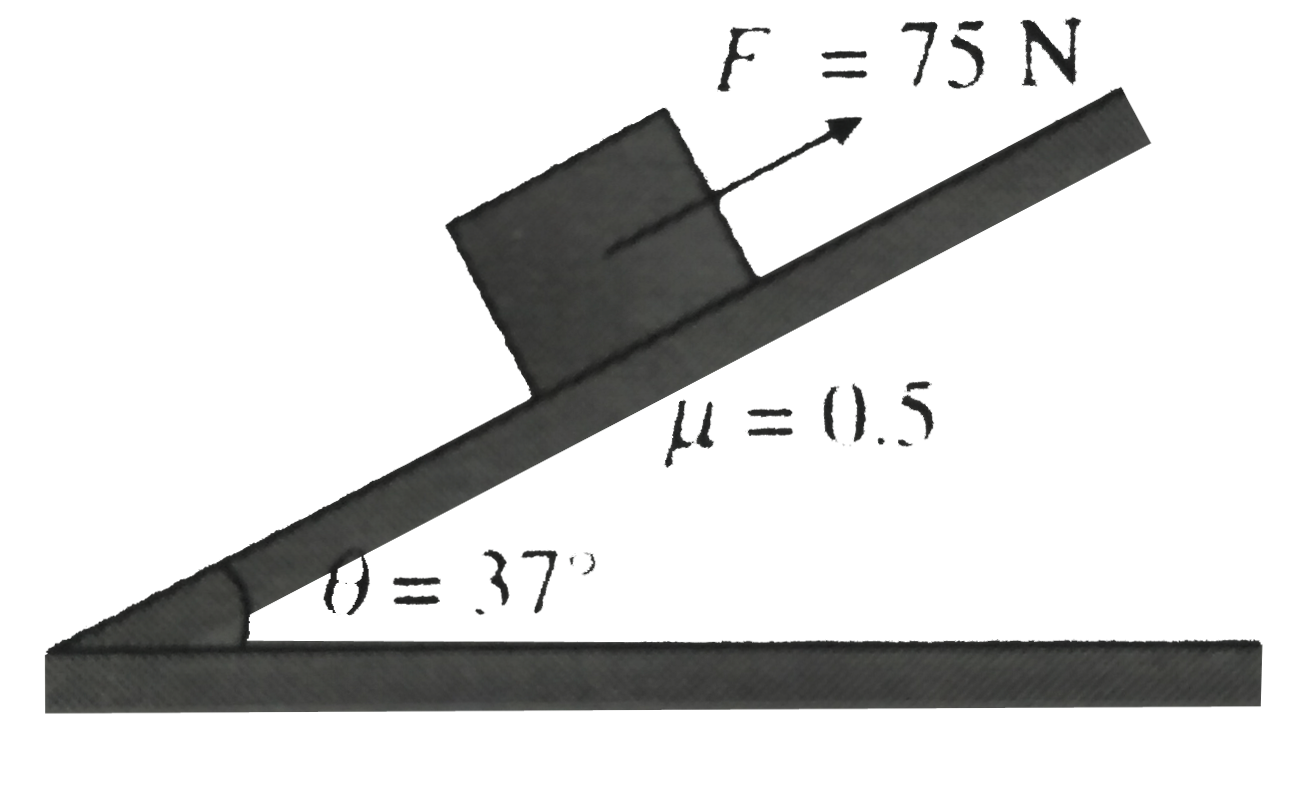
b. Now a force F=75N is appling on block as shown find out the acceleration of the block If the block is initially at rest .
c. In case (b), how much force should be added to 75N force so that block the starts to move up the incline.
d. In case (b), what is the minimum force by which 75N force should be replaced with so that the block does not move?
View SolutionThe coefficient of friction between the blocks is 0.5. Find the acceleration of each block if Fis(a)21N,(b)35 Nand(c)40 N.

View Solution
Knowledge Check
The acceleration of the block A and B are
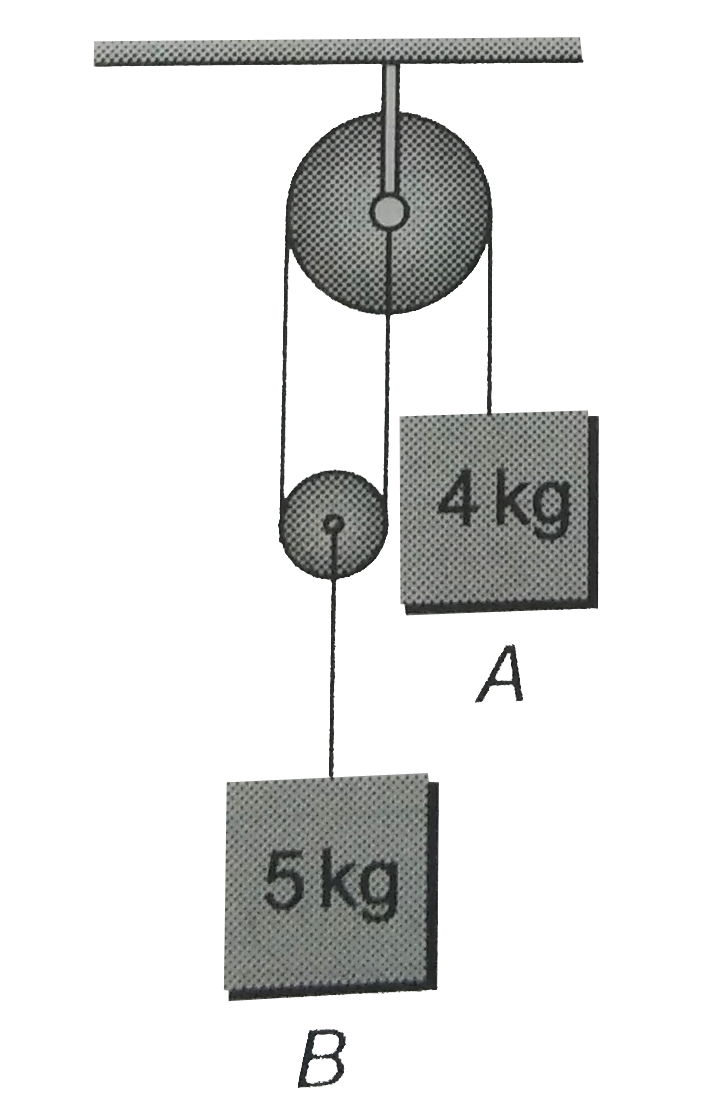 Ag/7 downward, 2g/7 upwardB2g/7 downward, g/7 upwardC3g/7 downward, g/7upwardD4g/7 downward, 2g/7 upward
Ag/7 downward, 2g/7 upwardB2g/7 downward, g/7 upwardC3g/7 downward, g/7upwardD4g/7 downward, 2g/7 upwardThe acceleration of the block A and B are
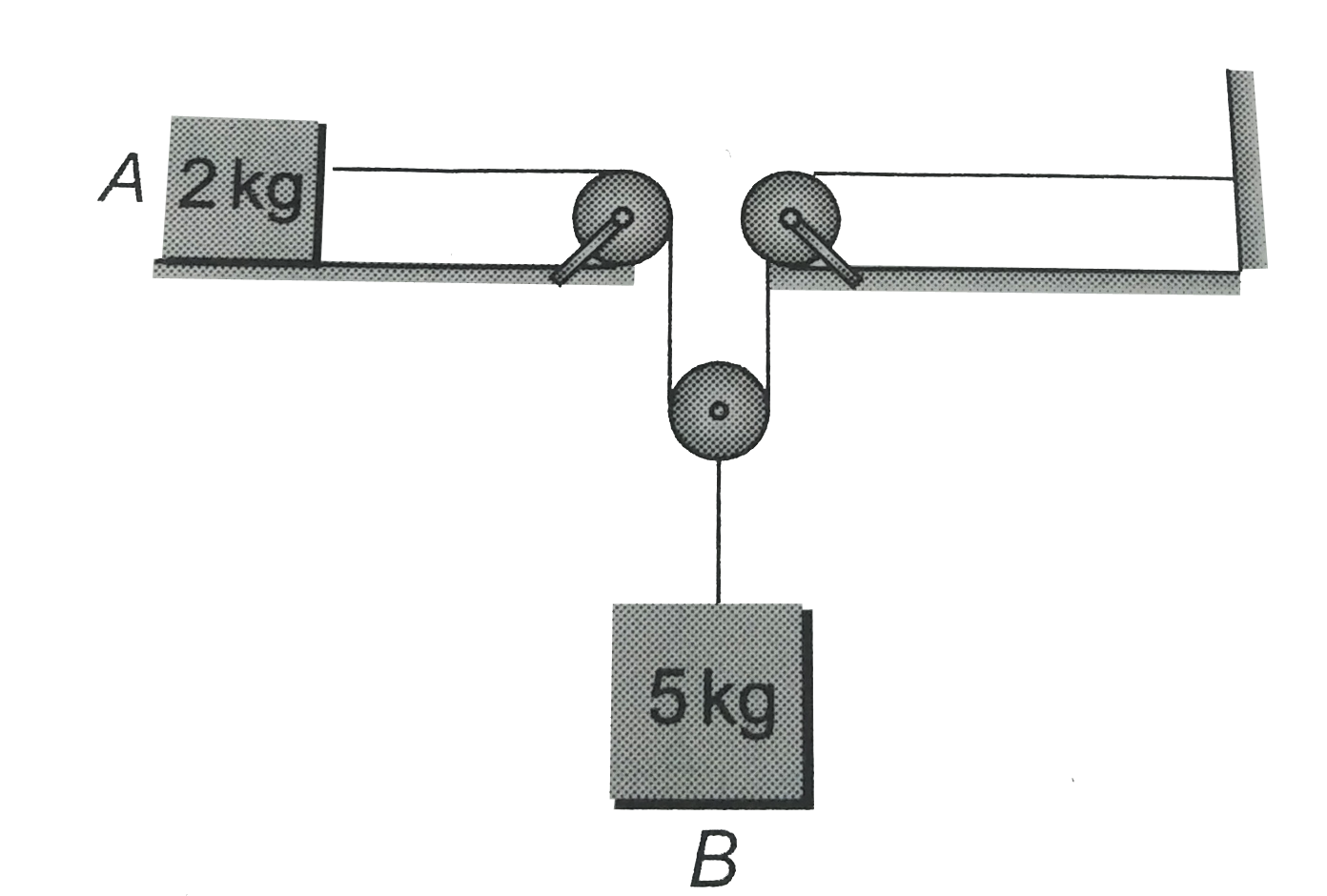 A10g/13 forward, 5g/13 downwardB5g/13 downward, 10g/13 downwardC6g/13 forward, 9g/13 downwardD9g/13 forward, 6g/13 downward
A10g/13 forward, 5g/13 downwardB5g/13 downward, 10g/13 downwardC6g/13 forward, 9g/13 downwardD9g/13 forward, 6g/13 downwardThree blocks A, B, and C of masses 3M,2M, and M are suspended vertically with the help of spring PQ and TU, and a string RS as shown in fig. If the acceleration of blocks A, B and C is a1,a2 and a3 , respectively, then
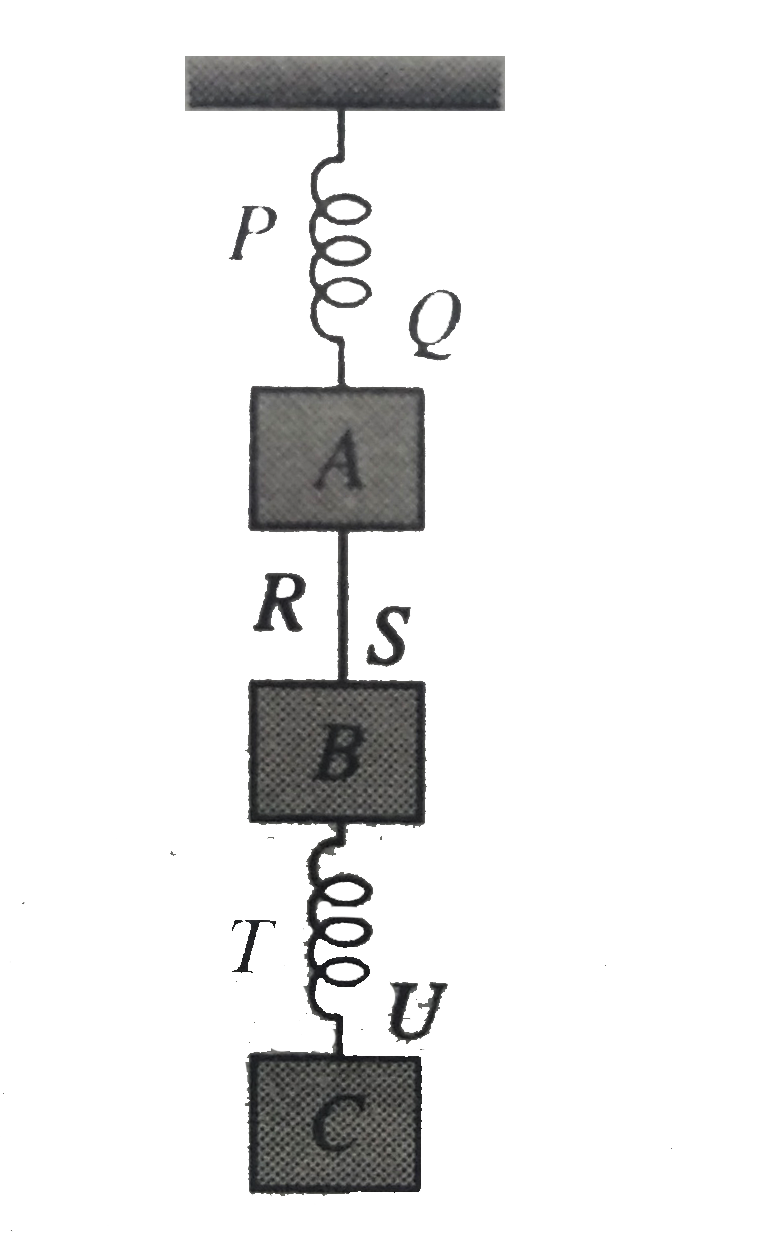
The value of acceleration a1 at the moment string RS is cut isAg, downwardBg, upwardCMore than g, downwardsDZero
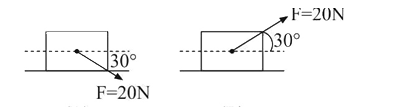
A block of mass 5 kg is (i) pushed in case (A) and (ii) pulled in case (B), by a force F = 20 N, making an angle of 30∘ with the horizontal, as shown in the figures. The coefficient of friction between the block and floor is μ=0.2 . The difference between the accelerations of the block, in case (B) and case (A) will be: (g=10ms−2)
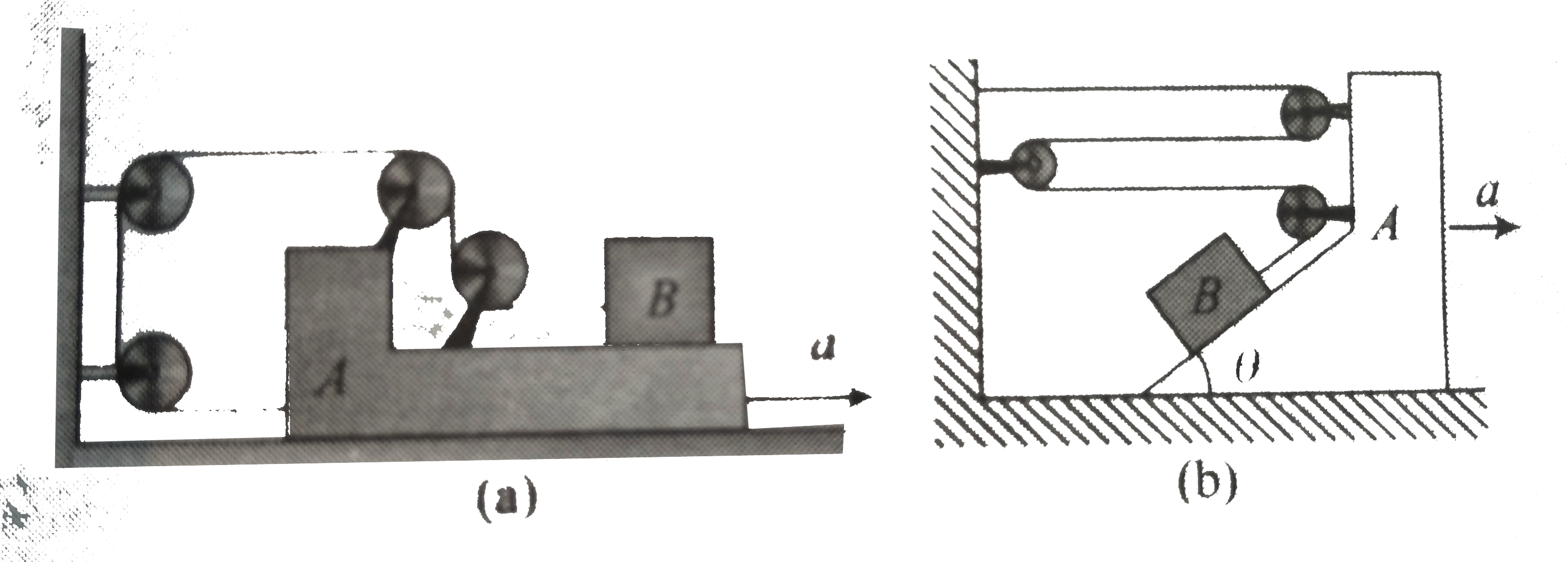
View SolutionFind the acceleration of block B as shown in fig. (a) and (b) relative to block A and relative to ground if block A is moving toward right with acceleration a.
View SolutionThree blocks A, B, and C of masses 3M,2M, and M are suspended vertically with the help of spring PQ and TU, and a string RS as shown in fig. If the acceleration of blocks A, B and C is a1,a2 and a3 , respectively, then
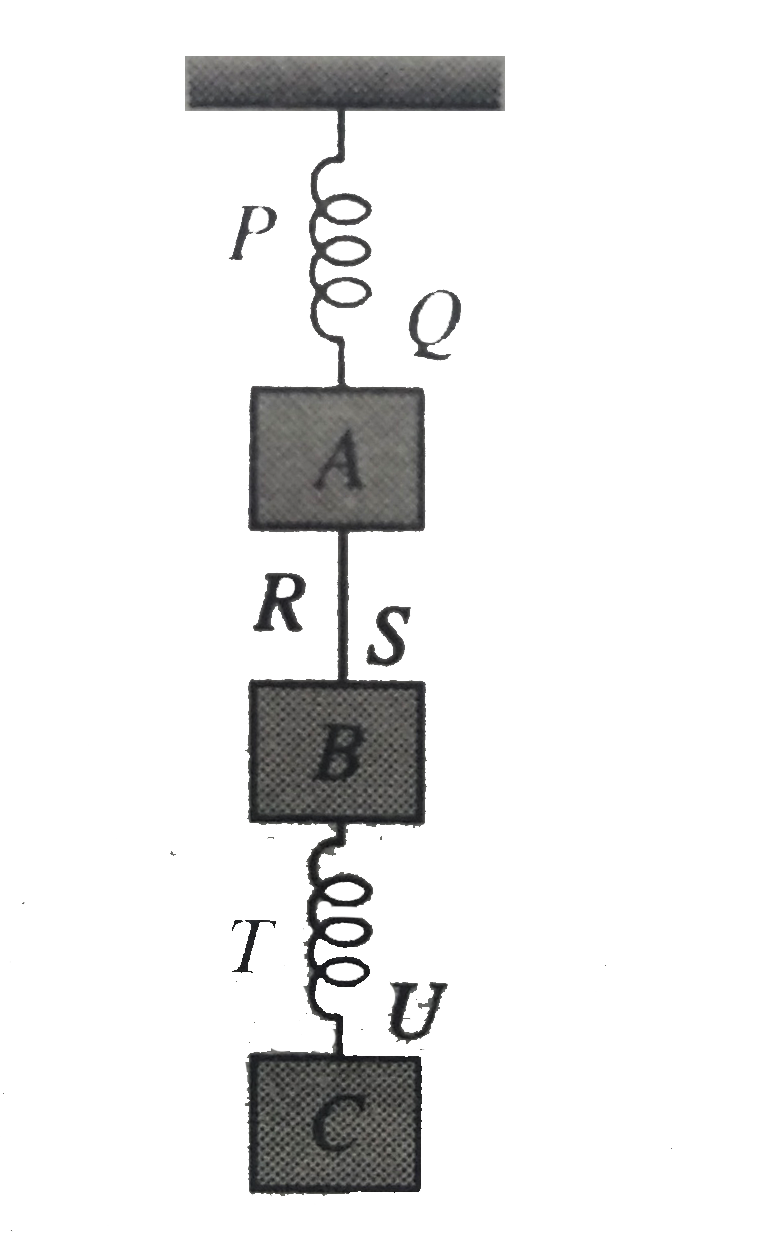
The value of acceleration a2 at the moment spring TU is cut is
View SolutionThree blocks A, B, and C of masses 3M,2M, and M are suspended vertically with the help of spring PQ and TU, and a string RS as shown in fig. If the acceleration of blocks A, B and C is a1,a2 and a3 , respectively, then
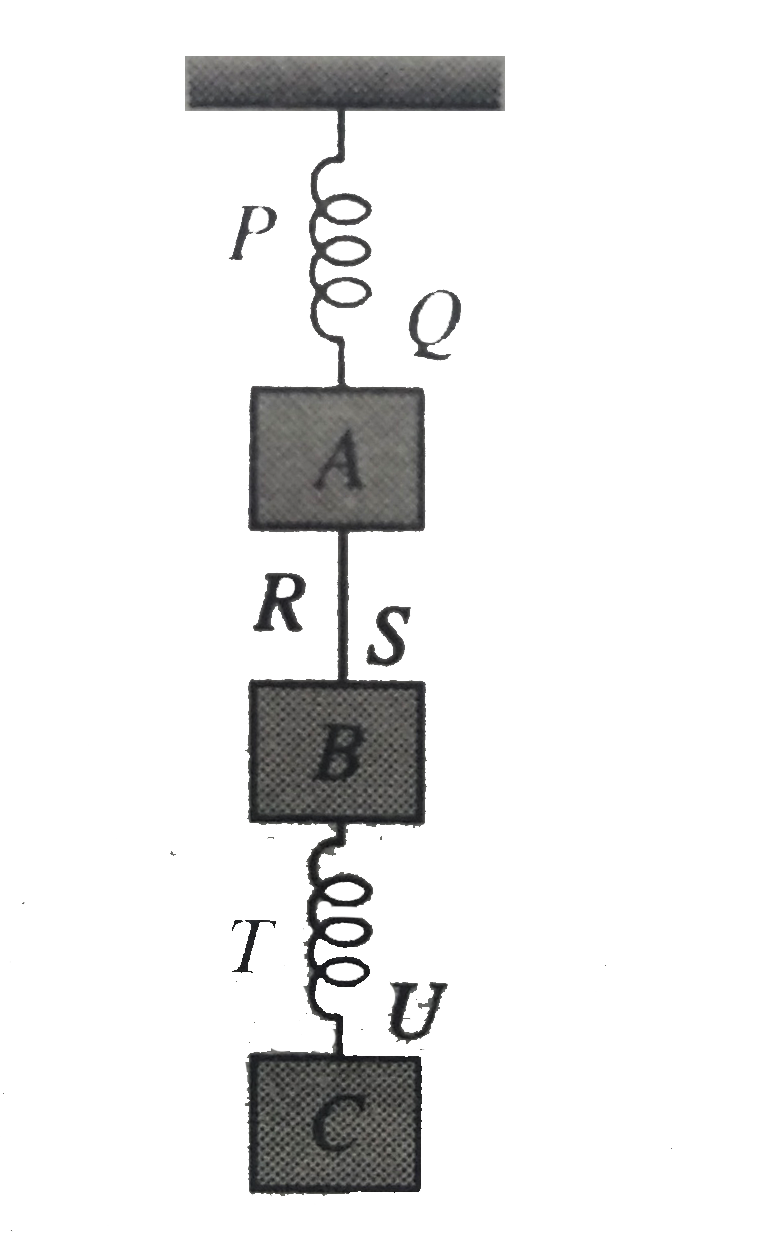
The value of acceleration a3 at the moment spring PQ is cut is
View SolutionIn an arrangement shown in the figure, the acceleration of block A and B are given-

View Solution
