In fig. shown, the speed of the truck is v to the right. Find the speed with which the block is moving up at θ=60∘.

Sum of total change on segment lengths should be zero.

(+x)+(0)+(−y)+(−y)+(0+xcosθ)=0
2y=x+xcosθ
y=x2(1+cos60∘)=x2(1+12)
y=3x4⇒vblock=34vtrcuk.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.4
13 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Subjective
15 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.2
35 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
Find the speed of the block after moving down by x
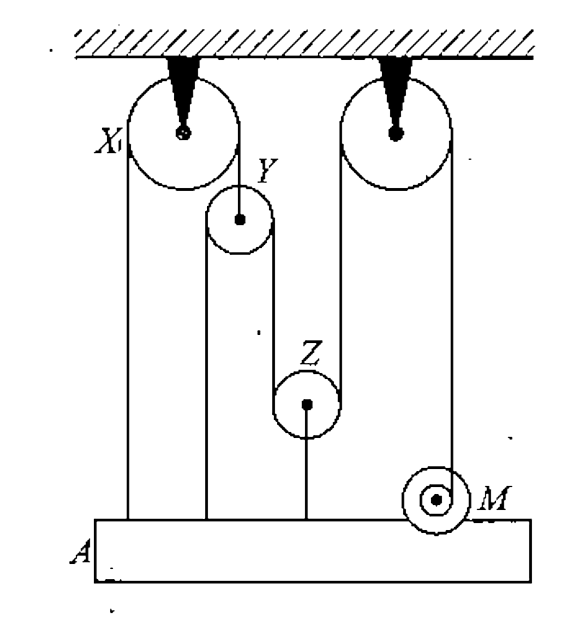
View SolutionConsider the situation of block pulley arrangement shown in figure-1.63. A plank is connected to three strings and an electric motor M is fitted on to it and a string is wound on it according to the arrangement shown in figure. Given that the string is winding on shaft of motor at a speed v. Find the speed with which the plank would be going up.
View Solution
Knowledge Check
truck is moving with a speed of 15 m/s. A car chasing this truck with a speed of 10 m/s fires a bullet at truck with muzzle velocity 90 km/h. The speed with which the bullet hit the truck is
A15 m/sB20 m/sC25 m/sD30 m/sThree blocks A, B and C each of mass m are placed on a surface as shown in the figure. Blocks B and C are initialyy at rest. Block A is moving to the right with speed v. It collides with block B and stricks to it. The A-B combination collides elastically with block C. Which of the following statement is (are) true about the velocity, of block B and C.
 AVelocity of the block C after collision is 2/3v towards rightBVelocity of the A−B combination after collision is v3 towards leftCVelocity of the A−B combination after collision is 23v towards leftDVelocity of the block C after collision is v3 towards right.
AVelocity of the block C after collision is 2/3v towards rightBVelocity of the A−B combination after collision is v3 towards leftCVelocity of the A−B combination after collision is 23v towards leftDVelocity of the block C after collision is v3 towards right.A truck has to move to a dimetrically opposite point on a circular track which surrounds a field. The speed of the truck along the track is 2v0. While that in the field is v0. The driver plans to move along an arc of a circle and then along a straight line as shown.
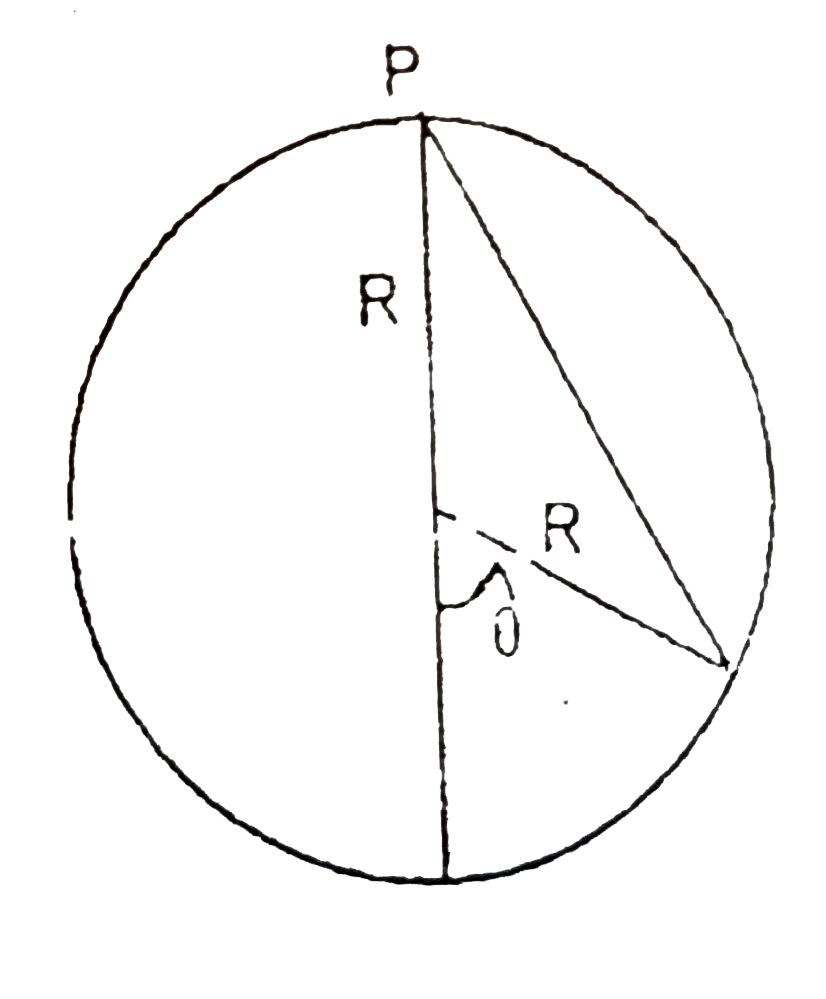 ATo reach P in shortest time, Q must be equal to 60∘BThe minimum time required to rach P is Rv0[π6+√3]CThe distance travelled to reach P in shortest time is R[π3+√3].DThe angle θ will not depend on the value of v0.
ATo reach P in shortest time, Q must be equal to 60∘BThe minimum time required to rach P is Rv0[π6+√3]CThe distance travelled to reach P in shortest time is R[π3+√3].DThe angle θ will not depend on the value of v0.
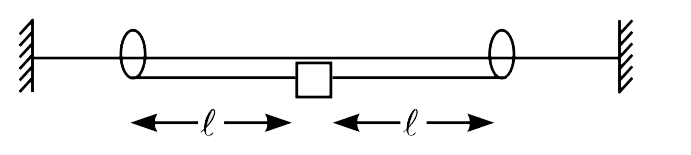
Two small rings each of mass ‘m’ are connected to a block of same mass ‘m’ through inextensible light strings. Rings are constrained to move along a smooth horizontal rod. Initially system is held at rest (as shown in figure) with the strings just taut. Length of each string is ‘l’. The system is released from the position shown. Find the speed of the block (v) and speed of the rings (u) when the strings make an angle of theta=60^(@)withvertical.(Takeg = 10 m//s^2)
View SolutionTwo particles of a mass 2m and m are tied with an inextensible string the particle of mass m is given a speed V as shown in the figure. Find the speed with which the particle of mass 2m starts moving.

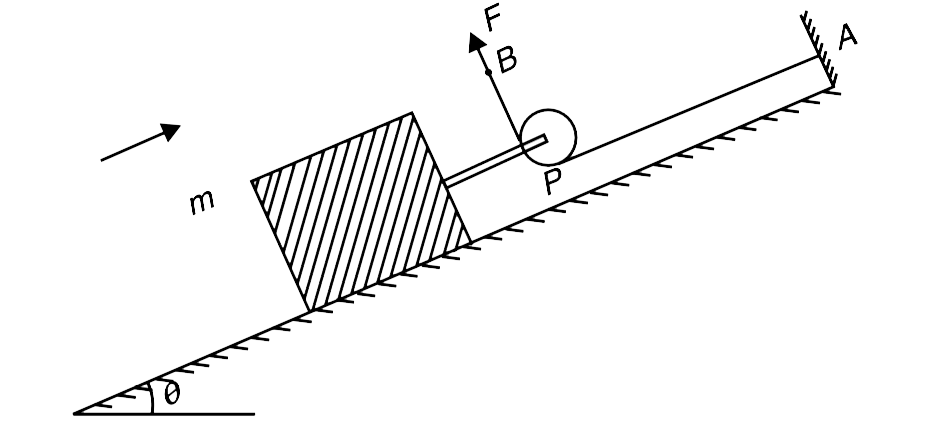
View SolutionIn the fig. shown, the pulley and string are mass less and the incline is frictionless. The segment AP of the string is parallel to the incline and the segment PB is perpendicular to the incline. End of the string is pulled with a constant force F.
(a) If the block is moving up the incline with acceleration while being in contact with the incline , then angle θ must be less than θ0. Find θ0
If θ=θ02 find the maximum acceleration with which the block can move up the plane without losing contact with the incline.
View SolutionA boy is standing on a truck moving with a constant velocity of 15m/s on the horizontal road. The boy throws a ball in such a way that it returns to the truck after the truck has moved 60m. Find the speed and the angle of projection (a) as seen by the truck and (b) as seen from the road.
View SolutionFind out the distance travelled by the block as shown in figure. If the initial speed of the block is v and μ is the friction coefficient between the surface of block and ground.
View Solution
