Calculate the impact parameter of 5.0 MeV α-particle scattered by 10∘ when it approaches a gold nuclcus (Z = 79).
To calculate the impact parameter b of a 5.0 MeV alpha particle scattered by 10 degrees when it approaches a gold nucleus (Z = 79), we can use the formula:
b=Ze2cot(θ/2)4πϵ0⋅12mv2
Step 1: Identify the given values
- Energy of the alpha particle, E=5.0MeV
- Charge of the alpha particle, Z=2 (since an alpha particle consists of 2 protons)
- Charge of the gold nucleus, Z=79
- Scattering angle, θ=10∘
Step 2: Convert energy to joules
1 MeV = 1.6×10−13J
E=5.0MeV=5.0×1.6×10−13J=8.0×10−13J
Step 3: Calculate 12mv2
The kinetic energy KE is given by:
KE=12mv2=E=8.0×10−13J
Step 4: Calculate cot(θ/2)
Convert the angle to radians:
θ/2=5∘
Using the cotangent function:
cot(5∘)≈11.43
Step 5: Substitute values into the impact parameter formula
Using e=1.6×10−19C and ϵ0=8.85×10−12C2/N m2:
b=79⋅(1.6×10−19)2⋅cot(5∘)4π(8.85×10−12)⋅(8.0×10−13)
Step 6: Calculate b
1. Calculate (1.6×10−19)2:
(1.6×10−19)2=2.56×10−38
2. Substitute into the equation:
b=79⋅2.56×10−38⋅11.434π(8.85×10−12)⋅(8.0×10−13)
3. Calculate the denominator:
4π(8.85×10−12)⋅(8.0×10−13)≈8.88×10−24
4. Finally, calculate b:
b≈79⋅2.56×10−38⋅11.438.88×10−24≈2.6×10−30m
Final Answer
The impact parameter b is approximately 2.6×10−30m.
---
- Energy of the alpha particle, E=5.0MeV
- Charge of the alpha particle, Z=2 (since an alpha particle consists of 2 protons)
- Charge of the gold nucleus, Z=79
- Scattering angle, θ=10∘
1 MeV = 1.6×10−13J
The kinetic energy KE is given by:
Convert the angle to radians:
Using e=1.6×10−19C and ϵ0=8.85×10−12C2/N m2:
1. Calculate (1.6×10−19)2:
(1.6×10−19)2=2.56×10−38
b=79⋅2.56×10−38⋅11.434π(8.85×10−12)⋅(8.0×10−13)
4π(8.85×10−12)⋅(8.0×10−13)≈8.88×10−24
b≈79⋅2.56×10−38⋅11.438.88×10−24≈2.6×10−30m
The impact parameter b is approximately 2.6×10−30m.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - EXERCISE - I20videos
ATOMS
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - EXERCISE - II19videos
ATOMS
- BOOK - AAKASH SERIESCHAPTER - ATOMSEXERCISE - EXERCISE -III25videos
ATOMS
- BOOK - AAKASH SERIESCHAPTER - APPENDICES (REVISION EXERCISE)EXERCISE - LAW OF MOTION128videos
APPENDICES (REVISION EXERCISE)
- BOOK - AAKASH SERIESCHAPTER - CAPACITORS EXERCISE - PRACTICE SHEET (ADVANCED) (Integer Type Questions)2videos
CAPACITORS
Similar Questions
Knowledge Check
- Question 1 - Select One
The impact parameter at which the scattering angle is 900,z=79 and initial energy 10MeV is
A1.137*10^-14B1.137*10^-16C2.24*10^-17Dzero
Calculate the mass of an alpha-particle.Its binding energy is 28.2 meV.
View SolutionCalculate distance of closet approach by an α-particle of KE=2.5MeV being scattered by gold nucleus (Z=79).
View SolutionWhat should be the effect of impact parameter upon the deviation of α-particle.
View SolutionWhen a silver foil (Z = 47) was used in an α ray scattering experiment , the number of α-particles scattered at 30∘ was found to be 200 per minute. If the silver foil is replaced by alumninium (z = 13) foil of same thickness, the number of α-particles scattered per minute at 30∘ is nearly equal to
View SolutionThickness of the foil of gold used in α-particle scattering experiment is
View SolutionThe distance of the closest approach of an alpha particle fired at a nucleus with kinetic of an alpha particle fired at a nucleus with kinetic energy K is r0. The distance of the closest approach when the α particle is fired at the same nucleus with kinetic energy 2K will be
 View Solution
View SolutionRutherford model: The approximate size of the nucleus can be calculated by using energy conservation theorem in Rutherford's α-scattering experiment. If an α-particle is projected from infinity with speed v towards the nucleus having Z protons, then the α-particle which is reflected back or which is deflected by 180∘ must have approached closest to the nucleus .It can be approximated that α particle collides with the nucleus and gets back. Now if we apply the energy conservation equation at initial point and collision point then:
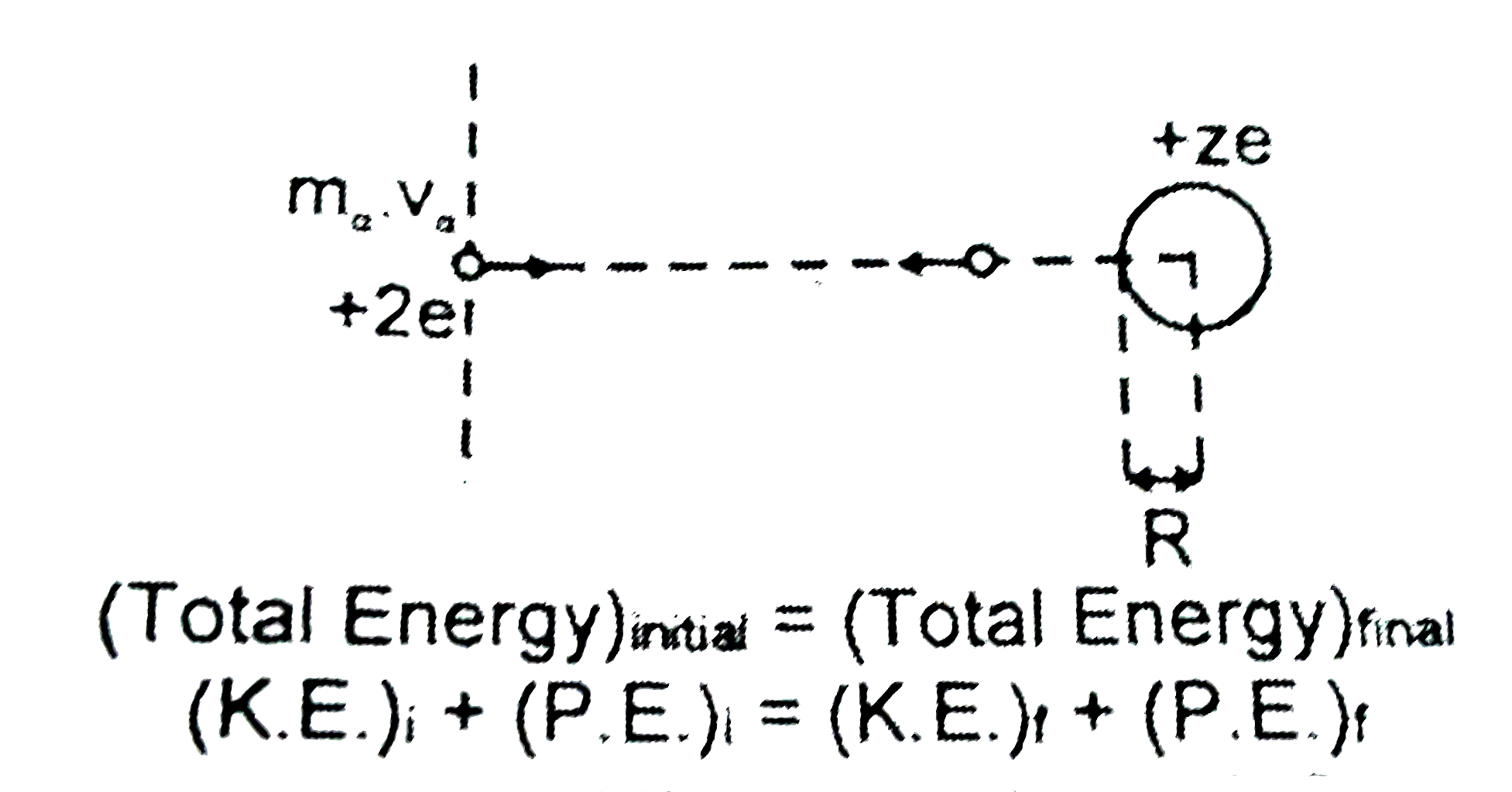
(P.E.)i=0, since P.E. of two charge system separated by infinite distance is zero. Finally the particle stops and then starts coming back.
12mαv2α+0=0+Kq1q2R⇒12mαv2α=K2e×ZeR⇒R=4KZe2mαv2α
Thus the radius of nucleus can be calculated using above equation. The nucleus is so small a particle that we can't define a sharp boundary for it
An α-particle with initial speed v0 is projected from infinity and it approaches up to r0 distance from a nuclie. Then, the initial speed of α-particle, which approaches upto 2r0 distance from the nucleus is :View Solution

