Calculate the moment of inertia of a cylinder of length 2 m, radius 5 cm and density 8×103kg/m3 about (i) the axis of the cylinder and (ii) and axis passing through the centre and perpendicular to its length.
The correct Answer is:(i) 0.157kgm2, (ii) 41.94kgm2
To solve the problem of calculating the moment of inertia of a cylinder, we will follow these steps:
Given Data:
- Length of the cylinder, L=2m
- Radius of the cylinder, r=5cm=0.05m
- Density of the cylinder, ρ=8×103kg/m3
Step 1: Calculate the Volume of the Cylinder
The volume V of a cylinder is given by the formula:
V=πr2h
Substituting the values:
V=π(0.05)2(2)=π(0.0025)(2)=0.005πm3
Step 2: Calculate the Mass of the Cylinder
The mass m can be calculated using the formula:
m=ρV
Substituting the values:
m=8×103×0.005π≈125.6kg
Step 3: Calculate the Moment of Inertia about the Axis of the Cylinder (I1)
The moment of inertia I1 of a solid cylinder about its own axis is given by:
I1=12mr2
Substituting the values:
I1=12×125.6×(0.05)2=12×125.6×0.0025
Calculating:
I1=12×125.6×0.0025≈0.157kg m2
Step 4: Calculate the Moment of Inertia about an Axis Perpendicular to its Length (I2)
The moment of inertia I2 about an axis passing through the center and perpendicular to its length is given by:
I2=112mL2+14mr2
Substituting the values:
I2=112×125.6×(2)2+14×125.6×(0.05)2
Calculating each term:
I2=112×125.6×4+14×125.6×0.0025
I2=112×502.4+14×0.314
Calculating:
I2=41.8667+0.0785≈41.94kg m2
Final Results:
1. Moment of inertia about the axis of the cylinder, I1≈0.157kg m2
2. Moment of inertia about the axis perpendicular to its length, I2≈41.94kg m2
- Length of the cylinder, L=2m
- Radius of the cylinder, r=5cm=0.05m
- Density of the cylinder, ρ=8×103kg/m3
The volume V of a cylinder is given by the formula:
V=πr2h
Substituting the values:
V=π(0.05)2(2)=π(0.0025)(2)=0.005πm3
The mass m can be calculated using the formula:
m=ρV
Substituting the values:
m=8×103×0.005π≈125.6kg
The moment of inertia I1 of a solid cylinder about its own axis is given by:
I1=12mr2
Substituting the values:
I1=12×125.6×(0.05)2=12×125.6×0.0025
Calculating:
I1=12×125.6×0.0025≈0.157kg m2
The moment of inertia I2 about an axis passing through the center and perpendicular to its length is given by:
I2=112mL2+14mr2
Substituting the values:
I2=112×125.6×(2)2+14×125.6×(0.05)2
Calculating each term:
I2=112×125.6×4+14×125.6×0.0025
I2=112×502.4+14×0.314
Calculating:
I2=41.8667+0.0785≈41.94kg m2
1. Moment of inertia about the axis of the cylinder, I1≈0.157kg m2
2. Moment of inertia about the axis perpendicular to its length, I2≈41.94kg m2
Topper's Solved these Questions
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ANGULAR MOMENTUM - CONSERVATION)
14 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM CENTRE OF MASS)
8 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
50 Videos
Similar Questions
Knowledge Check
The radius of gyration of an uniform rod of length l about an axis passing through one of its ends and perpendicular to its length is.
Al√2Bl3Cl√3Dl2
Moment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionDerive an expression per the M.I. of a rectangular rod about an axis passing through its centre of mass and perpendicular to its length.
View SolutionThe mass of a unifprm rod is 0.4 kg and its length is 2 m. .Calculate the radius of gyration of the rod about an axis passing through its centre of mass and perpendicular to its length ?
View Solutionfind the radius of gyration of a rod of mass m and length 2l about an axis passing through one of its ends and perpendicular to its length.
View SolutionLet the moment of inertia of a hollow cylinder of length 30 cm (inner radius 10 cm and outer radius 20 cm ), about its axis be I. the radius of a thin cylinder of the same about its axis is also I is :
View SolutionFind the moment of inertia of a solid cylinder of mass M and radius R about a line parallel to the axis of the cylinder and on the surface of the cylinder.
View SolutionMatch the Moment of inertia of a solid cylinder of mass m, radius R and length l along various axis as shown in figure.
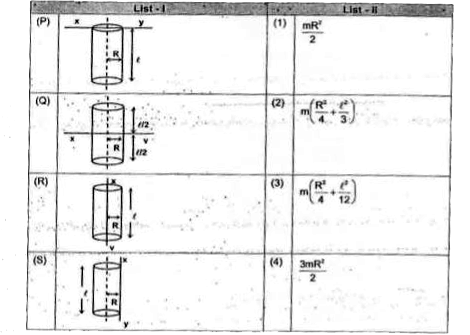
View Solution
