Calculate the self-gravitational potential energy of matter forming a. a thin uniform shell of mass M and radius R, b. a uniform sphere of mass m and radius R.
To solve the problem of calculating the self-gravitational potential energy for both a thin uniform shell and a uniform sphere, we will follow these steps:
Part A: Self-Gravitational Potential Energy of a Thin Uniform Shell
1. Understanding the Shell: We consider a thin spherical shell of mass M and radius R.
2. Gravitational Potential Due to the Shell: The gravitational potential V at the surface of the shell (or inside it) is given by:
V=−GMR
where G is the gravitational constant.
3. Work Done to Assemble the Shell: The work done dW to bring an infinitesimal mass dm from infinity to the shell is:
dW=V⋅dm=−GMR⋅dm
4. Integrating to Find Total Work: To find the total work done to assemble the entire mass M, we integrate from 0 to M:
W=∫M0−GMRdm=−GMR⋅M=−GM2R
5. Final Result for Part A: Thus, the self-gravitational potential energy U of the thin uniform shell is:
U=−GM2R
Part B: Self-Gravitational Potential Energy of a Uniform Sphere
1. Understanding the Sphere: We consider a uniform solid sphere of mass m and radius R.
2. Density of the Sphere: The density ρ of the sphere is given by:
ρ=m43πR3
3. Elemental Mass: We take an infinitesimal spherical shell of thickness dr at a distance r from the center. The volume of this shell is:
dV=4πr2dr
Thus, the elemental mass dm is:
dm=ρ⋅dV=ρ⋅4πr2dr=m43πR3⋅4πr2dr=3mR3r2dr
4. Gravitational Potential for Elemental Mass: The gravitational potential V at a distance r from the center of the sphere is:
V=−Gm(r)r
where m(r) is the mass enclosed within radius r:
m(r)=43πr3⋅ρ=43πr3⋅m43πR3=mr3R3
Hence, the potential becomes:
V=−G⋅m⋅r3R3r=−Gmr2R3
5. Work Done to Assemble the Sphere: The work done dW to bring dm from infinity to the sphere is:
dW=V⋅dm=−Gmr2R3⋅3mR3r2dr=−3Gm2R3r4dr
6. Integrating to Find Total Work: Now, we integrate from 0 to R:
W=∫R0−3Gm2R3r4dr=−3Gm2R3⋅(r55)R0=−3Gm2R3⋅R55=−3Gm25R
7. Final Result for Part B: Thus, the self-gravitational potential energy U of the uniform sphere is:
U=−3Gm25R
Summary of Results
- For a thin uniform shell:
U=−GM2R
- For a uniform sphere:
U=−3Gm25R
V=−GMR
where G is the gravitational constant.
dW=V⋅dm=−GMR⋅dm
W=∫M0−GMRdm=−GMR⋅M=−GM2R
U=−GM2R
ρ=m43πR3
dV=4πr2dr
Thus, the elemental mass dm is:
dm=ρ⋅dV=ρ⋅4πr2dr=m43πR3⋅4πr2dr=3mR3r2dr
V=−Gm(r)r
where m(r) is the mass enclosed within radius r:
m(r)=43πr3⋅ρ=43πr3⋅m43πR3=mr3R3
Hence, the potential becomes:
V=−G⋅m⋅r3R3r=−Gmr2R3
dW=V⋅dm=−Gmr2R3⋅3mR3r2dr=−3Gm2R3r4dr
W=∫R0−3Gm2R3r4dr=−3Gm2R3⋅(r55)R0=−3Gm2R3⋅R55=−3Gm25R
U=−3Gm25R
- For a thin uniform shell:
U=−GM2R
- For a uniform sphere:
U=−3Gm25R
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 6.113videos
GRAVITATION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise 6.215videos
GRAVITATION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE1videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer9videos
KINEMATICS-1
Similar Questions
Figure shows a hemispherical shell of mass M and radius R and a concentric shell of mass m and radius 3R/4.
 View Solution
View SolutionFor a uniform ring of mass M and radius R at its centre
View SolutionA thin spherical shell of mass M and radius R has a small hole. A particle of mass m released at its mouth. Then
View SolutionCalculate the gravitational field intensity and potential at the centre of the base of a solid hemisphere of mass m, radius R
View SolutionFind the moment of inertia of a uniform ring of mass M and radius R about a diameter.
View SolutionCalculate the gravitational field intensity at the centre of the base of a hollow hemisphere of mass M and radius R. (Assume the base of hemisphere to be open)
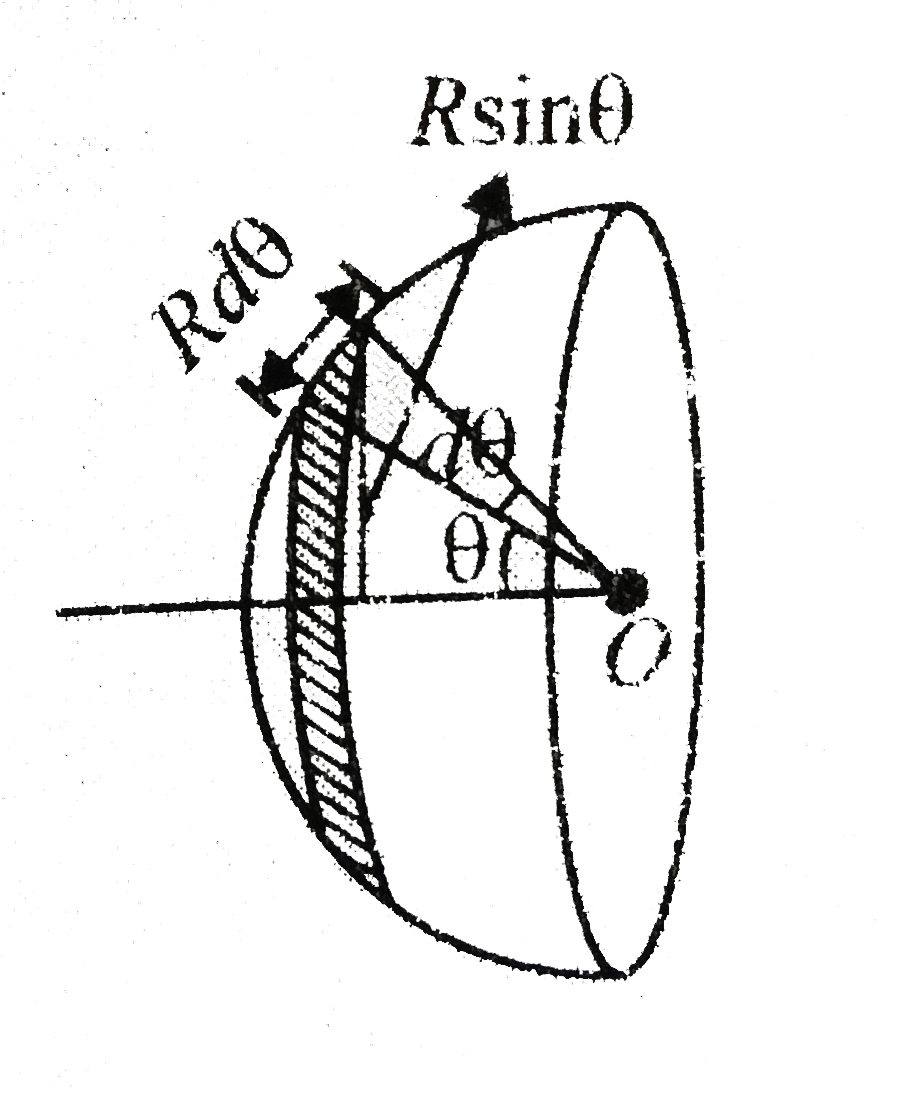 View Solution
View SolutionFind the moment of inertia of a uniform sphere of mass m and radius R about a tangent if the spheres (1) solid (ii) hollow?
View SolutionThe gravitational field due to an uniform solid sphere of mass M and radius a at the centre of the sphere is
View SolutionGravitational potential energy of body of mass m at a height of h above the surface of earth (M = mass of earth , R = radius of earth) is
View SolutionFind the gravitational potential due to a spherical shell of mass M and radius R at r<R and r>R, where r is the distance from the centre of the shell.
View Solution

