Can a gravitational field be added vectorially to an electric field to get a total field?
To determine whether a gravitational field can be added vectorially to an electric field to obtain a total field, we can analyze the properties of both fields step by step.
Step 1: Define the Gravitational Field
The gravitational field (denoted as g) is defined as the force experienced by a unit mass in a gravitational field. Mathematically, it can be expressed as:
g=Fm
where F is the gravitational force and m is the mass. The unit of the gravitational field is Newton per kilogram (N/kg).
Step 2: Define the Electric Field
The electric field (denoted as E) is defined as the force experienced by a unit charge in an electric field. It can be expressed as:
E=FQ
where F is the electric force and Q is the charge. The unit of the electric field is Newton per coulomb (N/C).
Step 3: Compare the Units
Now, we need to compare the units of both fields:
- Gravitational field: N/kg
- Electric field: N/C
Step 4: Analyze the Dimensions
The dimensions of the gravitational field and electric field are different. The gravitational field is related to mass, while the electric field is related to charge. Since they have different physical bases (mass vs. charge), they cannot be directly compared or combined.
Step 5: Conclusion
Since the gravitational field and electric field have different units and dimensions, they cannot be added vectorially. Therefore, we conclude that a gravitational field cannot be added to an electric field to obtain a total field.
Final Answer
No, a gravitational field cannot be added vectorially to an electric field to get a total field because they have different units and dimensions.
---
The gravitational field (denoted as g) is defined as the force experienced by a unit mass in a gravitational field. Mathematically, it can be expressed as:
g=Fm
where F is the gravitational force and m is the mass. The unit of the gravitational field is Newton per kilogram (N/kg).
The electric field (denoted as E) is defined as the force experienced by a unit charge in an electric field. It can be expressed as:
E=FQ
where F is the electric force and Q is the charge. The unit of the electric field is Newton per coulomb (N/C).
Now, we need to compare the units of both fields:
- Gravitational field: N/kg
- Electric field: N/C
The dimensions of the gravitational field and electric field are different. The gravitational field is related to mass, while the electric field is related to charge. Since they have different physical bases (mass vs. charge), they cannot be directly compared or combined.
Since the gravitational field and electric field have different units and dimensions, they cannot be added vectorially. Therefore, we conclude that a gravitational field cannot be added to an electric field to obtain a total field.
No, a gravitational field cannot be added vectorially to an electric field to get a total field because they have different units and dimensions.
Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 1
9 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 2
7 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises
75 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Short answer
6 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Questions for short answer
9 Videos
Similar Questions
Knowledge Check
The Electric field at a point is
Aalways continuousBcontinuous if there is no charge at that pointCdiscontinuous if there is a charge at that pointDboth (b) and ( c) are correct
Gravitational field due to a solid sphere
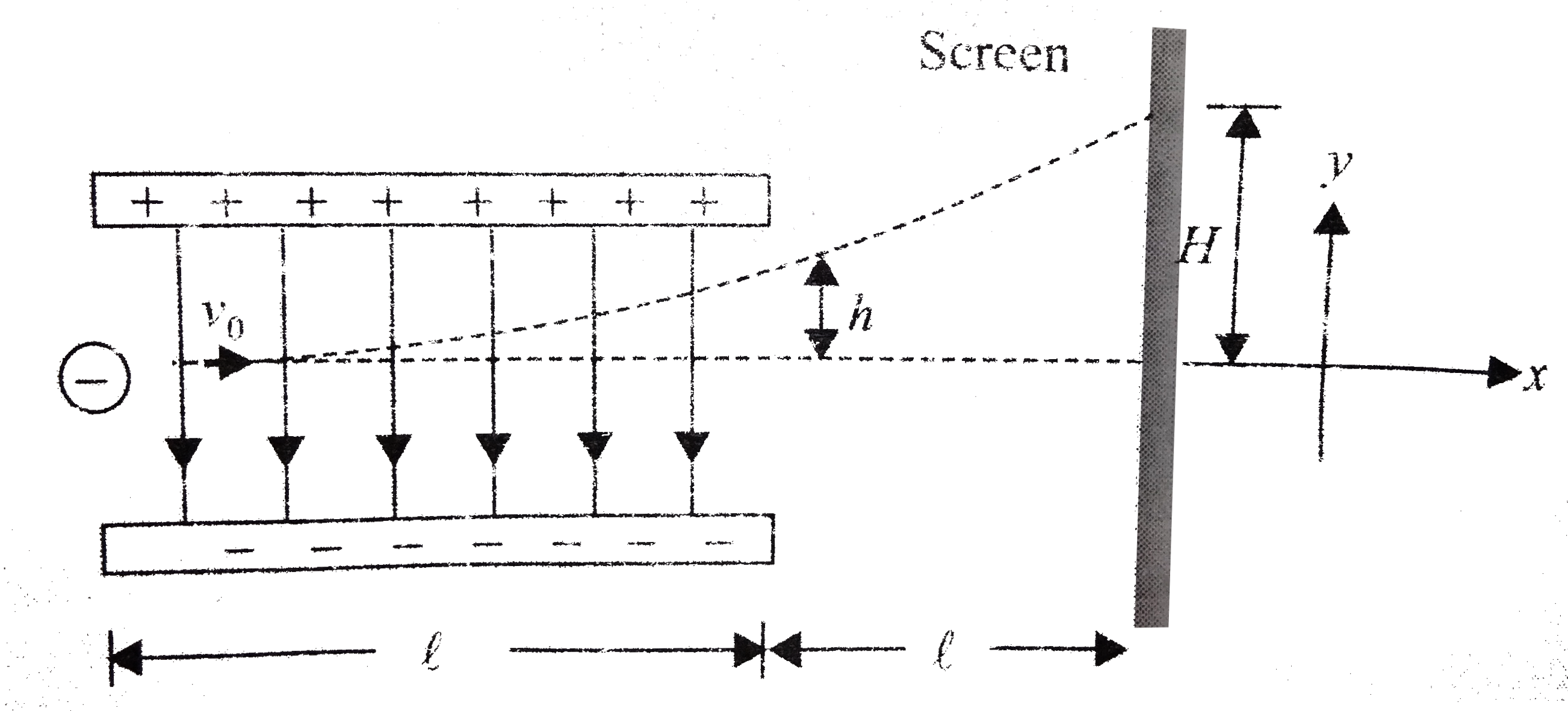
View SolutionAn electron moving in a gravitational free space enters a unifrom electric field E with an initial velocity shown in fig.
 .
.
(a) Find the deflection distance h in the field .
(b) Find an expression for the velocity of electron when it just emerage from the field.
(c ) Find an expression for the total deflection distance H at a vertical screen placed at a distance l from the region of uniform field .(Assume that the field abruptly ends outside the field. ).
View SolutionElectric Field due to Dipole
View SolutionElectric flux over a surface in an electric field
View SolutionIn the depletion layer, the electric field created
View SolutionIn the depletion layer, the electric field created
View SolutionCan a photon be deflected by an electric field ? By a magnetic field ?
View Solution