Consider a block connected to a light spring of spring constant . Now the block is displaced by applying a constant force F which gives zero resultant force when spring is stretched through 10 cm. 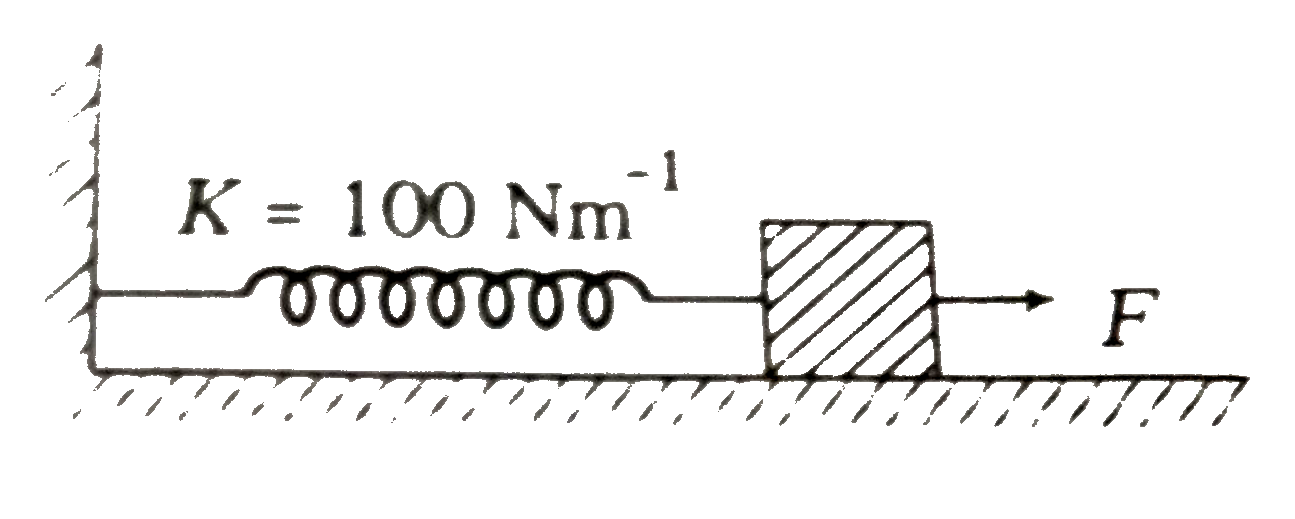
Evaluate
(i) Work done by the spring force when the block attains equilibrium.
(ii) Net work done on the block when it attains maximum speed.
(i) Work done by the spring force,
Negative sign indicates that the work done by the spring force is negative.
(ii) When the block attains equilibrium, its speed is maximum.
Work done on the block by external force F
Thus, net work done on the block
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.120videos
WORK, ENERGY AND POWER
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.220videos
WORK, ENERGY AND POWER
- BOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based2videos
WORK, ENERGY & POWER
- BOOK - DC PANDEYCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions11videos
WORK, POWER AND ENERGY
Similar Questions
20 J work is required to stretch a spring through 0.1 m . Find the force constant of the spring. If the spring is further stretched through 0.1 m. Calculate work done.
View SolutionA spring of spring constasnt 50 N/m is compressed from its natural position through 1 cm. Find the work done by the work done by the spring force on the agency compressing the spring.
View Solution
Knowledge Check
- Question 1 - Select One or More
A block is suspended by an ideal spring of force constant k. If the block is pulled down by applying a constant force F and if maximum displacement of the block from its initial position of rest is δ, then
A(a) Fk<δ>2FkB(b) δ=2FkC(c) Work done by force F is equal to FδD(d) Increase in energy stored in the spring is 12kδ2 - Question 2 - Select One
A 10 kg metal block is attached to a spring constant 1000Nm−1. A block is displaced from equilibrium position by 10 cm and released. The maximum acceleration of the block is
A10ms−2B100ms−2C200ms−2D0.1ms−2 - Question 3 - Select One
Work done for a certain spring when stretched through 1 mm is 10 joule. The amount of work that must be done on the spring to stretch it further by 1 mm is
A30 JB40 JC10 JD20 J
A block of mass m is connected to another block of mass M by a massless spring of spring constant k. the blocks are kept of a smooth horizontal plane and are at rest. The spring is unstretched when a constant force F starts acting on the block of mass M of pull it. Find the maximum extension of the spring
 View Solution
View SolutionA block of mass 'm' is connected to another block of mass 'M' by a spring (massless) of spring constant 'k' The blocks are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is unstretched Then a constant force 'F' starts acting on the block of mass 'M' to pull it. Find the force on the block of mass 'm' :
View SolutionA block of mass m is connected to another block of mass M by a spring (massless) of spring constant k. The block are kept on a smooth horizontal plane. Initially the blocks are at rest and the spring is unstretched. Then a constant force F starts acting on the block of mass M to pull it. Find the force of the block of mass M.
View SolutionBlocks A&B of mass m each are connected with spring of constant k both blocks lie on frictionless ground and are imparted horizontal velocity v as shown when spring is unstretched find the maximum stretch of spring.
 View Solution
View SolutionA block of mass 1kg is connected with a massless spring of force constant 100N/m. At t=0, a constant force F=10N is applied on the block. The spring is in its netural length at t=0. The speed of particel at x=6cm form mean position is
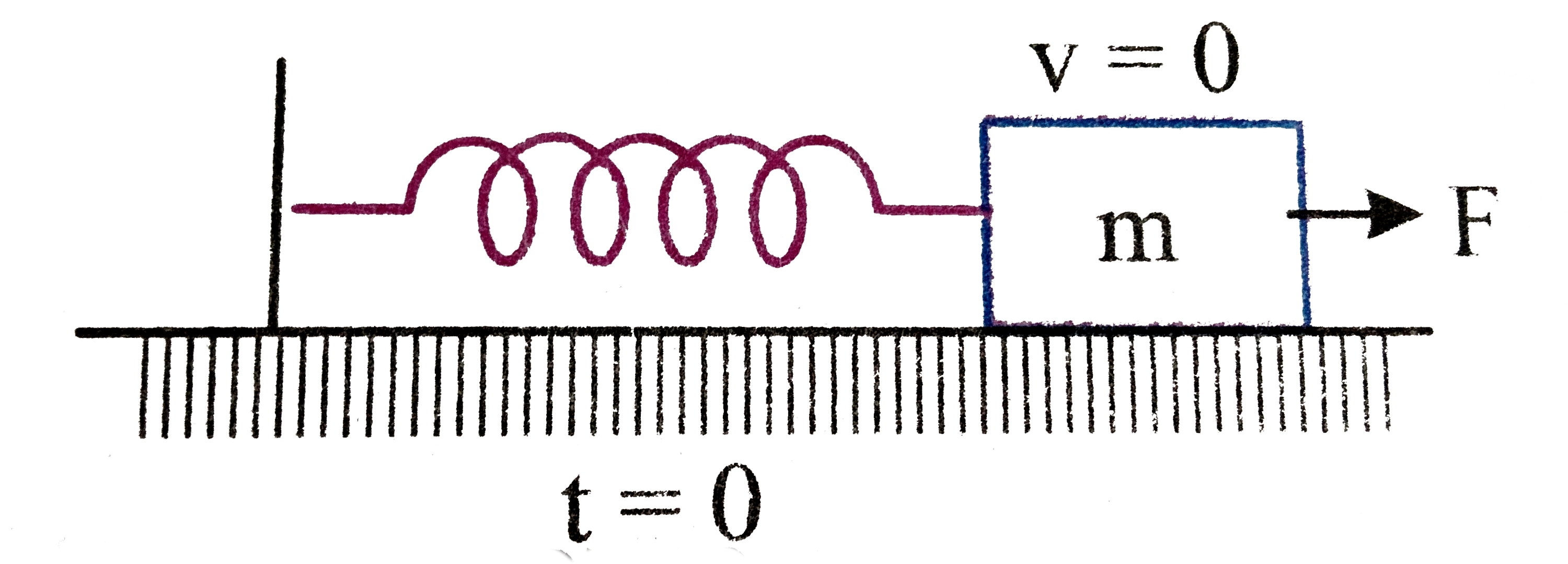 View Solution
View Solution

