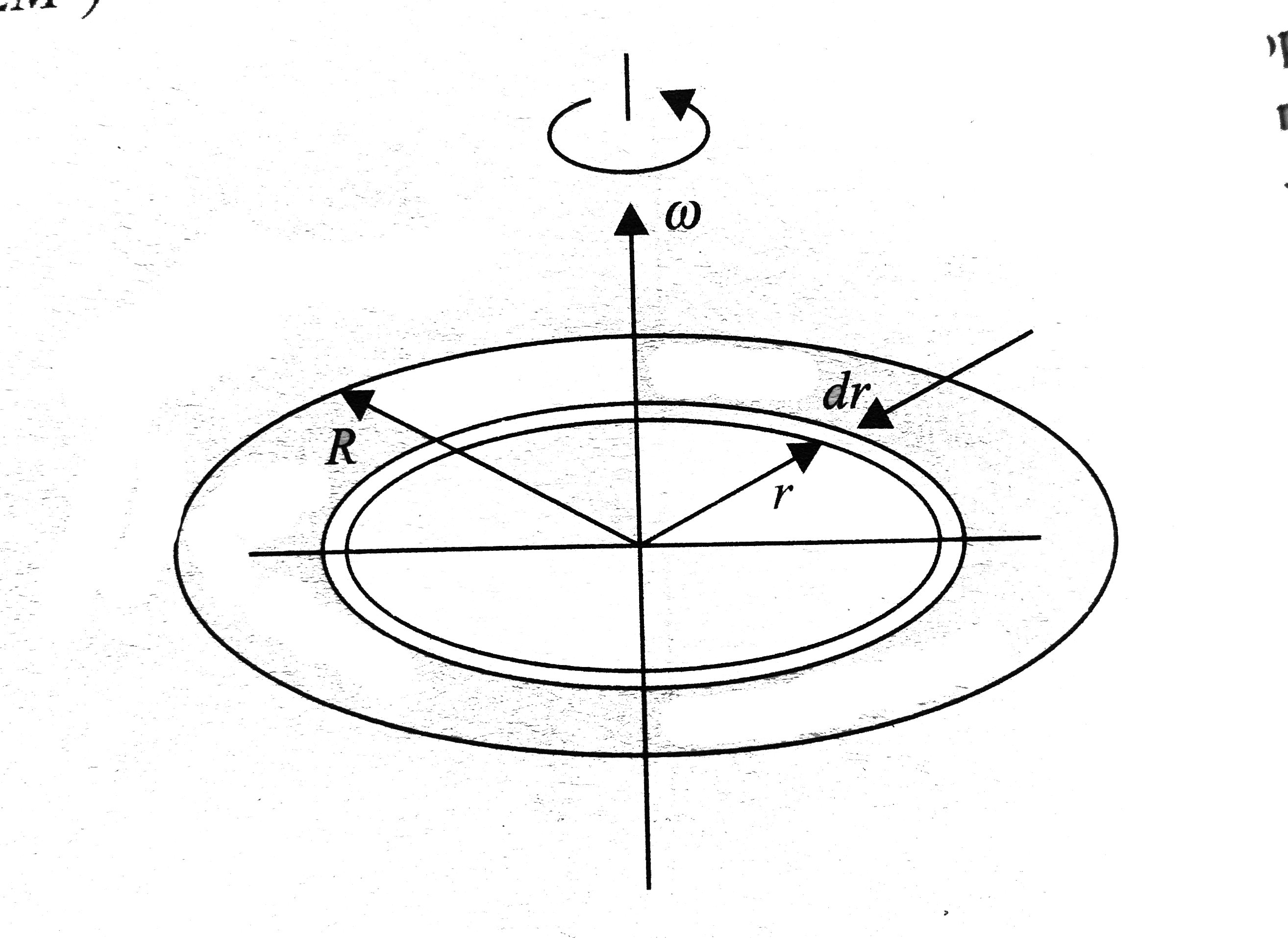
Consider a nonconducting plate of radius r and mass m which has a charge q distributed uniformly over it. The ring is rotated about its axis with an angular speed $ω$ Show that the magnetic moment $μ$ and the angular momentum I of the plate are rlated as $μ = \frac{q}{2 m} l .$

Video Solution

Text Solution

Verified by Experts

The charge is distributed on the surface of the disk.
We consider a differential ring of radius r and thickness dr.
The charge on the element is $d q = σ d A = σ (2 π r d r)$
The magnetic moment of the ring $d μ = (d I) A = (d I) π r^{2}$
The current in the differential ring
$d I = (d q) f = (σ d A) \frac{ω}{2 π} = (σ^{2} ω r d r) = σ ω r d r$
The magnetic moment of the differential ring,
$d μ (σ ω r d r) π r^{2} = π σ ω r^{3} d r$
$μ = \int d μ = \int_{0}^{R} π σ ω r^{3} d r = \frac{1}{4} π σ ω R^{4}$
The magnetic vector $\to μ$ is parallel to $\to ω$ if charge is
positive.
$\to μ = \frac{1}{4} π σ R^{4} \to ω$
In terms of total charge $Q = σ π R^{2}$ , the magnetic moment is
$\to μ = \frac{1}{4} Q R^{2} \to ω$
The angular momentum of disk is $v e L = (\frac{1}{2} M R^{2}) \to ω$ and
$\to μ = (\frac{Q}{2 M}) \to L$ .

This is a general result for any rigid body of any arbitrary shape
having mass M and charge Q.

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS ENGLISH-MAGNETIC FIELD AND MAGNETIC FORCES-Multiple Correct Answer type

Home
Ask Doubt
Profile