Consider a sphere of radius R and cylinder of length L. If both have same charge density σ and EsandEc are electric intensity at a point at a distance r from axis of sphere and cylinder respectively, then Es equal to
EcRr
EcrR
2EcrR
Ecr2R
The correct Answer is:A
EcEs=σR∈0krσR2∈0kr2
EcEs=Rr×r2R2=rR
∴Es=Ec⋅Rr
Topper's Solved these Questions
Similar Questions
Charge is uniformly distributed with density ρ in a cylindrical region of radius R and infinite length. The magnitude of electric field at distance R2 and 2R from the axis of the cylinder is E1 and E2 respectively. The ratio E1E2 is equal to ___________.
View Solution(a) There is a long uniformly charged cylinder having a volume charge density of ρC/m3. Radius of the cylinder is R. Find the electric field at a point at a distance x from the axis of the cylinder for following cases (i) x lt R (ii) x gt R What is the maximum field produced by the charge distribution at any point? (b) The cylinder described in (a) has a long cylindrical cavity. The axis of cylindrical cavity is at a distance a from the axis of the charged cylinder (see figure). Find electric field inside the cavity.
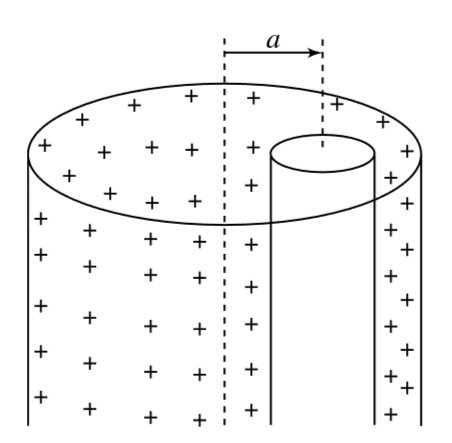
View Solution
Knowledge Check
A sphere of radius R has a charge density σ. The electric intensity at a point at a distance r from its centre is
AE=σR2∈0kr2BE=σ∈0kCE=R2DE=∈0kr2σ2A cylinder of length L has a charge of magnitude q. The electric intensity at a point at a distance r from the axis of the cylinder is
AE=14π∈0K⋅qr2BE=12π∈0k⋅qrLCE=σ/∈0kDnone of theseIf σ is surface density of charge on the charged cylinder of radius R, then electric intensity E at outer point at a distance r from its axis is
AσR∈0krBσr∈0kRCσ∈0kDσ2R∈0kr
If charge q is induced on outer surface of sphere of radius R, then intensity at a point P at a distance S from its centre is
View SolutionIf charge q induced on outer surface of sphere of radius R, then intensity at point P at distance S from centre is
View SolutionTwo concentric spheres of radii R and r have similar charges with equal surface densities (σ) What is the electric potential at their common centre /
View SolutionTwo concentric spheres of radii R and r have similar charges with equal surface densities (σ) What is the electric potential at their common centre /
View SolutionThe electric field at a distance r from a long wire having charge per unit length λ is
View Solution
