Consider an interference arrangement similar to YDSE. Slits S1 and S2 are illuminated with coherent microwave sources each of frequency 2 MHz The sources are synchronized to have zero phase difference. The slits are separated by distance d= 75m. The intensity I(θ) is measured as a function of θ where θ is defined as shown in figure. if I0 is maximum intensity then calculated I(θ) for (i)θ=0∘,(ii)θ=π/6and(iii)θ=π/2
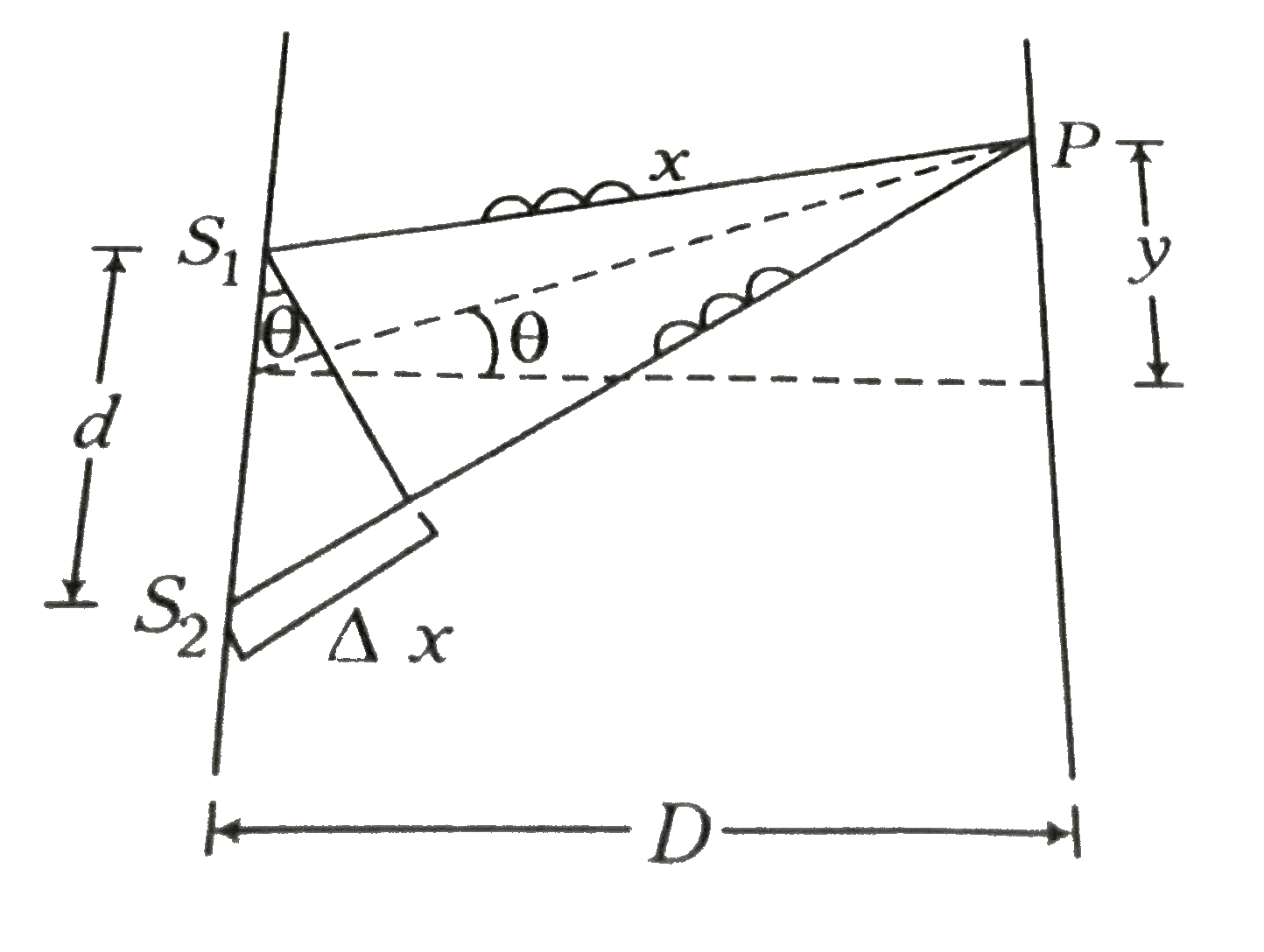
Given v=2 MHz=106 Hz d=75m
As, c=vλ
⇒λ=cv=3×1082×106=150m
Path difference Δx=dsinθ
Phase difference θ=2πλΔx=2πλdsinθ=2π150×75sinθ
=πsinθ
Intensity at point P, I=I0cos2(θ/2)=I0cos2(πsinθ2)
(i)θ=0,I=I0
(ii)θ=π6=30∘
I=I0cos2(πsin30∘2)=I0cos2(π/4)=I02
(iv)θ=π2=90∘,I=0
Topper's Solved these Questions
Similar Questions
In an interference arrangement similar to Young's double-slit experiment, the slits S_1 and S_2 are illuminated with coherent microwave sources, each of frequency 10^6 Hz. The sources are synchronized to have zero phase difference. The slits are separated by a distance d=150.0 m. The intensity I (theta) is measured as a function of theta, where theta is defined as shown. If I_0 is the maximum intensity, then I (theta) for 0lethetale90degree is given by
 View Solution
View Solution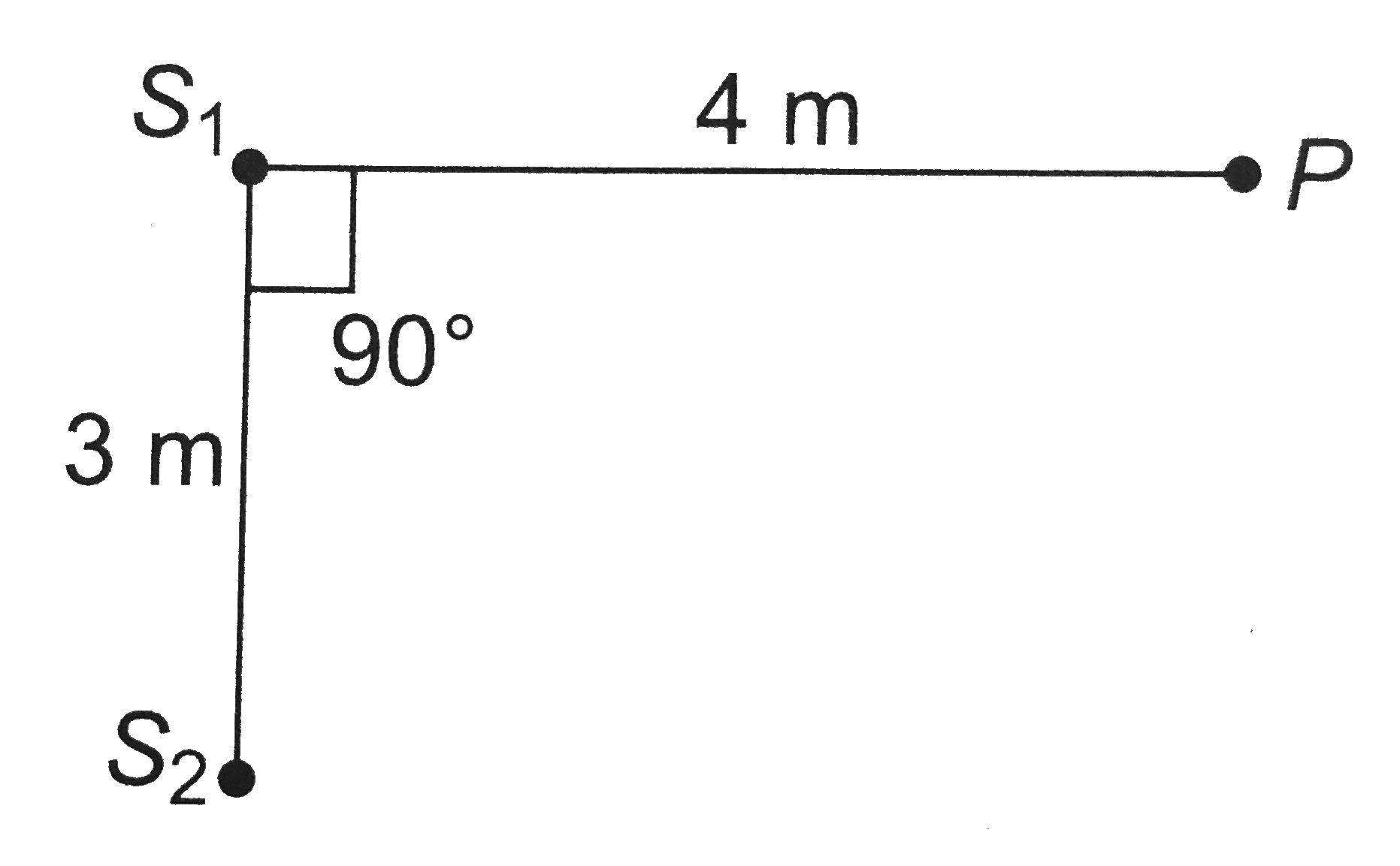
S1 and S2 are two coherent sources of sound of frequency 110Hz each they have no initial phase difference. The intensity at a point P due to S1 is I0 and due to S2 is 4I0. If the velocity of sound is 330m/s then the resultant intensity at P isView Solution
Knowledge Check
- Question 1 - Select One
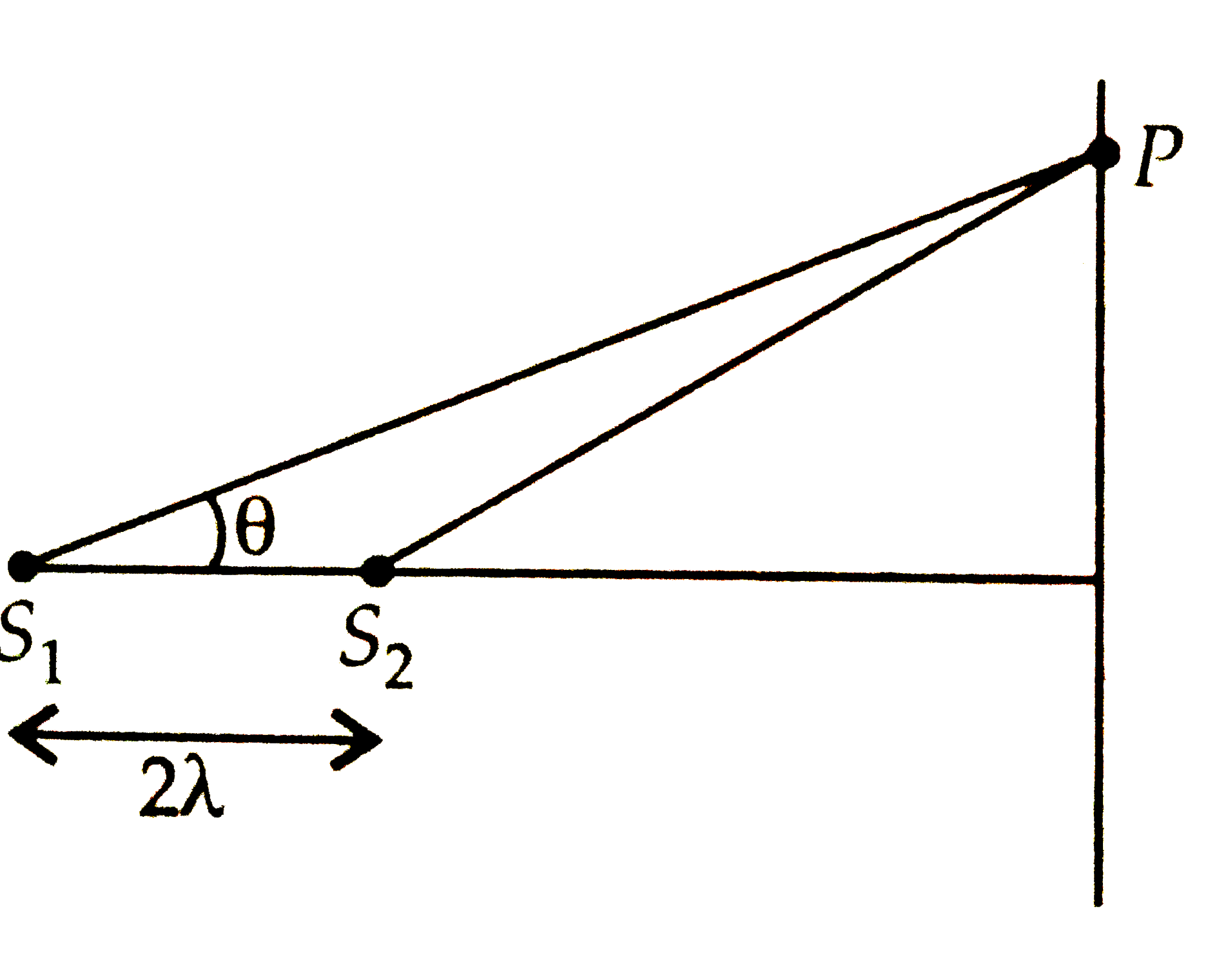
In Young's double slit experiment, the slits are horizontal. The intensity at a point P as shown in figure is 34I0,whereI0 is the maximum intensity.
Then the value of θ is,
(Given the distance between the two slits S1 and S2 is 2λ)
 Acos−1(112)Bsin−1(112)Ctan−1(112)Dsin−1(35)
Acos−1(112)Bsin−1(112)Ctan−1(112)Dsin−1(35)
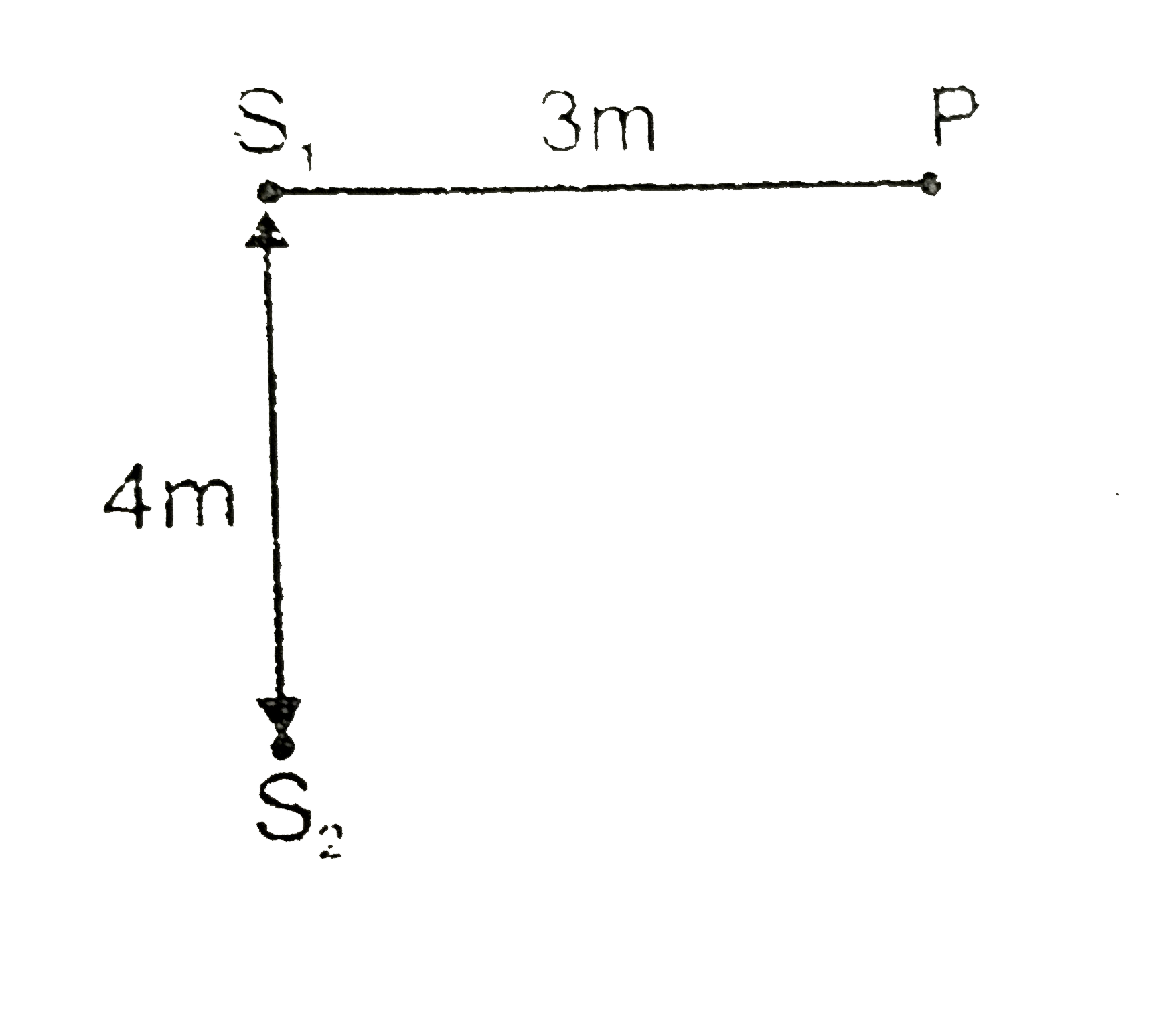
S1 and S2 are two coherent sources of frequency f each.(θ1=θ2=0∘)Vsound=330m/s.
(i) so that constructive interfence at 'p'
(ii) so that destructive interfrece at 'p'
 View Solution
View SolutionTwo coherent sources each emitting light of intensity I0 Interfere, in a medium at a point, where phase different between them is 2π3. Then, the resultant intensity at that point would be.
View SolutionDerive formulas for the following in terms of functions of 2θand and then of θ.
(i)sin4θ
(ii) cos4θ,
(iii) tan4θView SolutionFind the value of θ in each of the following: (i)2sin2θ=√3 (ii)2cos3θ=1 (iii) √3tan2θ−3=0
View SolutionFind the value of θ in each of the following: (i)2sin2θ=√3 (ii)2cos3θ=1
View SolutionConsider interference between waves form two sources of intensities I0&4I0.Find intensities at point where the phase difference is π
View SolutionTwo identical sources each of intensity I0 have a separation d=λ/8, where λ is the wavelength of the waves emitted by either source. The phase difference of the sources is π/4 The intensity distribution I(θ) in the radiation field as a function of θ Which specifies the direction from the sources to the distant observation point P is given by
View Solution

