Question

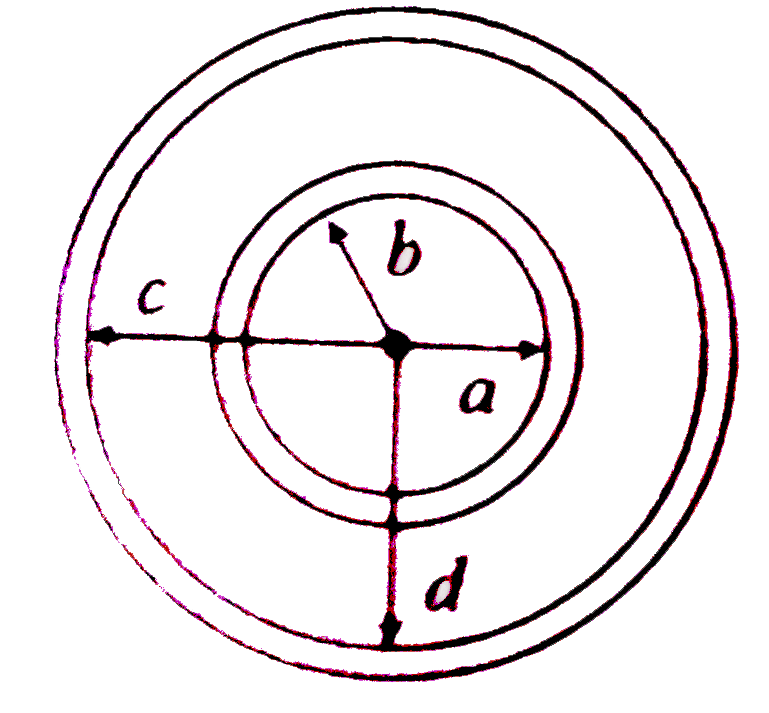
Consider the previous problem , let the outer shell have the charge $-4q$. As in the above problem , the inner shell has the charge $+2q$. Calculate the electric field in terms of $q$ and the distance $r$ from the common center of the two shells for
$r<a$

Options

A. zero
B. `(1)/( 4 pi epsilon_(0)) ( 2q)/( r^(2))`
C. `-(1)/( 4 pi epsilon_(0)) ( 2q)/( r^(2))`
D. `(1)/( 4 pi epsilon_(0)) ( 6 q)/( r^(2))`

Answer

A

Explanation

To solve the problem, we need to calculate the electric field inside the inner shell (where $$r<a$$) given the charges on the inner and outer shells.

1. Identify the Charges:
- The inner shell has a charge of \( +2q \).
- The outer shell has a charge of \( -4q \).

2. Understand the Region of Interest:
- We are interested in the region where \( r < a \), which is the space inside the inner shell.

3. Apply Gauss's Law:
- Gauss's Law states that the electric flux through a closed surface is proportional to the enclosed charge:
\( \Phi_E = \oint \vec{E} \cdot d\vec{A} = \frac{Q_{\text{enc}}}{\epsilon_0} \)
- Here, \( \Phi_E \) is the electric flux, \( \vec{E} \) is the electric field, \( d\vec{A} \) is the differential area vector, and \( Q_{\text{enc}} \) is the total charge enclosed by the Gaussian surface.

4. Choose a Gaussian Surface:
- We choose a spherical Gaussian surface of radius \( r \) (where \( r < a \)) centered at the common center of the two shells.

5. Calculate the Enclosed Charge:
- Inside the inner shell (for \( r < a \)), the enclosed charge \( Q_{\text{enc}} \) is \( 0 \) because the charge of the inner shell does not contribute to the electric field inside it.
- Thus, \( Q_{\text{enc}} = 0 \).

6. Apply Gauss's Law:
- Substituting \( Q_{\text{enc}} = 0 \) into Gauss's Law:
\( \oint \vec{E} \cdot d\vec{A} = \frac{0}{\epsilon_0} = 0 \)
- This means that the total electric flux through the Gaussian surface is zero.

7. Conclusion about the Electric Field:
- Since the electric flux is zero and the area of the Gaussian surface is non-zero, the electric field \( E \) must also be zero in this region:
\( E = 0 \quad \text{for } r < a \)

Final Answer:
The electric field \( E \) in the region where \( r < a \) is:
\( E = 0 \)