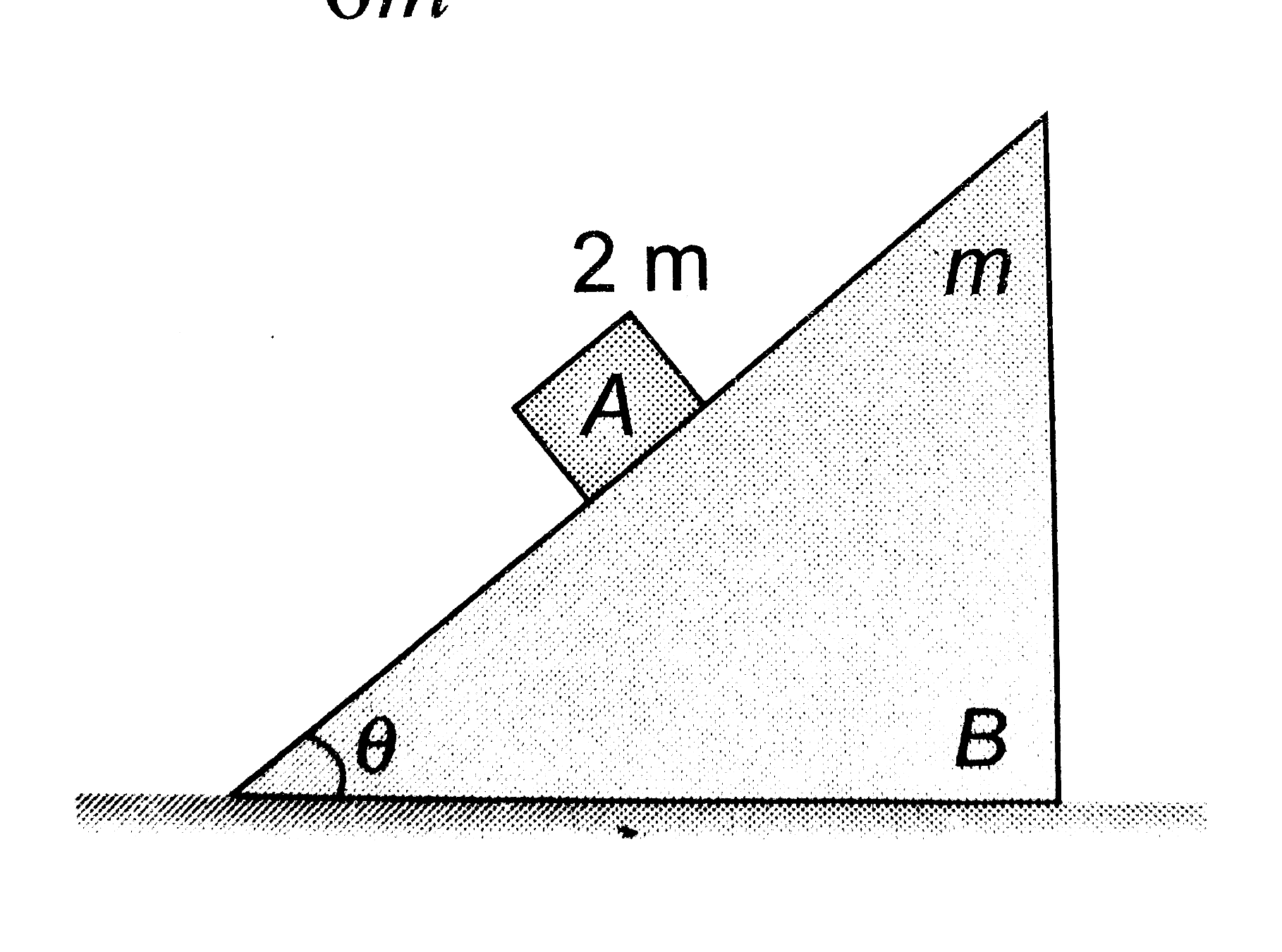
Consider the shown arrangement. Assume all surfaces to be smooth. If N represents magnitudes of normal reaction between block and wedge, then acceleration of M along horizontal is equla to
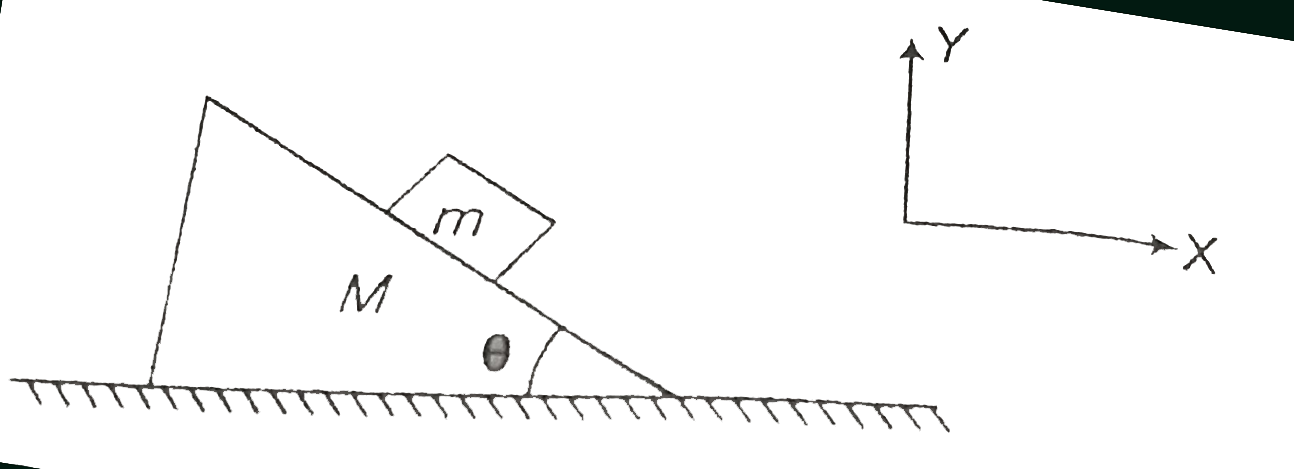
NsinθM along + veX-axis
NcosθM along - veX-axis
NsinθM along - veX-axis
Nsinθm+M along - veX-axis
The correct Answer is:C
( c) Wedge move due to horizontal component of normal reaction.
Thus a=NHM=NsinθM (along-ve X-axis)
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Assertion and reason
28 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Match the columns
7 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.4
20 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
Assuming all the surfaces to be smooth, find the acceleration of the triangular block of mass M when the block of mass m slides on it.

View SolutionIn the figure shown, all surfaces are smooth and block. A and wedge B have mass 10 kg and 20 kg respectively. Find normal reaction between block A &B, spring force and normal reaction of ground on block B. (g=10m/s2).
.
View Solution
Knowledge Check
In the above problem normal reaction between ground and wedge will have magnitude equla to
ANcosθ+MgBNcosθ+Mg+mgCNcosθ−MgDNsinθ+Mg+mgIn the figure shown, all surfaces are smooth and the pulley is massless. A constant force of magnitude F=mg2 is acting on the block of mass M. The acceleration of the block M is :
 Amg2(m+M) towards rightBmg2(m−M) towards rightsCmg2(m−M) towards leftDmg2(m+m) toward left
Amg2(m+M) towards rightBmg2(m−M) towards rightsCmg2(m−M) towards leftDmg2(m+m) toward leftAll surfaces are smooth. The acceleration of mass m relative to the wedge is
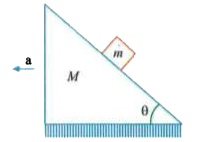 AgsinθBgsinθ+acosθCgsinθ−acosθDacosθ
AgsinθBgsinθ+acosθCgsinθ−acosθDacosθ
All surfaces are smooth Find the horizontal displacements of the block and the wedge when the block slides down from top to bottom
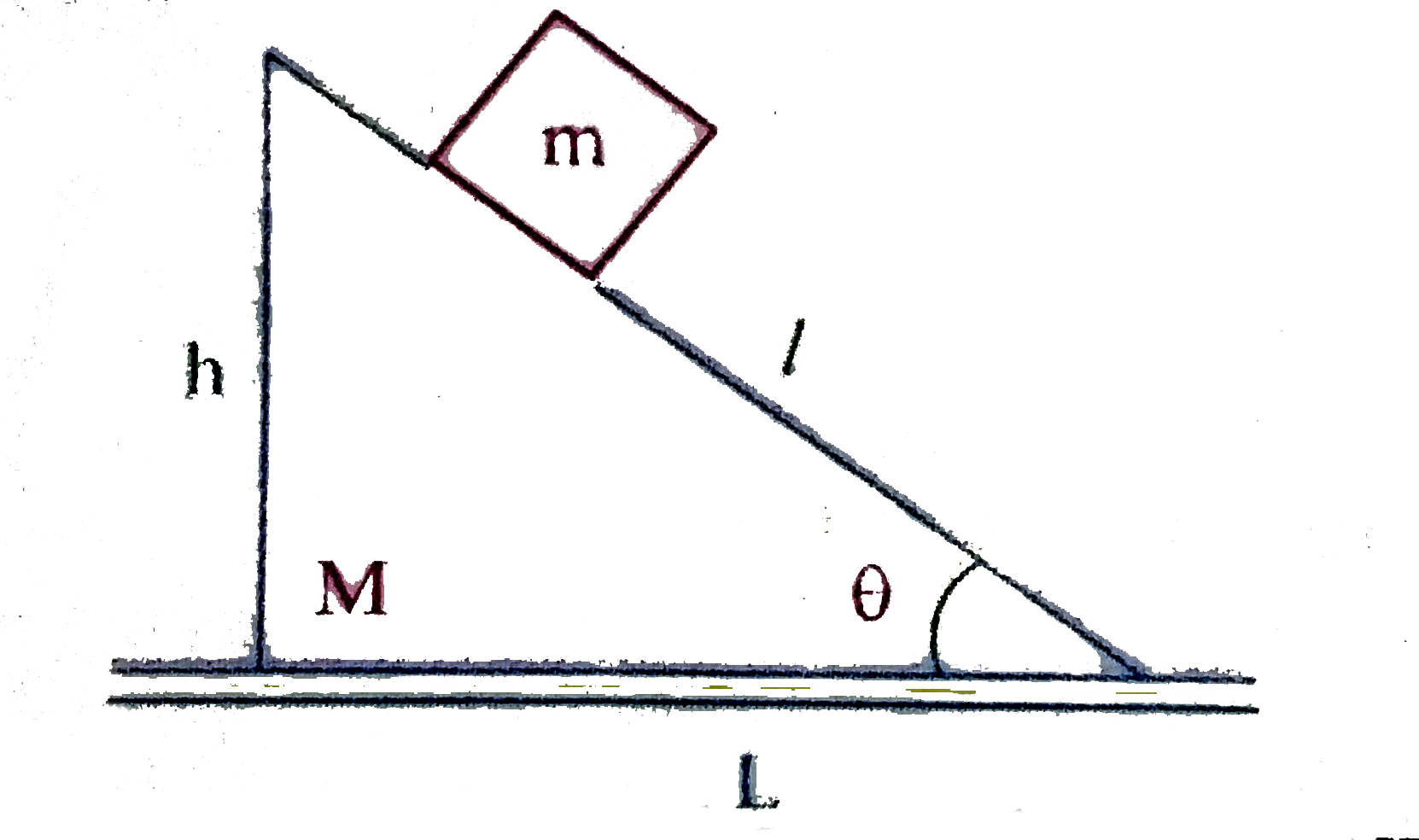 .
.
View SolutionConsider the situation shown in figure. All the surfaces are frictions and the string and the pulley are light. Find the magnitude of the acceleration of the two blocks.

View SolutionAll surfaces are smooth The acceleration of mass m relative to the wedge is
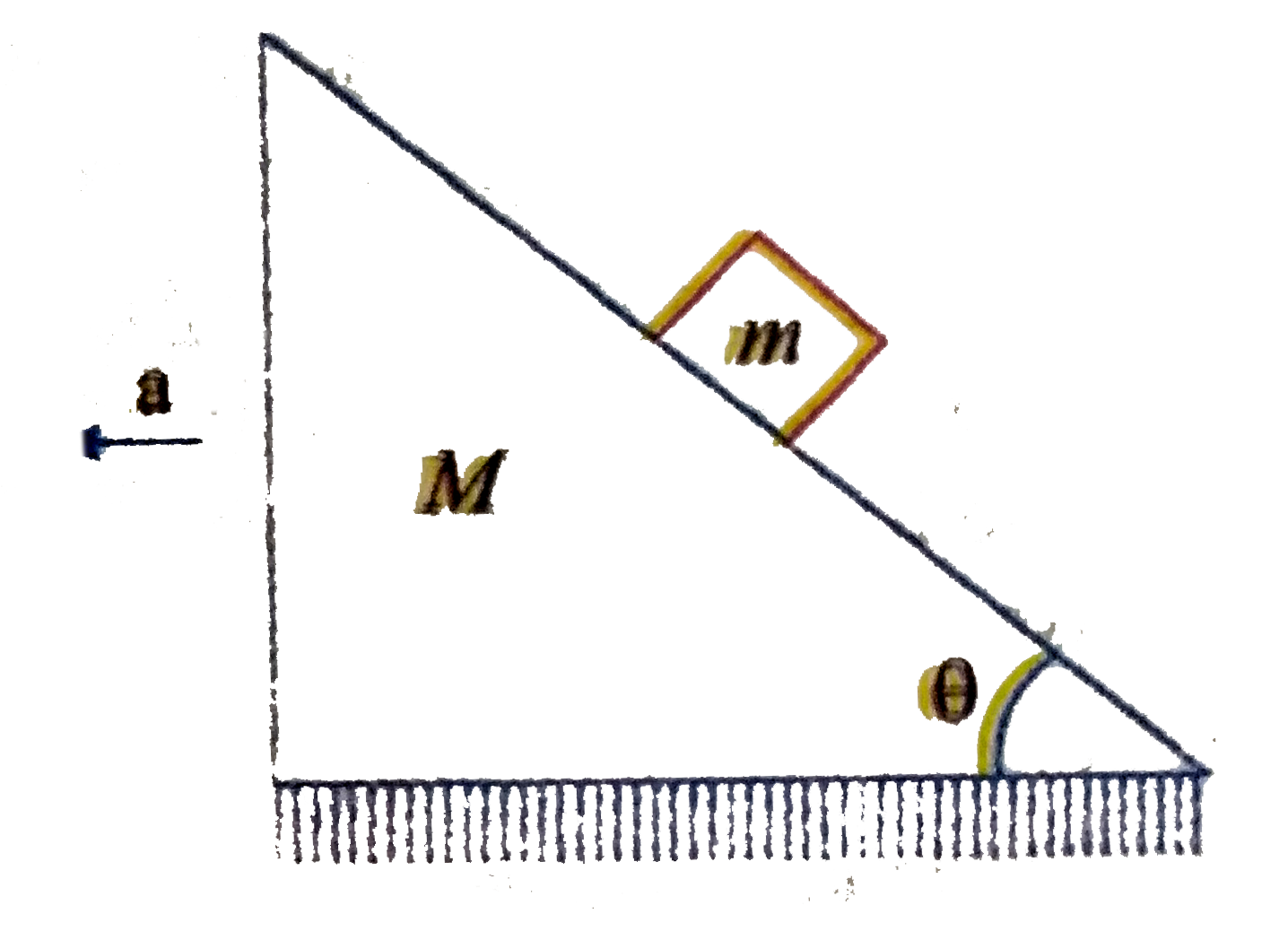 .
.
View SolutionAn arrangement is shown in figure. If all the surfaces are smooth and system is released from rest then the relation between accelerations of blocks P(ap)andQ(a_Q) will be
View SolutionIn the figure if block A and wedge b will move with same acceleration, then the magnitude of normal reaction between the block and the wedge will be (There is no friction between block and the wedge and the wedge moves on horizontal surface as shown.)
View Solution
