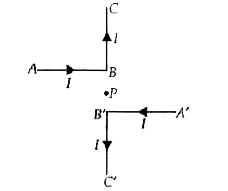
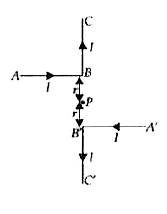
Current through ABC and A'B'C' is 1. What is the magneticfield at P? BP=PB=r(henceC'B'andpbc are collinear)

$B = \frac{1}{4 π} \frac{2 I}{r}$

$B = \frac{μ_{0}}{4 π} (\frac{2 I}{r})$

$B = \frac{μ_{0}}{4 π} (\frac{I}{r})$

zero

Text Solution

Verified by Experts

The correct Answer is:B

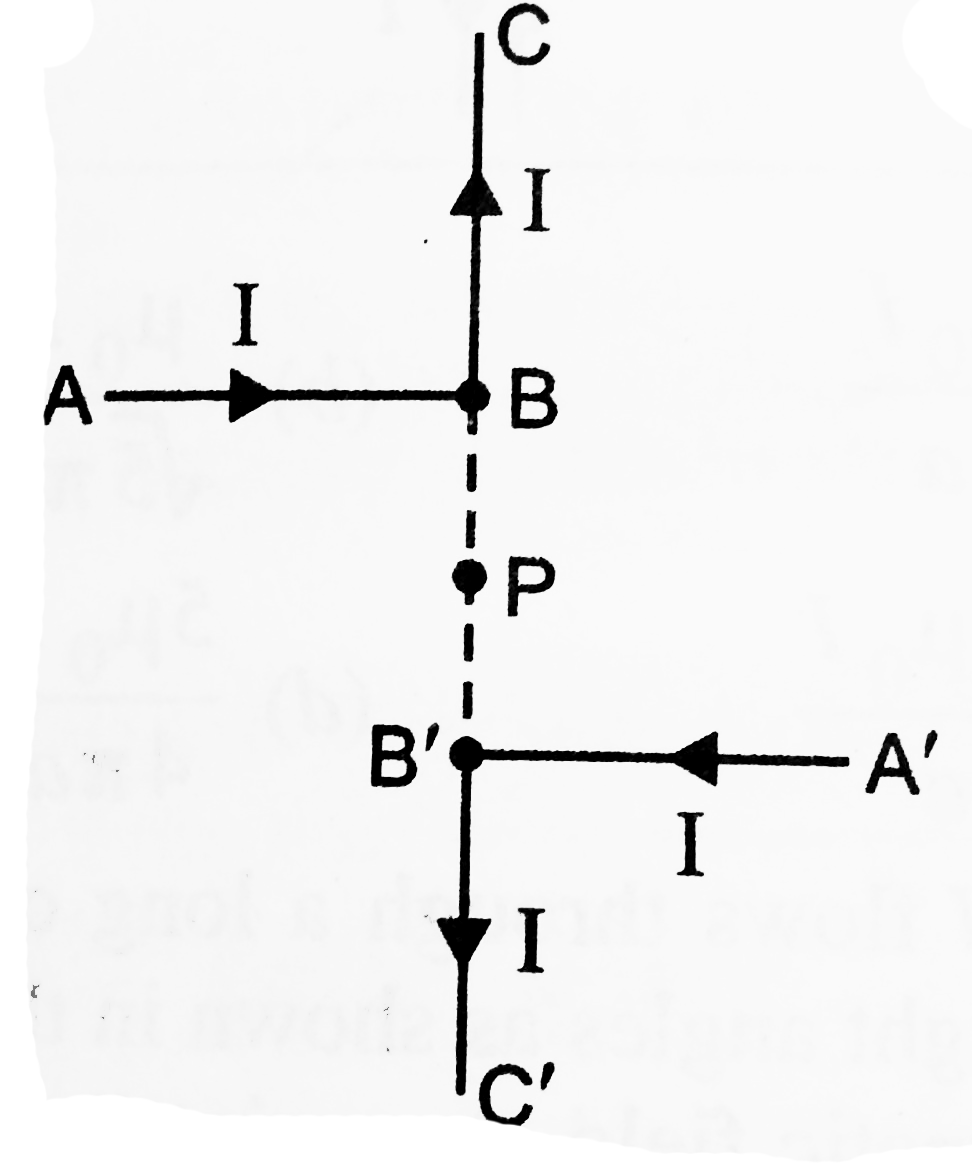
Current through ABC A'B'C' is I. What is the magnetic field at P? BP= PB' = r (Here C'B' PBC are collinear)

A $B = \frac{1}{4 π} \frac{2 I}{r}$
B $B = \frac{μ_{0}}{4 π} (\frac{2 I}{r})$
C $B = \frac{μ_{0}}{4 π} (\frac{I}{r})$
Dzero
Currents through ABC and $A^{'} B^{'} C^{'}$ is I, figure. What is the magnetic field at P? $B P = P B^{'} = r$ . (Here $C^{'} B^{'} P B C$ are collinear)

A $\frac{2 I}{4 π r}$
B $\frac{μ_{0}}{4 π} (\frac{2 I}{r})$
C $\frac{μ_{0}}{4 π} (\frac{1}{r})$
Dzero
A sphere of constant radius r through the origin intersects the coordinate axes in A, B and C What is the locus of the centroid of the triangle ABC?
A $x^{2} + y^{2} + z^{2} = r^{2}$
B $x^{2} + y^{2} + z^{2} = 4 r^{2}$
C $9 (x^{2} + y^{2} + z^{2}) = 4 r^{2}$
D $3 (x^{2} + y^{2} + z^{2}) = 2 r^{2}$