Define electric dipole. Show that the electric field is the negative gradient of its potential.
Electric dipole is a pair of equal and opposite point charges separated by a finite distance.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Long Answer Questions)
13 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Numerical Problems)
25 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Fill in the Blanks)
11 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
(Fig. 3.95) shows four orientations of an electric dipole in an external electric field. Rank the orientations acording to the
a. magnitude of the torque on the dipole, and
b. potential energy of the dipole, greatest first.
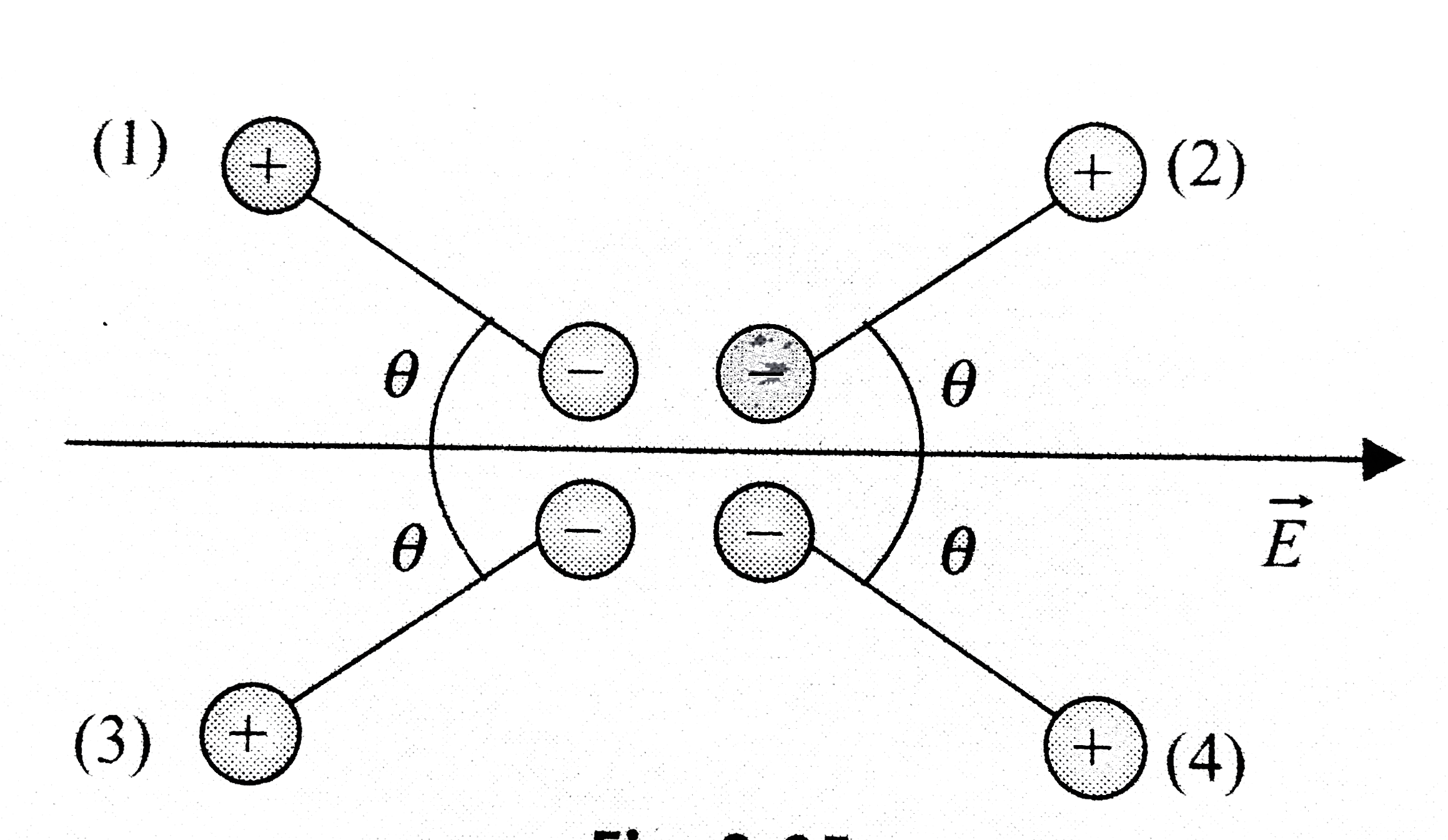 .
.
View SolutionThe angle between the electric dipole moment and electric field due to the dipole on its axial and equatorial lines respectively are
View Solution
Knowledge Check
For an electric dipole potential & electric field
Acan have same magnitude for a point at a distance less than 2 meterBcan have same magnitude fo a point at distacnes greater than 2 meterCcan never be equalDcan never be predictedAn electric dipole is placed in uniform electric field. The magnitude of electric dipole moment of dipole is p and external electric field intensity is E. Asume θ is the angle between electric dipole moment and electric field intensity and U represents potential energy of electric dipole
AIf U=0 for θ=0 then for other orientations U=pE(1−cosθ)BIf U=0 for θ=90∘ then for other orientations U=−pEcosθCIf U=0 for θ=180∘ then for other orientations U=−pE(1+cosθ)DAll of the aboveElectric field due to an electric dipole is
Aspherically symmetricBcylindrical symmetricCasymmetricDnone of the above
a) Define an ideal electric dipole. Give an example.
b) Derive an expression for the torque experienced by an electric dipole in a uniform electric field. What is net force acting on this dipole.
c) An electric dipole of length 2cm is placed with its axis making an angle of 60∘ with respect to uniform electric field of 105N/C. If it experiences a torque of 8√3 Nm, calculate the (i) magnitude of charge on the dipole, and its potential energy
View SolutionDerive an expression for potential energy of an electric dipole in a uniform electric field. In which situation, the potential energy fo dipole is (i) maximum and (ii) minimum.
View SolutionElectric field due to an electric dipole is
View SolutionAn electric dipole when placed in uniform electric field has zero potential energy. The angle between dipole moment and electric field is ....
View SolutionAn electric dipole placed in a nonuniform electric field experience
View Solution
