Derive the formula expressing molar heat capacity of a unidimensional crystal, a chain of identical atoms, as a function of temperature T if the Debye temperature of the chain is equal ot Θ. Simplyfie the octained expression for the case T>Θ
In the Debye approximation the number of modes per unit frequency interval is given by
dN=1πvdω0≤ω≤kΘħ
But kΘħ=πn0v
Thus dN=lπvdω,0≤ω≤πn0v
The enrgy per mode is
<E≥12ħω+ħωeħω/kT−1
The total interval energy of the chain is
U=lπv∫πn0v012ħωdω
+lπv∫πn0v0ħωeħω/kT−1dω=lħ4πv(πn0v)2+lħ4πv(πn0v)2+lπvħ(kT)2∫Θ/T0xdxex−1=ln0k.ħk(πn0v).14
+ln0kT2(πn0vħ/k)∫Θ/T0xdxex−1
We put ln0k=R for 1 mole of the chain
Then U=RΘ{14+(IΘ)2∫Θ/T0xdxex−1}
Hence the molar heat capacity is by defferentiation
Cv=(∂U∂T)=R[2(TΘ)∫Θ/T0xdxex−1−Θ/Tee/T−1]
when T>>Θ,Cv≈R
Topper's Solved these Questions
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Radioactivity
35 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Nuclear Reaction
42 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Properties Of Atom
69 Videos
- DIRECT CURRENTBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - DIRECT CURRENTEXERCISE - All Questions
15 Videos
Similar Questions
Demostrate that molar heat capacity of a crystal at a temperature T<Θ, where Θ is the Debye temperature, is defined by Eq. (6.4f)
View SolutionAt very low temperatures the heat capacity of crystals os equal to C=aT3, where a is a constant. Find the entropy of a crystal as a function of temperature in this temperature interval.
View Solution
Knowledge Check
Two moles of an ideal mono-atomic gas undergoes a thermodynamic process in which the molar heat capacity 'C' of the gas depends on absolute temperature as C=RTT0, where R is gas consant and T0 is the initial temperature of the gas. (V0 is the initial of the gas). Then answer the following questions:
The equation of process isA1P=V0T3/204RT52e(T−T0T0)B1P=V0T3/202RT52e(T−T0T0)C1P=V0T3/204RT52e(T+T0T0)D1P=V0T3/203RT52e(T−T0T0)Two moles of an ideal mono-atomic gas undergoes a thermodynamic process in which the molar heat capacity 'C' of the gas depends on absolute temperature as C=RTT0, where R is gas consant and T0 is the initial temperature of the gas. (V0 is the initial of the gas). Then answer the following questions:
The minimum volume of gas isA(23)3/2V0e1/2B(43)3/2V0e1/2C(53)3/2V0e1/2D(83)3/2V0e1/2According to Dulong & Petits law, molar heat capacity of solids at room temperature is nearly equal to 3R. This can be explained using law of equipartition. Each atom of solid is boned to neighboring atoms by interatomic forces. Each atom can vibrate about its equilibrium positum. So each atom has 6 degrees of freedom. But in relaity, the molar heat capacity of solids varies. with temperature as shown in figure.
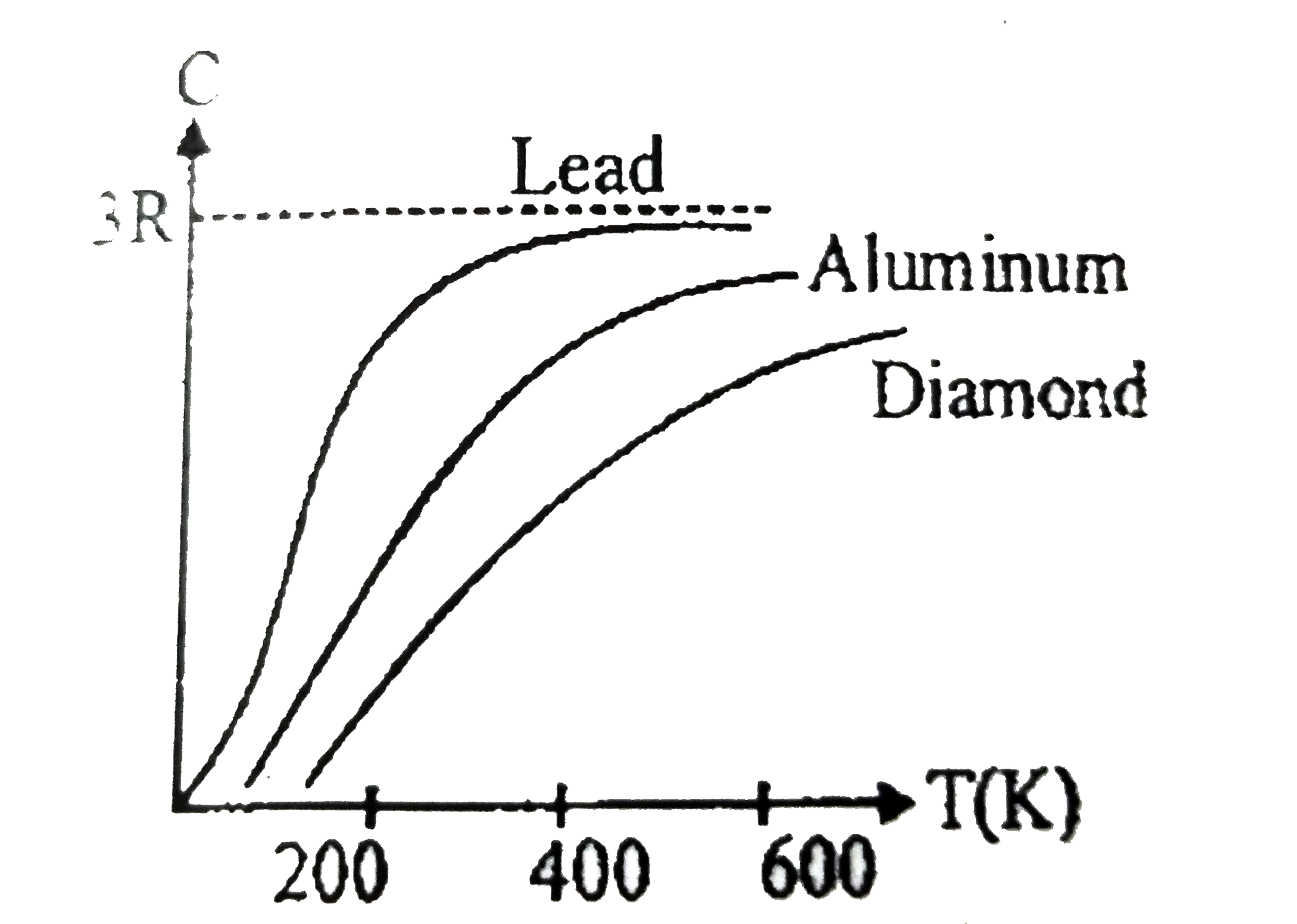
At low temperature, the heat capcities decrease with decreasing temperature. This is due to the fact that variational degrees of freedom are frozen out at low temperatures.
Selec the correct statement(s).AA sample of lead requires less heat to go from 100K to 150K then to go from 300K to 350KBA sample of lead requires more heat to go from 400K to 440K then to go from 100K to 140KCFor diamond at room temperatures also vibrational degrees of freedom are partially frozen.DThe specific heat capacity of all solids at high temperature is nearly the same.
A water vapour filling the space under the piston of a cylinder is compressed (or expanded) so that it remaind saturated all the time, being just on the verge of condensation. Find the molar heat capacity C of the vapour in this process as a function of temperature T, assuming the vapour to be an ideal gas and neglecting the specific volume of water in comparion with that of vapour. Calculate C at a temperature t=100∘C.
View SolutionAssuming the propagation velocities of longitudinal and transverse vibrations to be the same and equal to v, find the Debye temperature
(a) for a unidimensional crystal, i.e., a chain identical atoms, incorporating n0 atoms per unit length,
(b) for a two-dimensional crystal, i.e., a plance square grid consisting of identical atoms, containing n0 atoms per unit area,
(c ) for a simple cubic lattice consisting of identical atoms, containing n0 atoms per unit volume.
View SolutionTwo moles of an ideal mono-atomic gas undergoes a thermodynamic process in which the molar heat capacity 'C' of the gas depends on absolute temperature as C=RTT0, where R is gas consant and T0 is the initial temperature of the gas. (V0 is the initial of the gas). Then answer the following questions:
The equation of process is
View SolutionTwo moles of an ideal mono-atomic gas undergoes a thermodynamic process in which the molar heat capacity 'C' of the gas depends on absolute temperature as C=RTT0, where R is gas consant and T0 is the initial temperature of the gas. (V0 is the initial of the gas). Then answer the following questions:
The minimum volume of gas is
View Solutionm' kg of a substance of specific beat capacity J/kg∘C is heated, so that its temperature rises from θ∘1C to θ∘2C. Write down the expression for the heat Q supplied.
View Solution
