Equal current I flows in two segments of a circular loop in the direction shown in figure. Radius of the loop is 7. The magnitude of magnetic field induction at the centre of the loop O is
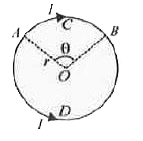
zero
μ0Iθ4πr
μ0Iθ2πr(π−θ)
μ0Iθ2πr(2π−θ)
The correct Answer is:C
Magnetic field induction at O due to current through ACB is
B1=μ04πIθr
It is acting perpendicular to the paper downwards . Magnetic field induction at O due to current through ABD is
B1=μ04πIr(2π−θ)
It is acting perpendicular to paper upward ∴ Total magnetic field at O due current loop is
B=B2−B1=μ04πIr(2π−θ)−μ04πIrθ=μ04πIr(π−θ)
acting perpendicular to paper upwards.
Topper's Solved these Questions
- BOOK - MTG GUIDECHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - NEET CAFE (TOPICWISE PRACTICE QUESTIONS)(AMPER.S CIRCUITAL LAW AND ITS APPLICATIONS)8videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
- BOOK - MTG GUIDECHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - NEET CAFE (TOPICWISE PRACTICE QUESTIONS)(FORCE ON A MOVING CHARGE IN UNIFORM ELECTRIC AND MAGNETIC FIELDS)37videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
- BOOK - MTG GUIDECHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - AIPMT/NEET (MCQs)48videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
- BOOK - MTG GUIDECHAPTER - ELECTROSTATICSEXERCISE - AIPMT/NEET (MCQ.s)34videos
ELECTROSTATICS
- BOOK - MTG GUIDECHAPTER - OPTICSEXERCISE - AIPMT/NEET55videos
OPTICS
Similar Questions
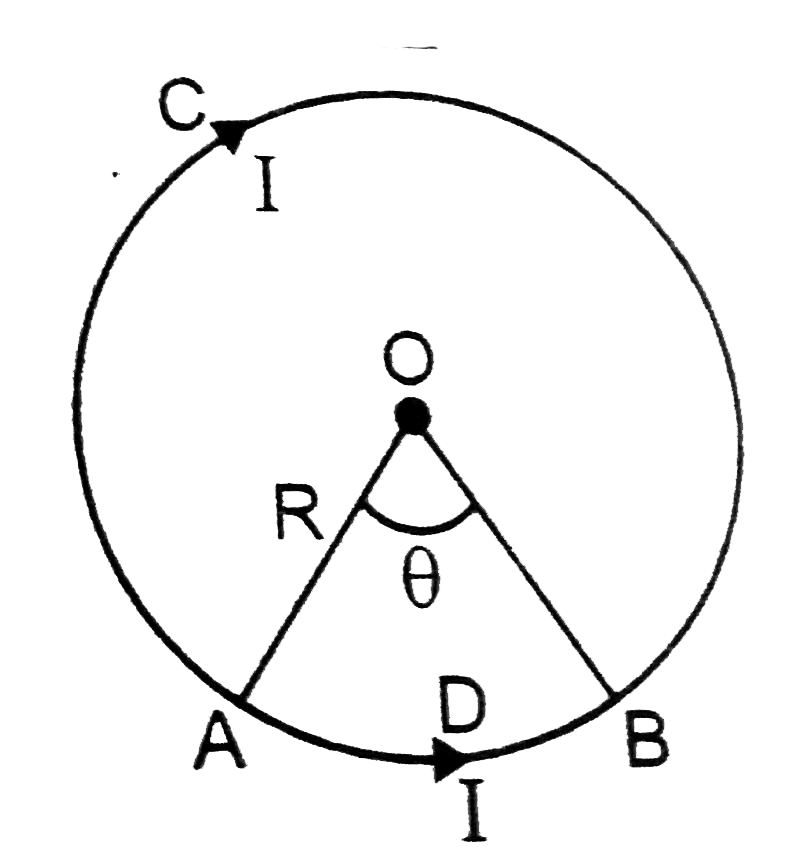
Equal current I flows in two segments of a circular loop in the direction shown in figure. Radius of loop is R. What is the magnitude of magnetic field induciton at the centre of the loop?
 View Solution
View SolutionEqual current I flows in two segments of a circular loop in the direction shown in figure. Radius of loop is R. What is the magnitude of magnetic field induciton at the centre of the loop?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A current I flows through a closed loop as shown in figure. The magnetic field induction at the centre 0 is
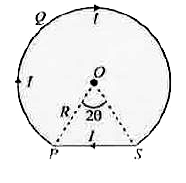 Aμ0I4πRθBμ0I4πR(θ+sinθ)Cμ0I4πR(π−θ+sinθ)Dμ0I4πR(π−θ+tanθ)
Aμ0I4πRθBμ0I4πR(θ+sinθ)Cμ0I4πR(π−θ+sinθ)Dμ0I4πR(π−θ+tanθ) - Question 2 - Select One
A current /is flowing through the loop. The direction of the current and the shape of the loop are as shown in the figure. The magnetic field at the centre of the loop is μ0IR×(MA=R,MB=2R,∠DMA=90∘
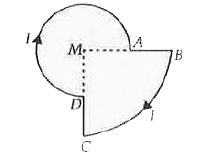 A516 ,but of the plane of the paper.B516, but into the plane of the paper.C716,but out of the plane of the paper.D716,but into the plane of the paper.
A516 ,but of the plane of the paper.B516, but into the plane of the paper.C716,but out of the plane of the paper.D716,but into the plane of the paper. - Question 3 - Select One
A current I is flowing thorugh the loop. The direction of the current and the shape of the loop are as the shown in the figure. The magnetic fild at the centre of the loop is μ0IR times
(MA=R,MB=2R,∠DMA=90∘)
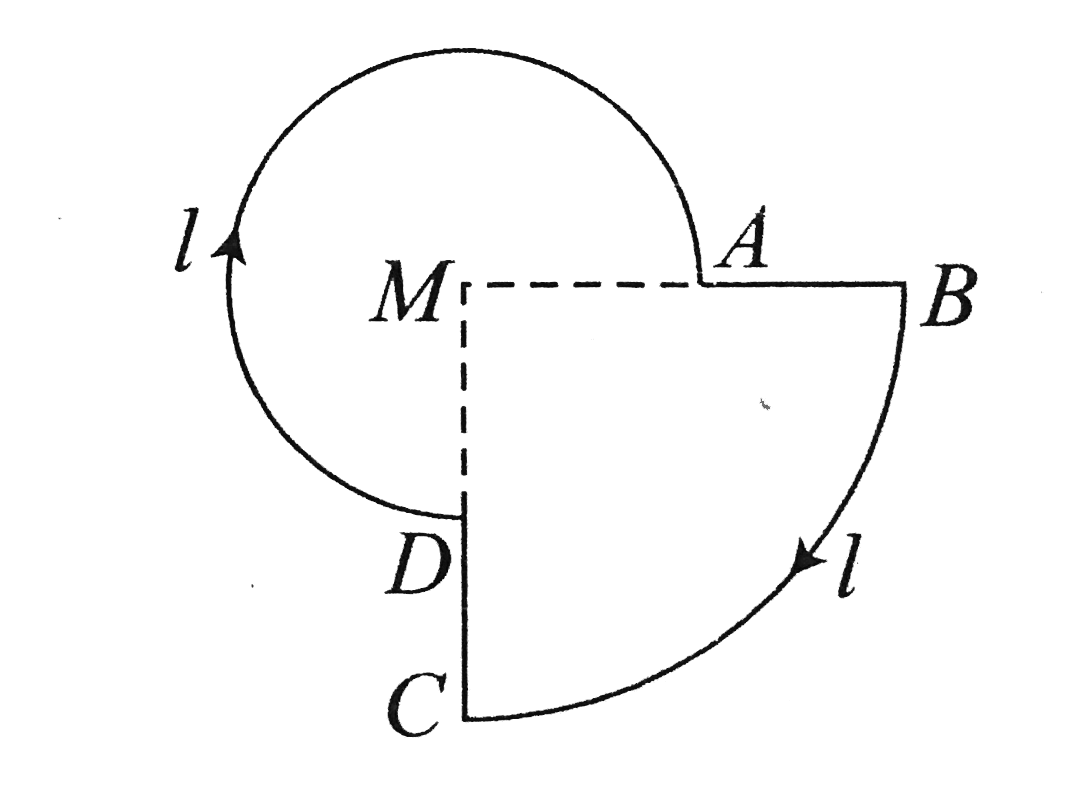 A516, but out of the plane of the paper.B716, but into of the plane of the paper.C516, but into of the plane of the paper.D716, but out of the plane of the paper.
A516, but out of the plane of the paper.B716, but into of the plane of the paper.C516, but into of the plane of the paper.D716, but out of the plane of the paper.
Find the magnetic field at the centre of the circular loop shown in figure.
 View Solution
View SolutionFind the magnetic field at the centre of the circular loop shown in figure.
 View Solution
View SolutionFind the magnetic field at the centre of the circular loop shown in figure.
 View Solution
View SolutionConsider the loop PQRSP, carrying clockwise current i, shown in the figure. The magnitude of magnetic field at the centre O of the curved portion is
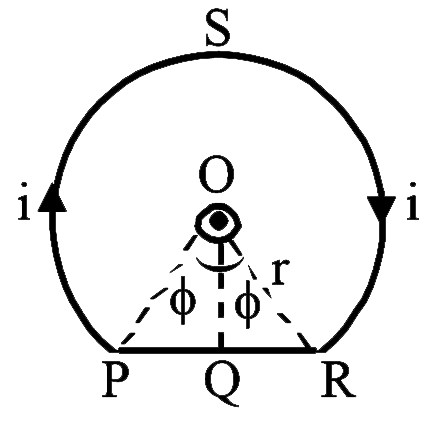 View Solution
View SolutionA circular loop of radius 9.7 cm carries a current 2.3 A. Obtain the magnitude of the magnetic field at the centre of the loop .
View Solution

