Estimate how large can be the aperture opening to work with laws of ray optics using a monochromatic light of wavelength 450 nm, to a distance of around 20 m.
6 mm
3 mm
2 mm
8 mm
The correct Answer is:B
To estimate how large the aperture opening can be to work with the laws of ray optics using monochromatic light of wavelength 450 nm at a distance of around 20 m, we can follow these steps:
Step 1: Understand the Given Values
We are given:
- Wavelength of light, λ=450nm=450×10−9m
- Distance, Zf=20m
Step 2: Use the Formula for Aperture Size
The relationship between the aperture size a, the distance Zf, and the wavelength λ is given by the formula:
Zf=a2λ
We need to rearrange this formula to solve for a:
a2=Zf⋅λ
a=√Zf⋅λ
Step 3: Substitute the Values
Now, substitute the known values into the equation:
a=√20m⋅(450×10−9m)
Step 4: Calculate the Value Inside the Square Root
First, calculate the product:
20⋅450×10−9=9000×10−9m2
Step 5: Take the Square Root
Now, take the square root:
a=√9000×10−9=√9×10−6=3×10−3m
Step 6: Convert to Millimeters
Convert the result from meters to millimeters:
3×10−3m=3mm
Conclusion
The maximum aperture size a that can be used while still adhering to the laws of ray optics is:
3mm
---
We are given:
- Wavelength of light, λ=450nm=450×10−9m
- Distance, Zf=20m
The relationship between the aperture size a, the distance Zf, and the wavelength λ is given by the formula:
Zf=a2λ
We need to rearrange this formula to solve for a:
a2=Zf⋅λ
a=√Zf⋅λ
Now, substitute the known values into the equation:
a=√20m⋅(450×10−9m)
First, calculate the product:
20⋅450×10−9=9000×10−9m2
Now, take the square root:
a=√9000×10−9=√9×10−6=3×10−3m
Convert the result from meters to millimeters:
3×10−3m=3mm
The maximum aperture size a that can be used while still adhering to the laws of ray optics is:
3mm
Topper's Solved these Questions
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assertion reason
8 Videos
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - match column
4 Videos
- WAVE OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Check point
65 Videos
- SOLVED PAPERS 2018BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER
22 Videos
Similar Questions
In a Young's double slit experiment, the slit separation is 1mm and the screen is 1m from the slit. For a monochromatic light of wavelength 500nm, the distance of 3rd minima from the central maxima is
View SolutionIn Young's double-slit experiment, the slit separation is 0.5 mm and the screen is 0.5 m away from the slit. For a monochromatic light of wavelength 500 nm, the distance of 3rd maxima from the 2nd minima on the other side of central maxima is
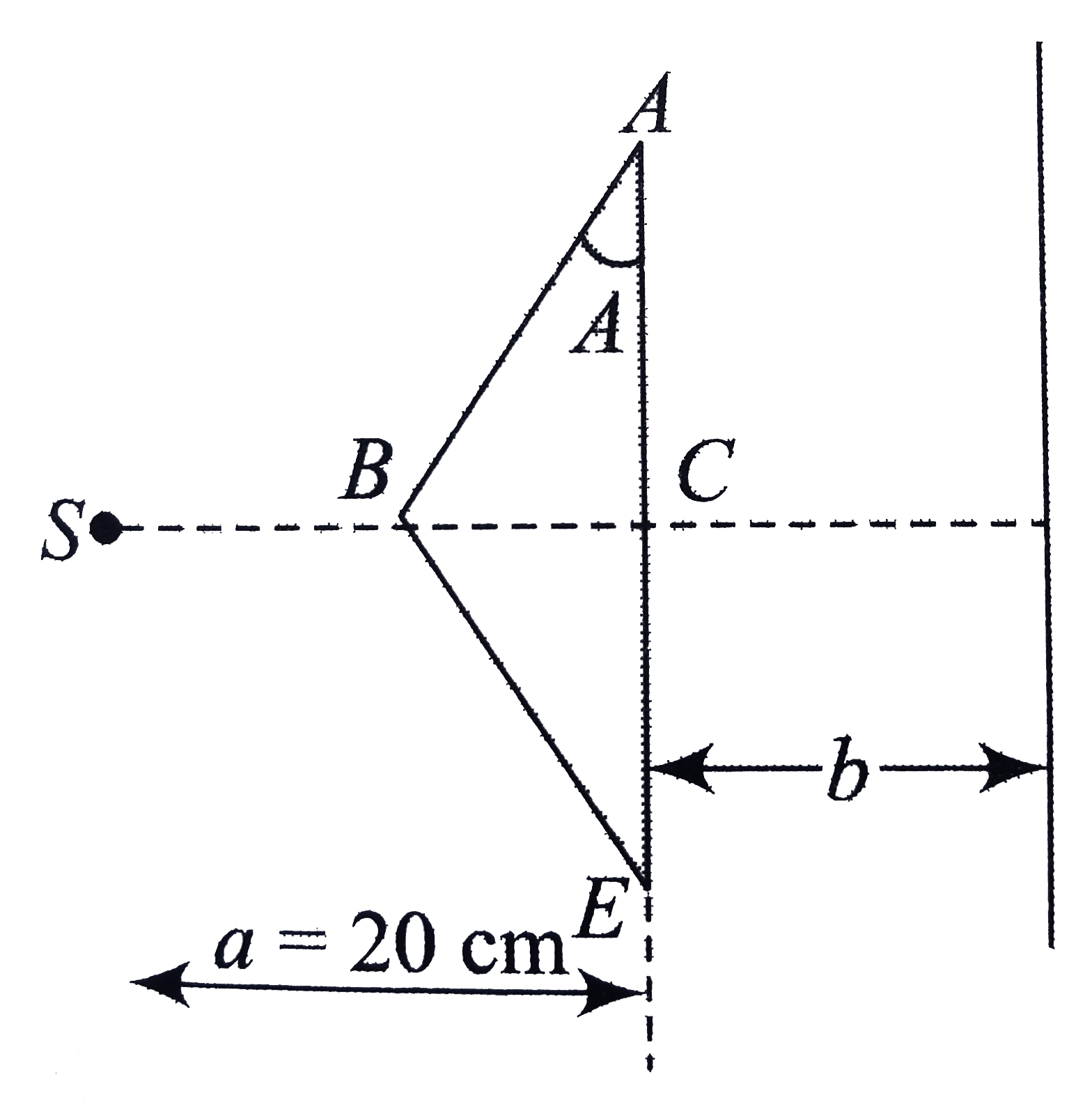
View SolutionTwo small angled transparent prisms (each or refracting angle A=1∘) are so placed that their bases coincide, so that common base is BC. This device is called Fresnel's biprism and is used to obtain coherent sources of a point source S illuminated by monochromatic light of wavelength 6000Å placed at a distance a=20 cm. Calculate the separation between coherent sources. If a screen is placed at a distance b=80 cm. from the device, what ist the finge which of fringes obtained (Refractive index of material of each prism =1.5.)
View SolutionIn the diffration due to single slit experiment, the aperture of the slit is 3 mm. If monochromatic light of wavelength 620 nm in incident normally on the slit, calculate the separation in between the first order minima and the 3rd order maxima on one side of the screen. The distance between the slit and the screen is 1.5 m.
View SolutionA small piece of cesium metal ϕ=1.9eV is kept at a distance of 20 cm form a large metal plate having a charge density of 1.0×10−9Cm−2 on the surface facing the cesium piece. A monochromatic light of wavelength 400nm is incident on the cesium piece. Find the minimum and the maximum kinetic energy of the photoelectrons reaching the large metal plate. Neglect any change in electric field due to the small piece of cesium present.
View SolutionA transparent paper (refractive index = 1.45) of thickness 0.02 mm is pasted on one of the slits of a Young's double slit experiment which uses monochromatic light of wavelength 620 nm. How many fringes will cross through the centre if the paper is removed ?
View SolutionEstimate the distance for which ray optics is good approximation for an aperture of 4mm and wavelength 400nm.
View SolutionEstimate the distance for which ray optics is good approximation for an aperture of 4mm and wavelength 400nm.
View SolutionEstimate the distance for which ray optics is good approximately for an aperture of 2mm and wavelength 500 nm.
View SolutionIn a double slit experiment, the two slits are 1mm apart and the screen is placed 1m away. A monochromatic light of wavelength 500nm is used. What will be the width of each slit for obtaining ten maxima of double slit within the central maxima of single-slit pattern?
View Solution
