Explain thermal conductivity and coefficient of thermal conductivity. A copper bar of thermal conductivity 401 W (mk) has one end at and the other end at . The length of the bar is 0.10 m and the cross-sectional area is . What is the rate of heat conduction along the bar ?
The ability to conduct heat in solids is called thermal conductivity.
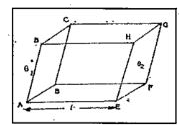
Consider a bar with rectangular cross-section as shown in the figure. The faces ABCD and EFGH are maintained at and respectively Heat passes from one end to the other.
The amount of heat conducted (Q) depends on the following factors.
1) Amount of heat conducted Q is proportional to area of cross-section A perpendicular to flow.
2) Amount of heat flowing through the rod is proportional to temperature difference between the two ends.
3) Amount of heat conducted is proportional to the time of flow.
4) Amount of heat conducted is inversely proportional to the length of the conductor.
From the above laws or
where k is a constant called coefficient of thermal conductivity.
Coefficient of thermal conductivity k: It is defined as the amount of heat conducted normally per sec per unit area of cross-section per unit temperature gradient.
S.I..Unit
Dimensional formula =
Problem:, Thermal conductivity of copper,
Temperature at one end,
Temperature of 2nd end,
Length of copper bar,, Area,
Rate of conduction,
Topper's Solved these Questions
Similar Questions
A thermal conductor is heated at one end. What is the theoretical value of its thermal conductivity in order that the other end also attains the same temperature?
View Solution" A metal rod of area of cross section A has length L and coefficient of thermal conductivity K The thermal resistance of the rod is "
View Solution
Knowledge Check
A metal rod of area of cross section A has length L and coefficient of thermal conductivity K the thermal resistance of the rod is .
ALKABKLLCKALDAKL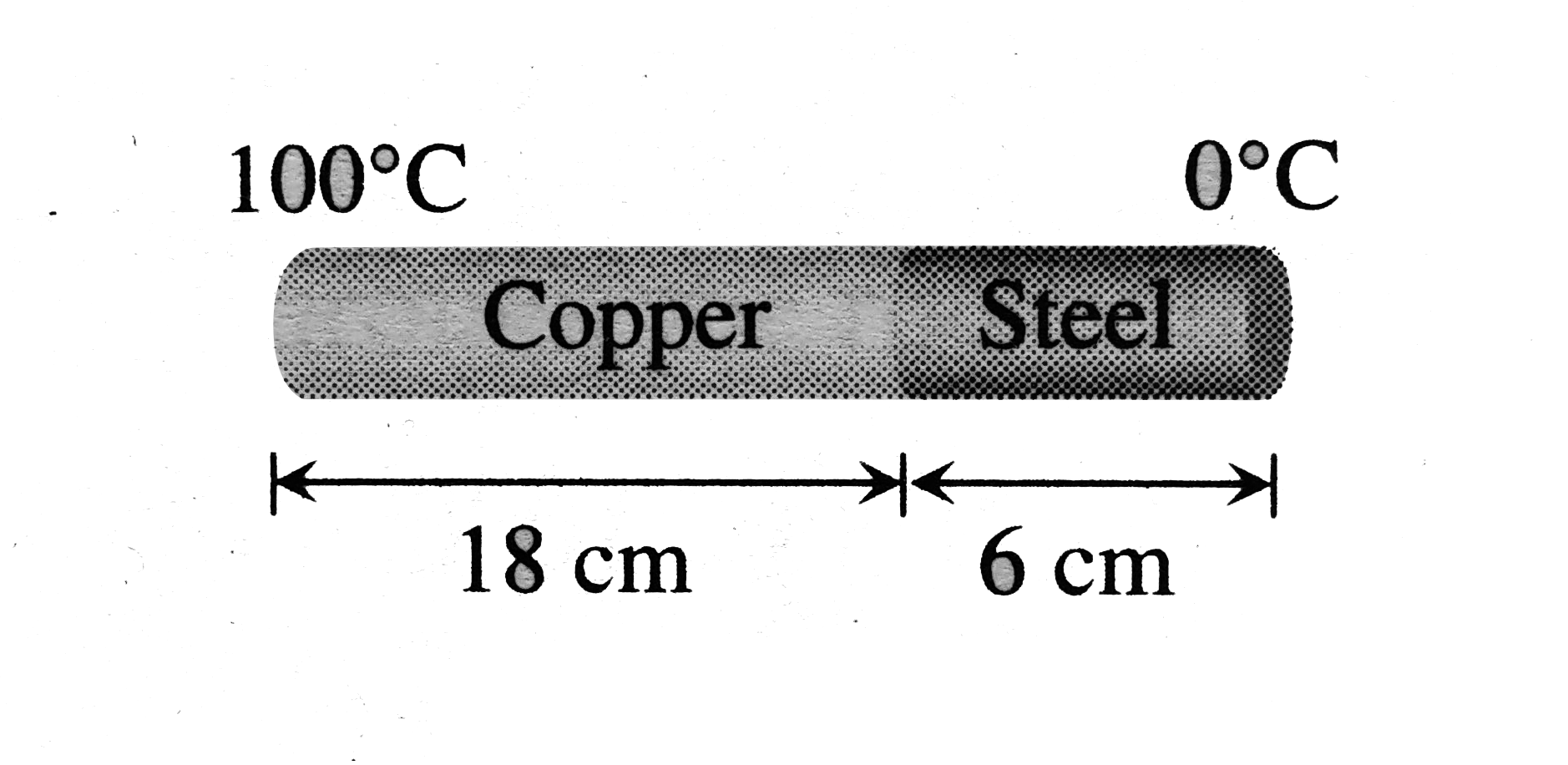
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?A75∘CB67∘CC33∘CD25∘CA cylindrical steel rod of length 0.10 m and thermal conductivity 50W.m−1K−1 is welded end to end to copper rod of thermal conductivity 400W.m−1.K−1 and of the same area of cross section but 0.20 m long. The free end of the steel rod is maintained at 100∘C and that of the copper and at 0∘C. Assuming that the rods are perfectly insulated from the surrounding, the temperature at the junction of the two rods–
A20∘CB30∘CC40∘CD50∘C

The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?
View SolutionA long rod has one end at 0∘C and other end at a high temperature. The coefficient of thermal conductivity varies with distance from the low temperature end as K=K0(1+ax), where K0=102 SI unit and a=1m−1 . At what distance from the first end the temperature will be 100∘C? The area of cross-section is 1cm2 and rate of heat conduction is 1 W.
View Solution
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?
View SolutionThe coefficient of thermal conductivity of copper is nine times of steel in the composite cylindrical bar shown in the figure. What is the temperature at the junction of copper & steel?

View SolutionA long rod has one end at 0∘C and other end at a high temperature. The coefficient of thermal conductivity varies with distance from the low temperature end as K=K0(1+ax), where K0=102 SI unit and a=1m−1 . At what distance from the first end the temperature will be 100∘C? The area of cross-section is 1cm2 and rate of heat conduction is 1 W.
View Solution
