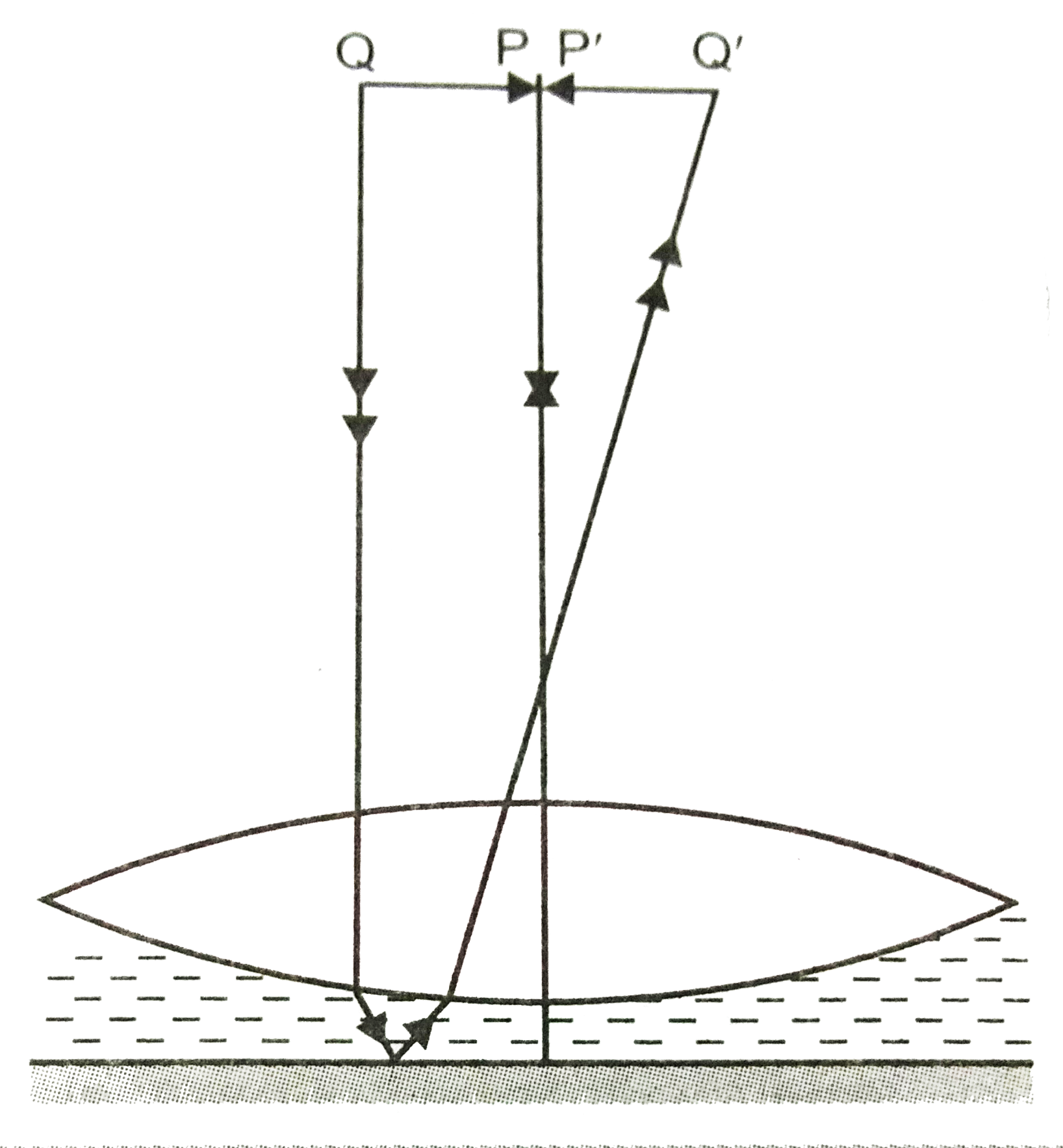
Fig. shows an equiconvex lens (of refractive index 1.5) in contact with a liquid layer on top of a plane mirror. A small needle with its tip on the principal axis is moved along the axis until its inverted image is found at the position of the needle. The distance of the needle from the lens is measured to be 45.0cm. The liquid is removed and the experiment is repeated. The new distance is measured to be 30.0cm. What is the refractive index of the liquid ?
Focal length of the convex lens, f1=30cm
The liquid acts as a mirror. Focal length of the liquid = f2
Focal length of the system (convex lens+liquid), f=45 cm
For a pair of optical systems placed in contact, the equivalent focal length is given as:
1f=1f1+1f2
1f2=1f−1f1
= 145−130=−190
∴f2=−90cm
Let the refractive index of the lens be μ1 and the radius of curvature of one surface be R. Hence, the radius of curvature of the other surface is -R
R can be obtained using the relation:
1f1=(μ1−1)(1R+1−R)
130=(1.5−1)(2R)
∴R=300.5×2=30cm
Let the refractive index of the liquid.
Radius of curvature of the liquid on the side of the lens, R=-30cm
The value of μ2 can be calculated using the relation.
1f2=(μ2−1)[1−R−1∞]
190=(μ2−1)[130−0]
μ2−1=13
∴μ2=43=1.33
Hence, the refractive index of the liquid is 1.33.
Topper's Solved these Questions
- BOOK - NCERTCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Exercise32videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - NCERTCHAPTER - NUCLEIEXERCISE - Exercise31videos
NUCLEI
- BOOK - NCERTCHAPTER - SEMICONDUCTOR ELECTRONICS MATERIALS DEVICES AND SIMPLE CIRCUITSEXERCISE - Exercise15videos
SEMICONDUCTOR ELECTRONICS MATERIALS DEVICES AND SIMPLE CIRCUITS
Similar Questions
Fig. 9.12 shows an equiconvex lens (of refractive index 1.50) in contact with a liquid layer on top of a plane mirror. A small needle with its tip on the principal axis is moved along the axis until its inverted image is found at the position of the needle. The distance of the needle from the lens is measured to be 45.0 cm. The liquid is removed and the experiment is repeated. The new distance is measured to be 30.0 cm. What is the refractive index of the liquid ?
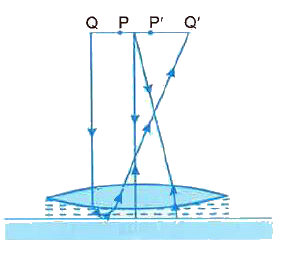 View Solution
View SolutionAn equiconvex lens, with redii of curvature of magnitude 10cm each, is put over a liquid layer poured on top of a plane mirror. A small needle, with its tip on the principal axis of the lens, is moved along the axis until its inverted real image coincides with the needle itself. The distance of the needle, from the lens, is measured to be 15cm .
On removing the liquid layer, and repeating the experiment the distance is measured to be 10cm . Given that the two values of the distance measured represent the force length values in the two cases, calculate the refractive index of the liqiud.View SolutionAn equiconvex lens with radii of curvature of magnitude R each is put over a liquid layer poured on top of a plane mirror. A small needle, with its tip on the principal axis of the lens, is moved along the axis until its inverted real image coincides with the needle itself. The distance of the needle from the lens is measured to be 'a'. On removing the liquid layer and repeating the experiment the distance is found to be 'b'.
Given that the two values of distances measured represent the focal length values in the two cases, obtain a formula for the refractive index of the liquid.
 View Solution
View SolutionA symmetric biconvex lens of radius of curvature R and made of glass of refractive index 1.5, is placed on a layer of liquid placed on top of a plane mirror as shown in the figure. An optical needle with its tip on the principal axis of the lens is moved along the axis until its real, inverted image coincides with the needle itself. The distance of the needle from the lens is measured to be x. On removing the liquid layer and repeating the experiment, the distance is found to be y. Obtain the expression for the refractive index of the liquid in terms of x and y.
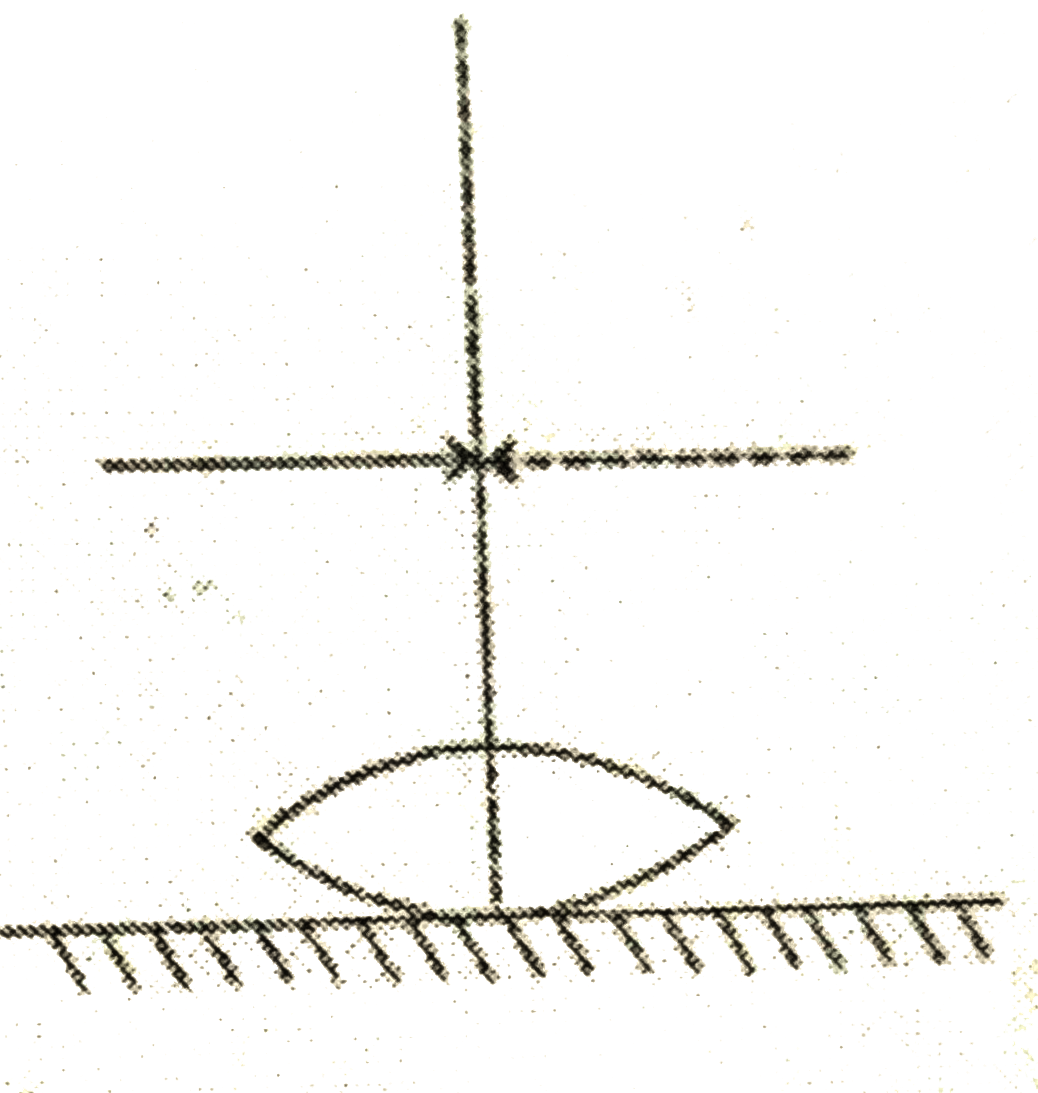 View Solution
View SolutionA synunetdc bionvex fens of radius of curvature Rand made of glass of refractive index 1.5, is placed on a placed on top of a plane mirror as shown in the figure.An optical needle with with its tip on the principal axis of the lens is moved along the axis until its real, inverted images conicides with the needle itself. The distance from the lens in measured to be x. On removing the liquid layer and repeating the experiment, the distance is found to be y . Obtain the expression for the refractive index of the liquid in terms of x and y.
 View Solution
View SolutionAn equiconvex lens with radii of curvature of magnitude R each is put over a liquid layer poured on top of a plane mirror. A small needle, with its tip on the principal axis of the lens, is moved along the axis until its inverted real image coincides with the needle itself. The distance of the needle from the lens is measured to be 'a'. On removing the liquid layer and repeating the experiment the distance is found to be 'b'.
Given that the two values of distances measured represent the focal length values in the two cases, obtain a formula for the refractive index of the liquid.
 View Solution
View SolutionAn equiconvex lens with radii of curvature of magnitude R each is put over a liquid layer poured on top of a plane mirror. A small needle, with its tip on the principal axis of the lens, is moved along the axis until its inverted real image coincides with the needle itself. The distance of the needle from the lens is measured to be 'a'. On removing the liquid layer and repeating the experiment the distance is found to be 'b'.
Given that the two values of distances measured represent the focal length values in the two cases, obtain a formula for the refractive index of the liquid.
 View Solution
View SolutionA glass lens of refractive index 1.45 disappears when immersed in a liquid. What is the value of refractive index of the liquid ?
View SolutionA needle 10 cm long is placed along the axis of a convex lens of focal length 10 cm such that the middle point of the neddle is at a distance of 10 cm from the lens. Find the length of the image of the neddle.
View SolutionAn object 50cm tall is placed on the principal axis of a convex lens. Its 20cm tall image is formed on the screen placed at a distance of 10cm from the lens. Calculate the focal length of the lens.
View Solution

