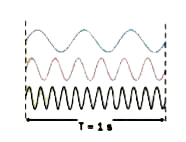
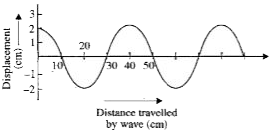
Figure given shows a sinusoidal wave on a string. If the frequency of the wave is 150 Hz, what is the velocity and wavelength of the given wave?
0.04m,10ms−1
0.06m,12ms−1
0.08m,10ms−1
0.08m,12ms−1
The correct Answer is:D
Given : Frequency of the wave, υ = 150 Hz

From the fig ure,52λ=20cm
∴ Wavelength of the wave,
λ=405cm=8cm=8×10−2m=0.08m
Velocity of the wave,
v=υλ=(150s−1)(8×10−2m)=12ms−1
Topper's Solved these Questions
- WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WAVESEXERCISE - HOTS
8 Videos
- WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WAVESEXERCISE - EXEMPLER
10 Videos
- UNITS AND MEASUREMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - UNITS AND MEASUREMENTSEXERCISE - Assertion And Reason
15 Videos
- WORK , ENERGY AND POWERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
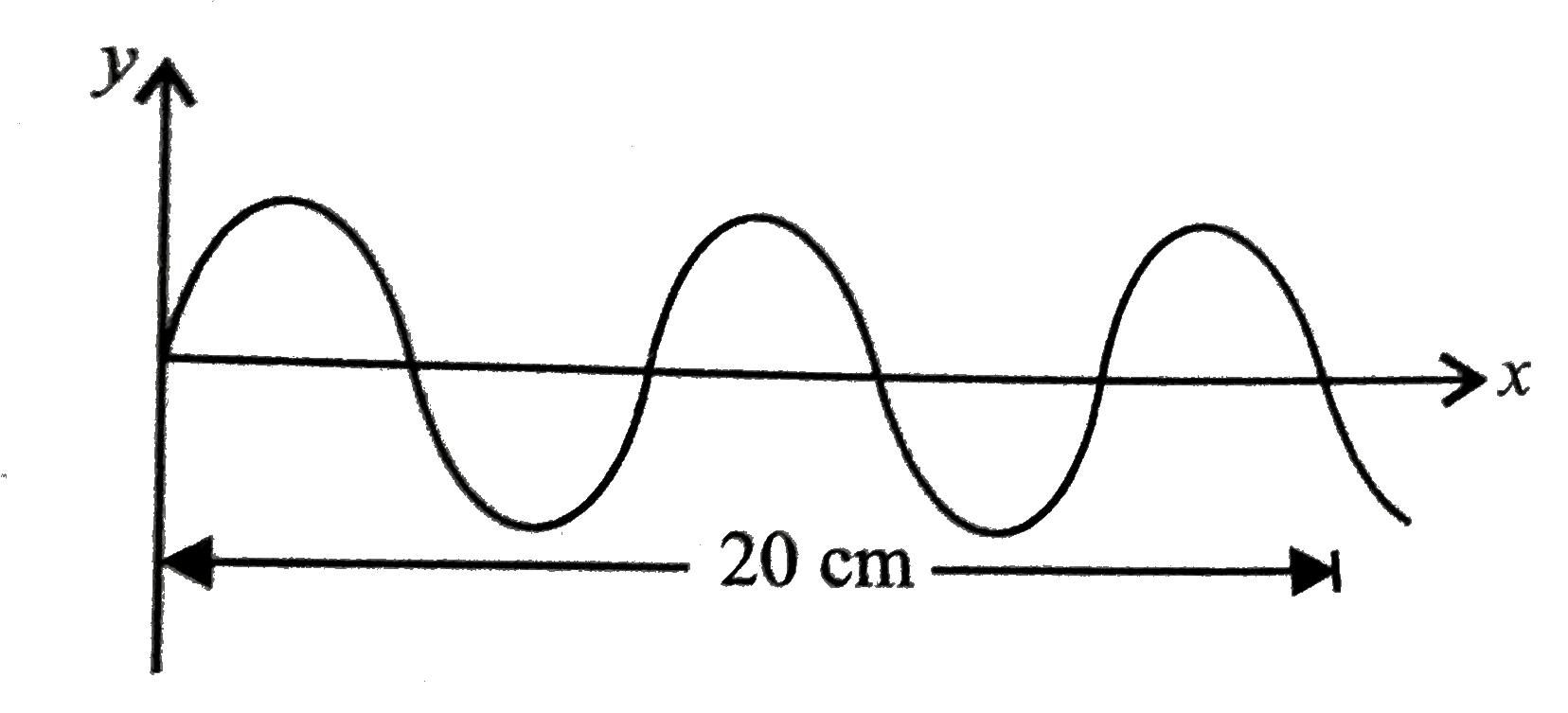
What is the wavelength of wave shown in given figure ?
 A0.6 mB0.3 mC0.08 mD4 cm
A0.6 mB0.3 mC0.08 mD4 cmFigure shows a sinusoidal wave at a given instant.
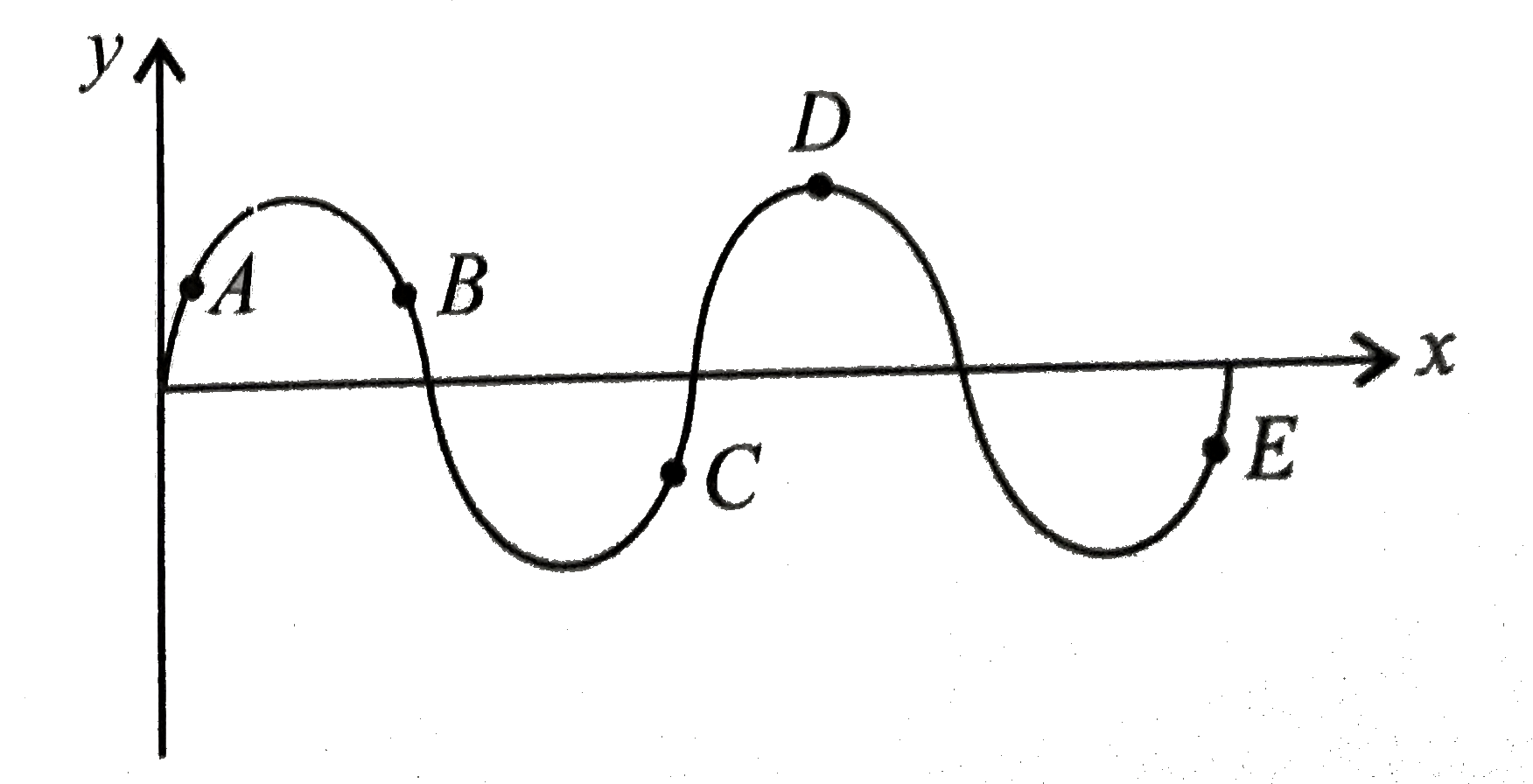
Which points are in phase?AA,BBB,CCB,DDC,E
Frequency of a wave is 6×1015 Hz. The wave is
View SolutionThe frequency of sound is 100 Hz and wavelength is 3m. Calculate the velocity of the wave.
View SolutionA wave travelling along the length of a string is shown in Fig. 14.4.12. Using the scale on the given axis, find
(i) The amplitude of the wave
(ii) The wavelength of the wave
(iii) If the frequency of the wave is 10 Hz, What is the speed of the wave?
(iv) What is the initial phase of the wave ?
(v) What is the phase difference between two points separated by a distance 10 cm ?
(vi) If this wave is made to travel through water what is the wavelength of the wave, if the speed of, the waves in water is 10 m/s.
View SolutionDefine wavelength and frequency of a wave.
View SolutionThe string shown in the figure has a sinusoidal wave travelling towards right. The frequency of the wave is n. the correct statement (s) is/are.
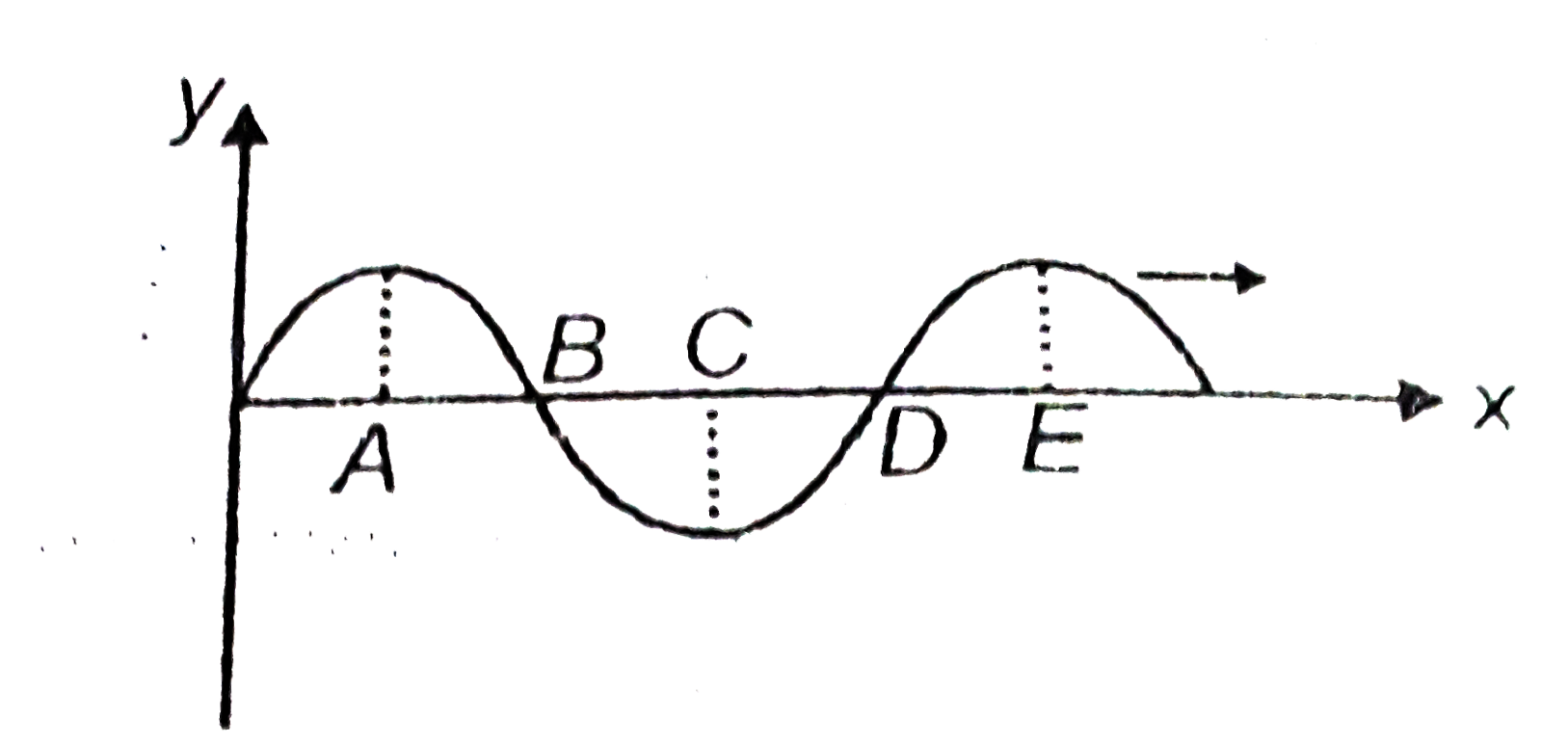
View SolutionDerive an expression of wave velocity in terms of frequency and wavelength of the wave.
View Solution