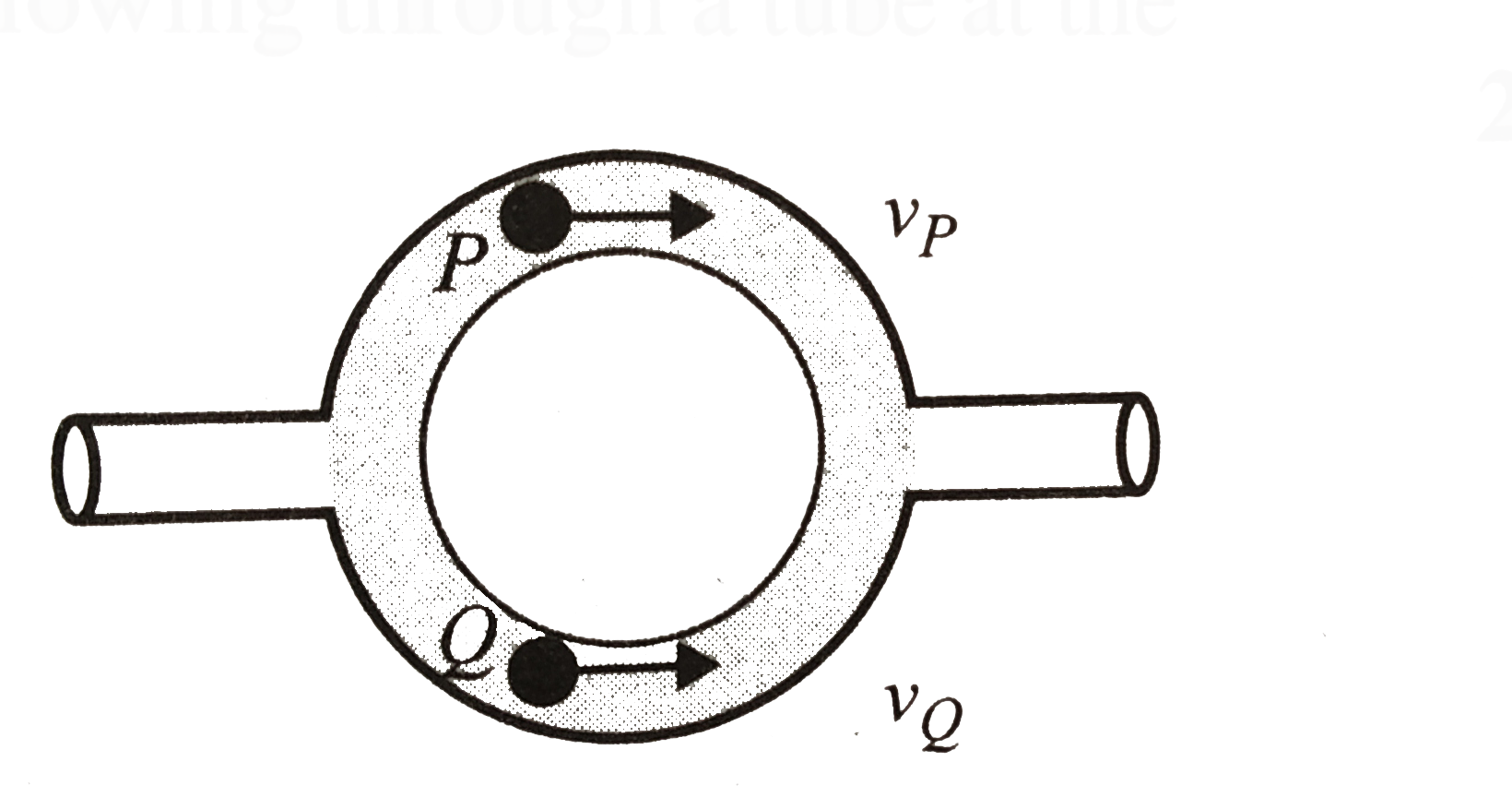
Figure shows a liquid flowing through a tube at the rate of $0.1 m^{3} / s$ . The tube is branched into two semicircular tubes of cross-sectional area $A / 3$ and $2 A / 3$ . The velocity of liquid at $Q$ is (the cross section of the main tube is $A = 10^{- 2} m^{2}$ and $v_{p} = 20 m / s$ )

A

$5 m / s$

B

$30 m / s$

C

$35 m / s$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

From a equationof continuity
Rate $= r = \frac{A}{3} V_{p} + (A - \frac{A}{3}) V_{Q}$
$∴ V_{P} + 2 V_{Q} = \frac{3 r}{A} = \frac{3 \times 0.1}{10^{- 2}} = 30 m / s$
As $V_{P} + 2 V_{Q} = 5 m / s$

|

Updated on:21/07/2023

Class 11 PHYSICS FLUID MECHANICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Flow rate of blood through a capillary of cross - sectional are of $0.25 m^{2}$ is $100 c m^{3} / s$ . The velocity of flow of blood iis
A1 mm/s
B0.2 mm/s
C0.3 mm/s
D0.4 mm/s
Question 2 - Select One
If cross- sectional area of limb I is $A_{1}$ and that of limb II is $A_{2}$ then velocity of the liquid in the tube will be, (cross- sectional area of tube is very small)

A $\sqrt{2 g (x - y)}$
B $\frac{A_{1}}{A_{2}} \sqrt{2 g (x - y)}$
C $\frac{A_{2}}{A_{1}} \sqrt{2 g (x - y)}$
DNone of these
Question 3 - Select One
Water is flowing through a capillary tube at the rate of $20 \times 10^{- 6} m^{3} / s$ . Another tube of same radius and double the length is connected in series to the first tube. Now the rate of flow of water in $m^{3} s^{- 1}$ is
A $10 \times 10^{- 6}$
B $3.33 \times 10^{- 6}$
C $6.67 \times 10^{- 6}$
D $20 \times 10^{- 6}$

CENGAGE PHYSICS-FLUID MECHANICS-Single Correct

Home
Ask Doubt
Profile