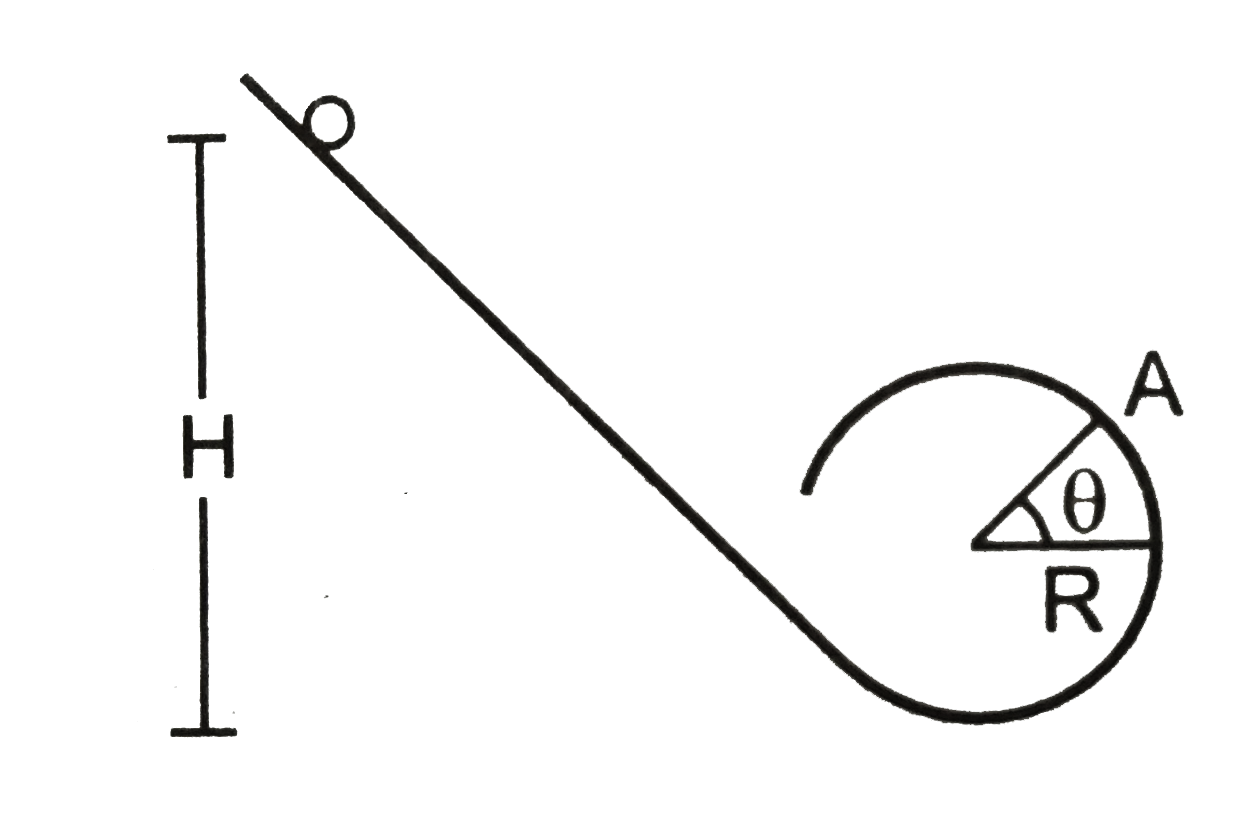
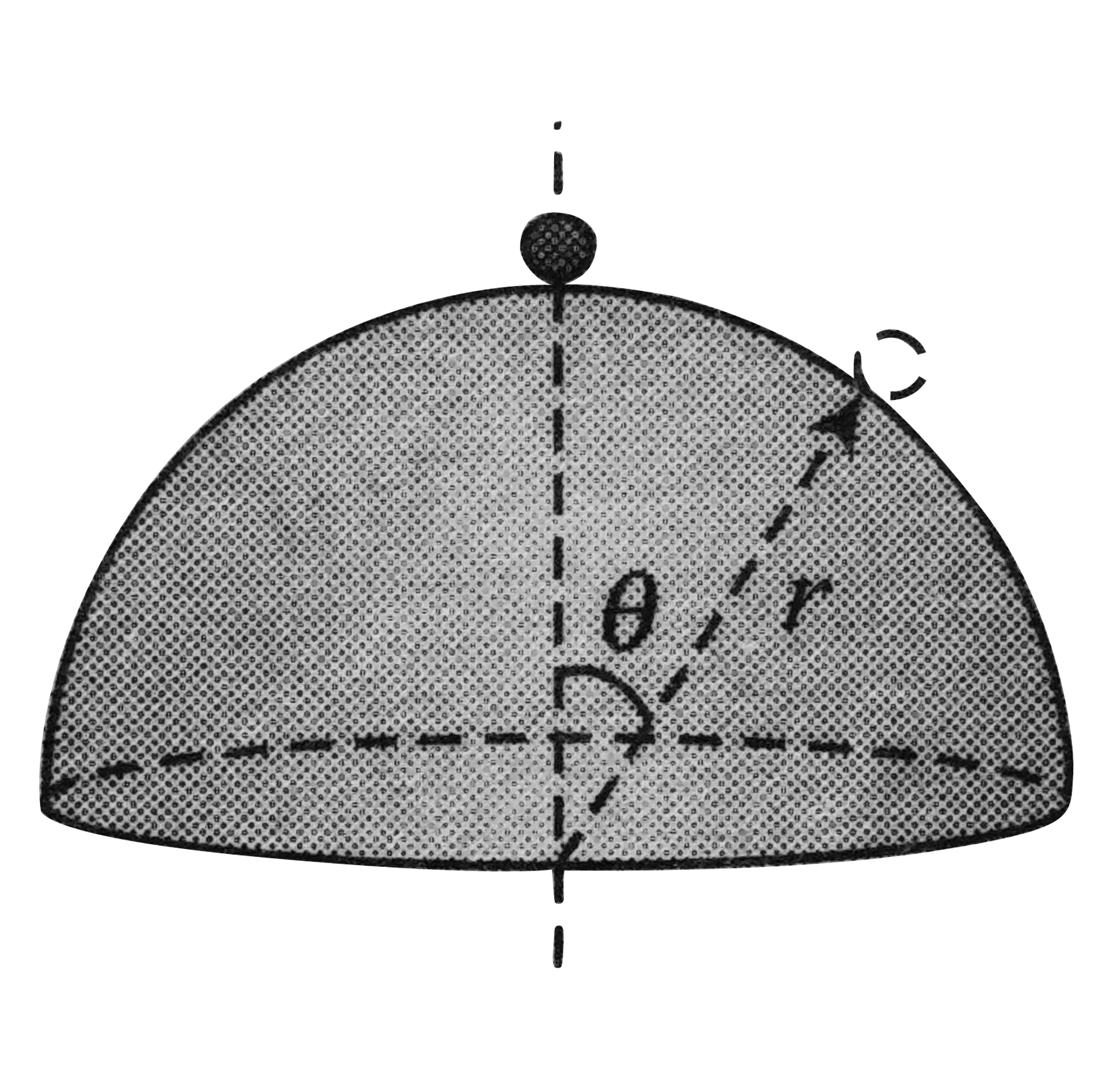
Figure shows a smasll sphereical bal of mass m rolling down the loop track. Thebasll is released on the linear portion at a verticla height H from the lowest point. The circular part show has a radius R.
a. find the kinetic energy of the ball when it is at a point where the radius makes an angle θ with the horizontal.
Find the radial and the tangential accelerations of the cente when the ball is at A. c. find the bnormal force and the frictionasl force acting on the ball if H=60 cm, R=10cm θ=0 and m=70 fg.
The correct Answer is:A, B, C, D
a. Total kinetic energy
y=12mv2+12Iω2
therefore, according to the question
mgH=12mv2+12Iω2+mgR(1+sinθ)
→mgH−mgR(1+sinθ)
=12mv2+12Iω2
→12mv2+12Iω2=mg(H−R−Rsinθ)
b. To find the acceleratiohn components,
→12mv2+12Iω2=mg(H−R−Rsinθ)
→k710mv2=mg(H−R−Rsinθ)
→v2=107g(H−R−Rsinθ)
→v2=107g(H−R−Rsinθ).....i
→v2R=107(g[H−R)−Rsinθ])R
→v2R=(10g[(H−R)−Rsinθ)])R
−2vdvdt=−(107)gRcosθdthηdt
→ωRdvdt=−(57)gRcosθdthηdt
→dvdt=−(57)gcosθ
→ tangential acceleration
c. Normal force at thea=0
mv2R=(70100)×(107)×10{(0.6−0.1)0.1}
=5N
Friction force:
=f=mg−ma
−f=m(g−a)
m(10−57x10)
=0.07(10−57×10)
=1100(70−50)=0.2N
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective 2
15 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
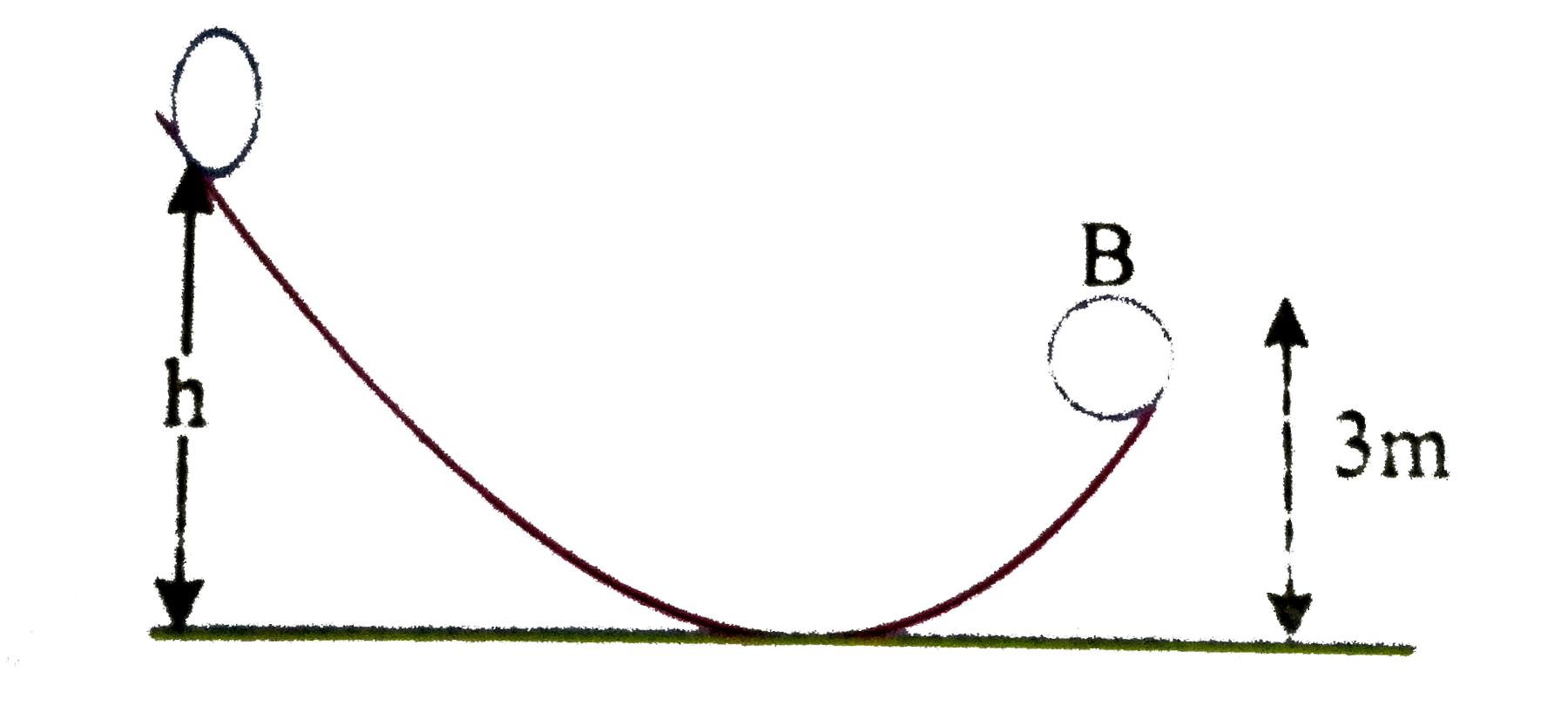
A ball leaves the track at B which is at 3m height from bottom most point of the track. The ball further rises upto 4m height from the bottom most point before falling down. Find h (in m), if the track at B makes an angle 30∘ with horizontal.
 .
.
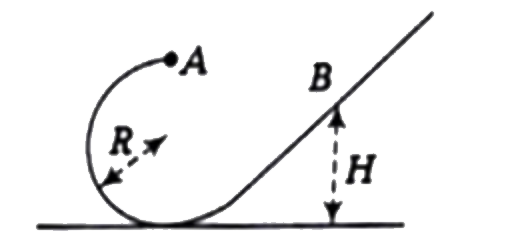
View SolutionInside a smooth spherical shell of the radius R a ball of the same mass is released from the shown position (Fig.) Find the distance travelled by the shell on the horizontal floor when the ball comes to the lowest point of the shell.
View Solution
Knowledge Check
A particle is projected with a speed u at an angle θ to the horizontal. Find the radius of curvature.
At the point where the particle is at a highest half of the maximum height H attained by it.A2u2(1+cos2θ)3/2g2√2cosθBu2(1+cos2θ)3/2g2√2cosθCu2(1−sin2θ)3/2g2√2cosθDu2(1−tan2θ)3/2g2√2cosθA small bead of mass M slides on a smooth wire that is bent in a circle of radius R. It is released at the top of the circular part of the wire (point A in the figure) with a negligibly small velocity. Find the height H where the bead will reverse direction
 ARB2 RC3R2D5R2
ARB2 RC3R2D5R2A solid cylinder of mass M and radius R rolls without slipping down an inclined plane making an angle 6 with the horizontal. Then its acceleration is.
A√34ghB√43ghC√4ghD√2gh
Figure below shows a small mass connected to a string, which is attached to a vertical post. If the ball is released when the string is horizontal as shown, the magnitude of the total acceleration (including radial and tangential) of the mass as a function of the angle Θis

View SolutionA point mass m starts from rest and slides down the surface of a frictionless hemisphere of radius r as shown figure. Measure angle from the vertical and potential energy from the top. Find
a. Find the changes in potential energy of the mass with angle
b. Find the kinetic energy as a function of angle
c. Find the radial and tangential acceleration as a function of angle
d. Find the angle at which the mass files off the hemisphere
e. If there is friction between the mass and hemisphere, does the mass fly off at a greater or lesser angle than in part (d) ?
View Solution
A ring of mass m is rolling without slipping with linear speed v as shown in figure. Four particles each of mass m are also attached at points A, B , C and D find total kinetic energy of the system.
View SolutionA small sphereical ball is released from a point at a height h on a rough track shown in figure. Assuming that it dow not slip anywhere, find its linear speed when it rolls on the horizontal part of the track.

View SolutionA ring of mass m and radius R is rolling down on a rough inclined plane of angle θ with horizontal. Plot the angular momentum of the ring about the point of contact of ring and the plane as a function of time
View Solution
