Figure. shows two parallel and coaxial loops. The smaller loop (radius r) is above the larger loop (radius R), by distance x>>R. The magnetic field due to current i in the larger loop is nearly constant throughout the smaller loop. Suppose that x is increasing at a constant rate of dx/dt=v.
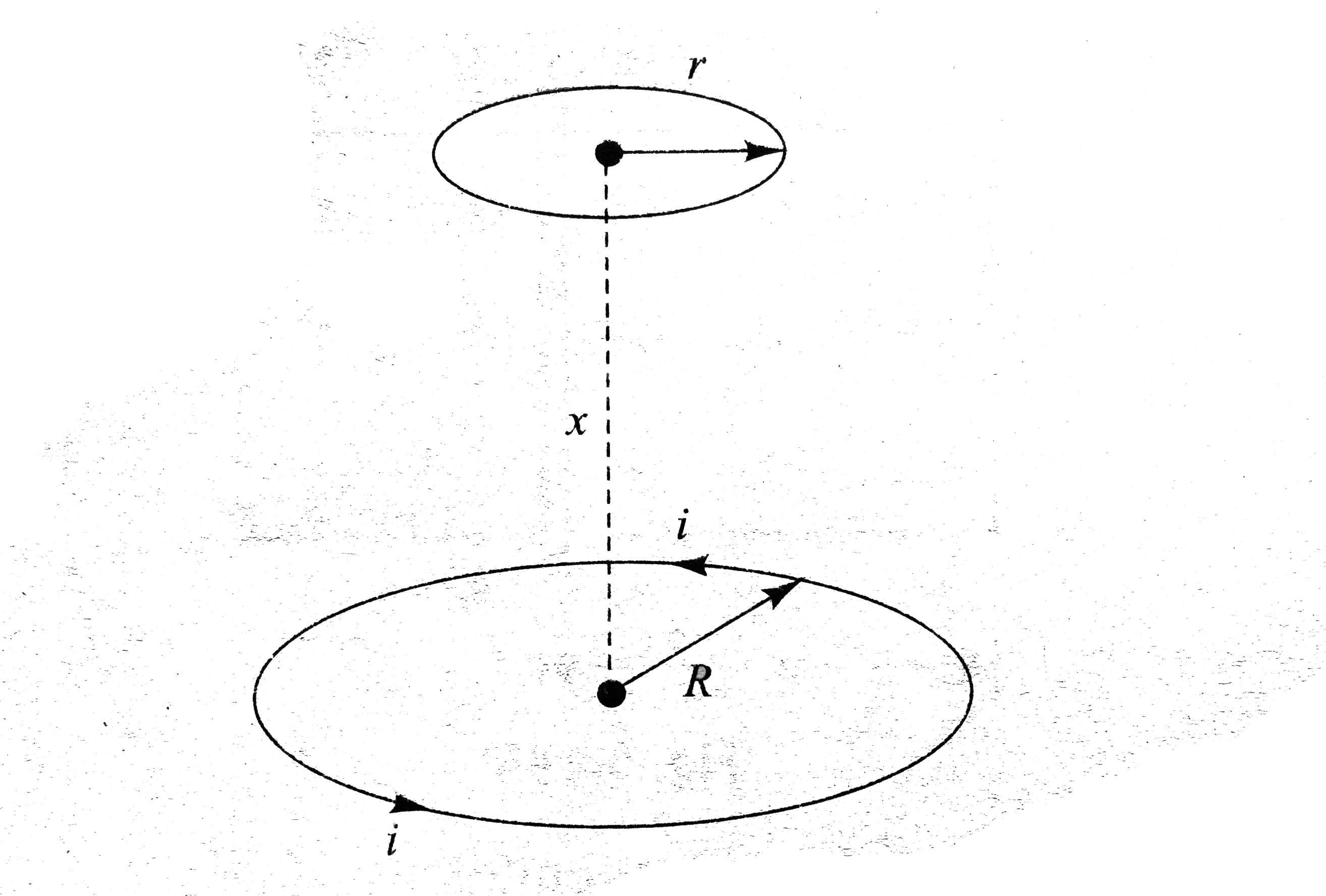
Determine the magneitc flux through the smaller loop as a function of x.
(a) μ0iR2πr2x3
(b) μ0iR2πr22x3
( c) 2μ0iR2πr2x3
( d) √2μ0iR2πr2x3
The correct Answer is:B
(b)Magnetic field on the axis of a circular coil is given by
B=μ0iR22(R2+x2)2
Since x>>R, therefore, nagnetic field at the centre of the smaller loop is
B≈μ0πiR2r22x3 ltbr> Flux linked with coil is
ϕ=B(πr2)=μ0πiR2r22x3
From Faraday's law we have
E=−dϕdt=32μ0πiR2r2x4v
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Integer
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Archives Fill In The Blanks
3 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercises Asserton - Reasoning
8 Videos
- ELECTROMAGENTIC INDUCTIONBOOK - CENGAGE PHYSICSCHAPTER - ELECTROMAGENTIC INDUCTIONEXERCISE - QUESTION BANK
40 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICSCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
Similar Questions
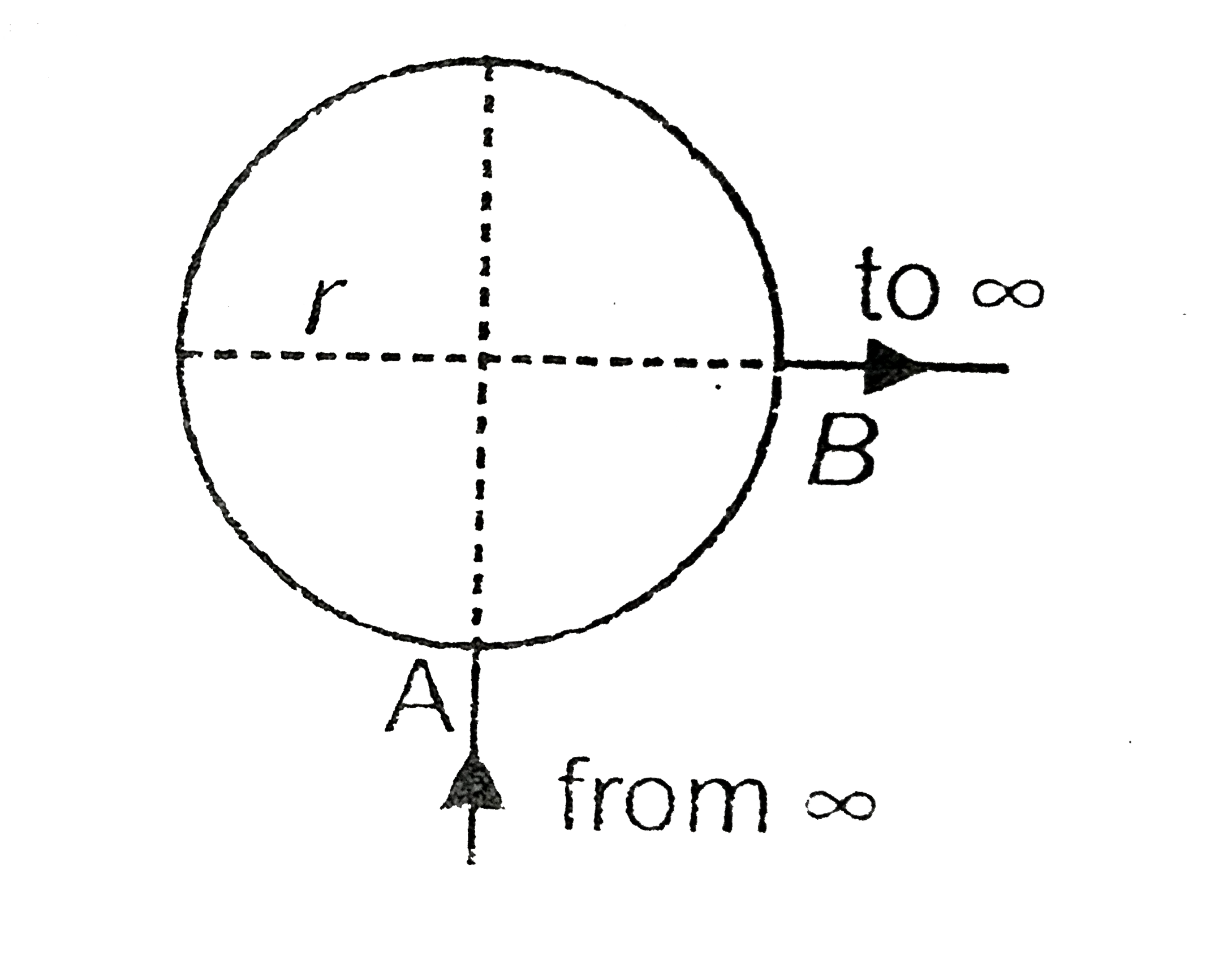
In the figure, the current l enters the circular loop of uniform wire of radius r at A and leaves it at B. The magnetic field of the centre of circular loop is
View SolutionA circular loop of radius R carries a current I. Another circular loop of radius r(ltltR) carries a current I. The plane of the smaller loop makes an angle of 30^@ with that of the larger loop. The planes of the two circles are at right angle to each other. Find the torque acting on the smaller loop.
View Solution
Knowledge Check
Figure 3.188 shows two parallel and coaxial loops. The smaller loop (radius r) is above the larger loop (radius R), by distance x>>R. The magnetic field due to current i in the larger loop is nearly constant throughout the smaller loop. Suppose that x is increasing at a constant rate of dx/dt=v.
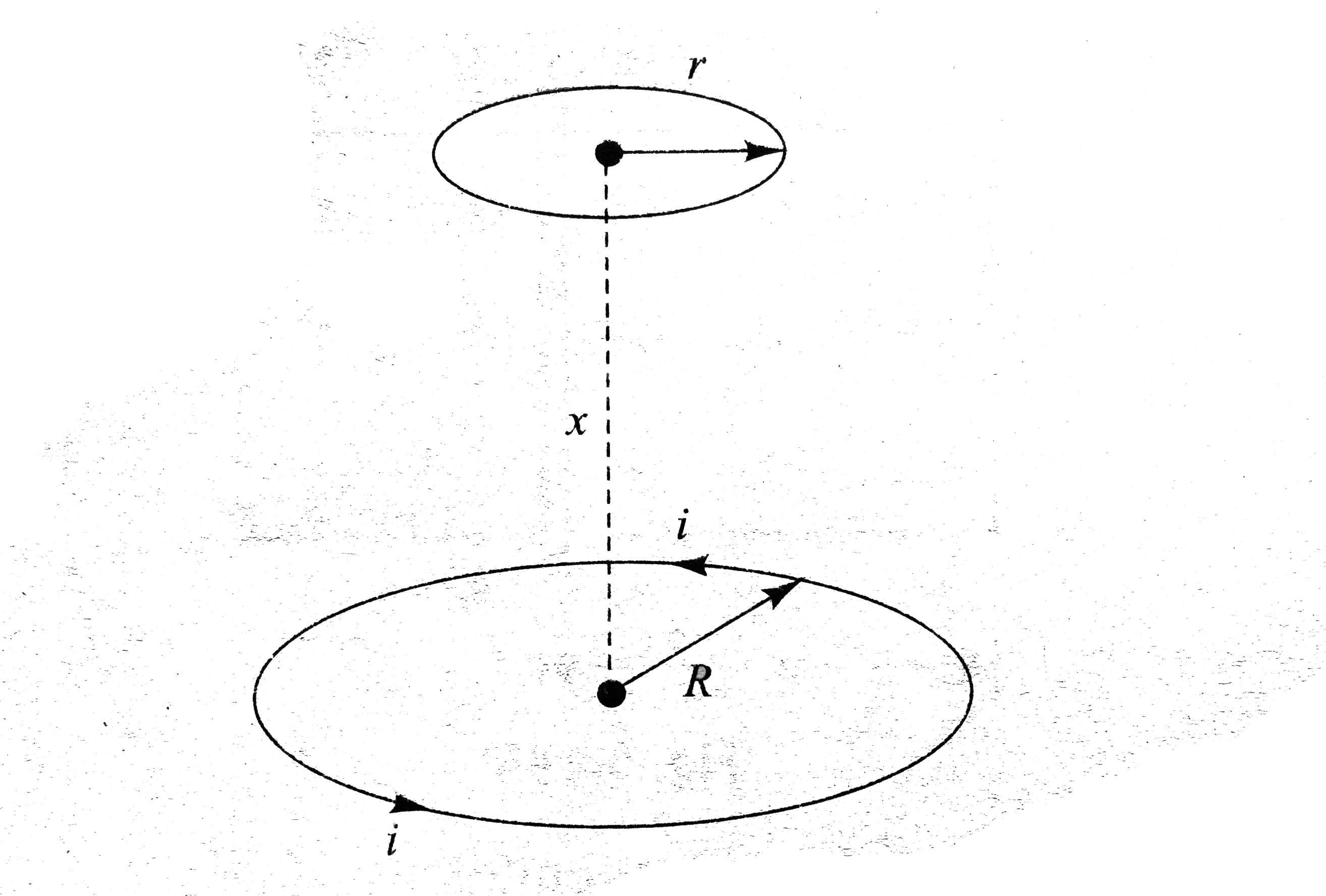
The induced emf in the smaller loop isA(a) μ0πiR2r2x4vB(b) μ0πiR2r22x4vC( c) 32μ0πiR2r2x4vD(d) μ0πiR2r23x4vWhat will be magnetic field at centre of current carrying circular loop of radius R?
Aμ0I4πRBμ0I2πRCμ0I2RDzeroA circular loop of radius r, carrying a current I lines in y - z plane with its centre at the origin. The net magnetic flux through the loop is
Adirectly proportional to r.BzeroCinversely proportional to r.Ddirectly proportional to I.
A very small circular loop of radius a is initially (at t = 0) coplanar and concentric with a much larger fixed circular loop of radius b. A constant current I flows in the larger loop. The smaller loop is rotated with a constant angular speed ω about the common diameter. The emf induced in the smaller loop as a function of time t is
View SolutionA very small circular loop of radius a is initially (at t = 0) coplanar and concentric with a much larger fixed circular loop of radius b. A constant current I flows in the larger loop. The smaller loop is rotated with a constant angular speed ω about the common diameter. The emf induced in the smaller loop as a function of time t is
View SolutionThe linear loop has an area of 5×10−4m2 and a resistance of 2Ω. The larger circular loop is fixed and has a radius of 0.1m. Both the loops are concentric and co-planner. The smaller loop is rotated with an angular velocity ωrads−1 about its dismeter. The magnetic flux with the smaller loop is

View SolutionA long straight conductor carrying a current i is bent to form an almost complete circular loop of radius r on it. The magnetic field at the centre of the loop
View SolutionA current I flows along a round circular loop of radius R. Find the line integration of magnetic field along the axis of the loop from center to ∞
View Solution
