Find the centre of mass of a uniform :
(a) half-disc, (b) quarter-disc. Solution in Gujrati
M = mass of half disc
R = radius of half disc
Mass per unit area of half disc
μ=M12πR2=2MπR2

(a) Suppose, half disc consists of many semicircular rings of mass dm and thickness dr from r=0 to r=R.
Area of semicircular ring of radius,
r=da=122πr×dr=πrdr
∴ Mass of this ring, dm=da×μ
dm=πrdr×2MπR2
dm=2MR2rdr
If (x,y) are co-ordinates of centre of mass of this element, then (x,y)=(0,2rπ)
Therefore, x=0andy=2rπ
Let xCMandyCM be the co-ordinates of the centr of mass of the semicircular disc.
Then, xCM=1MR∫0xdm=1MR∫00dm=0
∴yCM=1MR∫0ydm=1MR∫02rπ×(2MR2rdr)
4πR2R∫0r2dr=4πR2[r33]R0
=4πR2×(R33−0)=4R3π
∴ Centre of mass of the semicircular disc,
(0,4R3π)
(b) Centre of mass of a uniform quarter disc.
Mass per unit area of the quarter disc,
=MπR24=4MπR2

Using symmetry.
For a half-disc along y-axis centre of mass will be at x=4R3π
For a half-disc along x-axis centre of mass will be at x−4R3π
Hence, for the quarter disc centre of mass,
=(4R3π,4R3π)
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SECTION-E MULTIPLE CHOICE QUESTIONS105videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SECTION-F QUESTIONS FROM MODULE22videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SECTION-D NCERT EXEMPLAR SOLUTIONS (SHORT ANSWER TYPE QUESTIONS)4videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
- BOOK - KUMAR PRAKASHANCHAPTER - QUESTIONS ASKED IN JEE - 2020 EXERCISE - Question16videos
QUESTIONS ASKED IN JEE - 2020
- BOOK - KUMAR PRAKASHANCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Question Paper (Section - D) (Answer following in brief :) Each carry 4 marks1videos
THERMAL PROPERTIES OF MATTER
Similar Questions
Peak value divided by the rms value gives us
10:09View SolutionIf I1 is the moment of inertia of a uniform rod about an axis perpendicular to its length and passing through its one end. Now ring formed by bending the rod, if the moment of inertia about the diameter of ring I1 then I1I2 = ……….
02:31View SolutionThe vector sum of a system of non-collinear forces acting on a rigid body is given to be non-zero. If the vector sum of all the torques due to the system of forces about a certain point is found to be zero, does this mean that it is necessarily zero about any arbitrary point?
03:12View SolutionA non-isotropic solid metal cube has coefficient of linear expansion as 5×10−5/∘C along the x-axis and 5×10−6/∘C along y-axis and z-axis. If the coefficient of volumetric expansion of the solid is n×10−6/∘C then the value of n is....
06:51View SolutionIn an experiment on the specific heat of a metal, a 0.20 kg block of the metal at 150∘C is dropped in a copper calorimeter (of water equivalent 0.025 kg) containing 150 cm3 of water at 27∘C. The final temperature is 40∘C. Compute the specific heat of the metal. If heat losses to the surroundings are not negligible, is your answer greater or smaller than the actual value for specific heat of the metal ?
06:34View SolutionCentre Of Mass Of Uniform Semi Circular Disc
02:12View SolutionThe moment of inertia of a uniform semicircular disc of mass disc through the centre is
02:44View SolutionWhat will be the position of centre of mass of a half disc shown ?
 .02:36View Solution
.02:36View SolutionFind the centre of mass of a unifrom (a) half-disc,(b) quarter-disc.
12:20View SolutionFind moment of inertia of half disc of radius R2 and mass M about its centre. A smaller half disc of radius R1 is cut from this disc
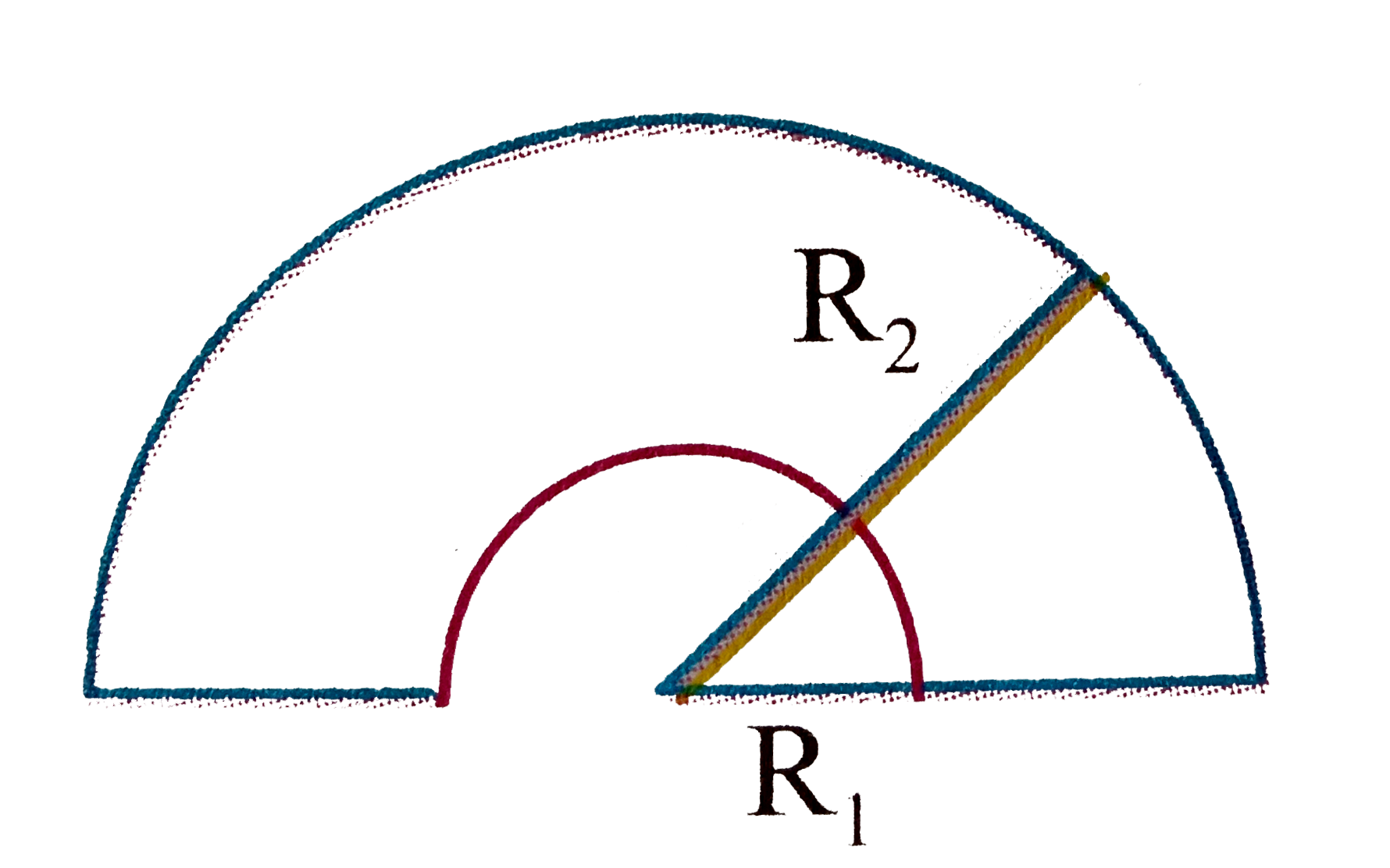 02:55View Solution
02:55View SolutionDetermine the coordinates of centre of mass of a half disc. Of mass M and radius R, assuming uniform mass disribution.
 View Solution
View SolutionFind the moment of inertia of a uniform half-disc about an axis perpendicular to the plane and passing through its centre of mass. Mass of this disc is M and radius is R.
05:48View SolutionStatement I : The centre of mass of a circular disc with uniform mass distribution lies always at the centre of the disc.
Statement II : Circular disc with uniform mass distribution is a symmetrical body.View Solution" Centre of mass for a half uniform ring and uniform half disc will be at respectively "
05:48View SolutionFind the centre of mass of a thin, uniform disc of radius R from which a small concentric disc of radius r is cut.
05:27View Solution

