Find the dimensions of
a. angular speed ω
angular acceleration α
torque τ and
d. moment of interia I.
. Some of the equations involving these quantities are ω=θ2−θ1t2−t1,α=ω2−ω1t2−t1,τ=F.randI=mr2
The symbols have standard meanings.
The correct Answer is:A, B, C, D
To find the dimensions of the given physical quantities, we will analyze each one step by step based on the provided equations.
Step 1: Find the dimensions of angular speed (ω)
Definition: Angular speed (ω) is defined as the rate of change of angle with respect to time.
Formula:
ω=θ2−θ1t2−t1
- The angle (θ) is a dimensionless quantity (it is measured in radians, which are unitless).
- Time (t) has the dimension of (T).
Calculation:
Dimension of ω=Dimension of angleDimension of time=1T=T−1
Step 2: Find the dimensions of angular acceleration (α)
Definition: Angular acceleration (α) is defined as the rate of change of angular speed with respect to time.
Formula:
α=ω2−ω1t2−t1
- We already found that the dimension of angular speed (ω) is (T^{-1}).
- Time (t) has the dimension of (T).
Calculation:
Dimension of α=Dimension of ωDimension of time=T−1T=T−2
Step 3: Find the dimensions of torque (τ)
Definition: Torque (τ) is defined as the product of force and the distance from the pivot point.
Formula:
τ=F⋅r
- The dimension of force (F) is (M L T^{-2}).
- The dimension of distance (r) is (L).
Calculation:
Dimension of τ=Dimension of F⋅Dimension of r=(MLT−2)⋅(L)=ML2T−2
Step 4: Find the dimensions of moment of inertia (I)
Definition: Moment of inertia (I) is defined as the mass times the square of the distance from the axis of rotation.
Formula:
I=m⋅r2
- The dimension of mass (m) is (M).
- The dimension of distance (r) is (L).
Calculation:
Dimension of I=Dimension of m⋅(Dimension of r)2=M⋅(L2)=ML2
Summary of Dimensions
- Angular speed (ω): T−1
- Angular acceleration (α): T−2
- Torque (τ): ML2T−2
- Moment of inertia (I): ML2
---
ω=θ2−θ1t2−t1
- Time (t) has the dimension of (T).
Dimension of ω=Dimension of angleDimension of time=1T=T−1
α=ω2−ω1t2−t1
- Time (t) has the dimension of (T).
Dimension of α=Dimension of ωDimension of time=T−1T=T−2
τ=F⋅r
- The dimension of distance (r) is (L).
Dimension of τ=Dimension of F⋅Dimension of r=(MLT−2)⋅(L)=ML2T−2
I=m⋅r2
- The dimension of distance (r) is (L).
Dimension of I=Dimension of m⋅(Dimension of r)2=M⋅(L2)=ML2
- Angular speed (ω): T−1
- Angular acceleration (α): T−2
- Torque (τ): ML2T−2
- Moment of inertia (I): ML2
Topper's Solved these Questions
- INTRODUCTION TO PHYSICSBOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Question for short Answer
4 Videos
- INTRODUCTION TO PHYSICSBOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Objective 2
3 Videos
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER
11 Videos
- KINETIC THEORY OF GASESBOOK - HC VERMA ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - All Questions
106 Videos
Similar Questions
Find the dimensions of
a. the specific heat capacity c,
b. the coeficient of linear expansion α and
c. the gas constant R
Some of the equations involving these quantities are
Q=mc(T2−T1),lt=l0[1+α(T2−T1)]andPV=nRT.
View SolutionFind the dimensional formulae of the following quantities:
a. the universal constant of gravitation G,
b. the surface tension S,
c. the thermal conductivity k and
d. the coeficient of viscosity η.
Some equation involving these quantities are
F=Gm1m2r2,S=ρgrh2,
Q=kA(θ2−θ1)tdandF=−ηAv2−v1x2−x1
where the symbols have their usual meanings.
View Solution
Knowledge Check
4t−1=3w−1
If in the equation above t≠1andw≠1, then t=A2w−1B2(w−1)Cw−2D2w
A torque T acts on a body of moment of inertia l rotating with angular speed ω. It will be stopped just after time
View SolutionA uniiform disc of radius r spins with angular velocity ω and angular acceleration α. If the centre of mass of the disc has linear acceleration a, find the magnitude and direction of aceeleration of the point 1,2, and 3.
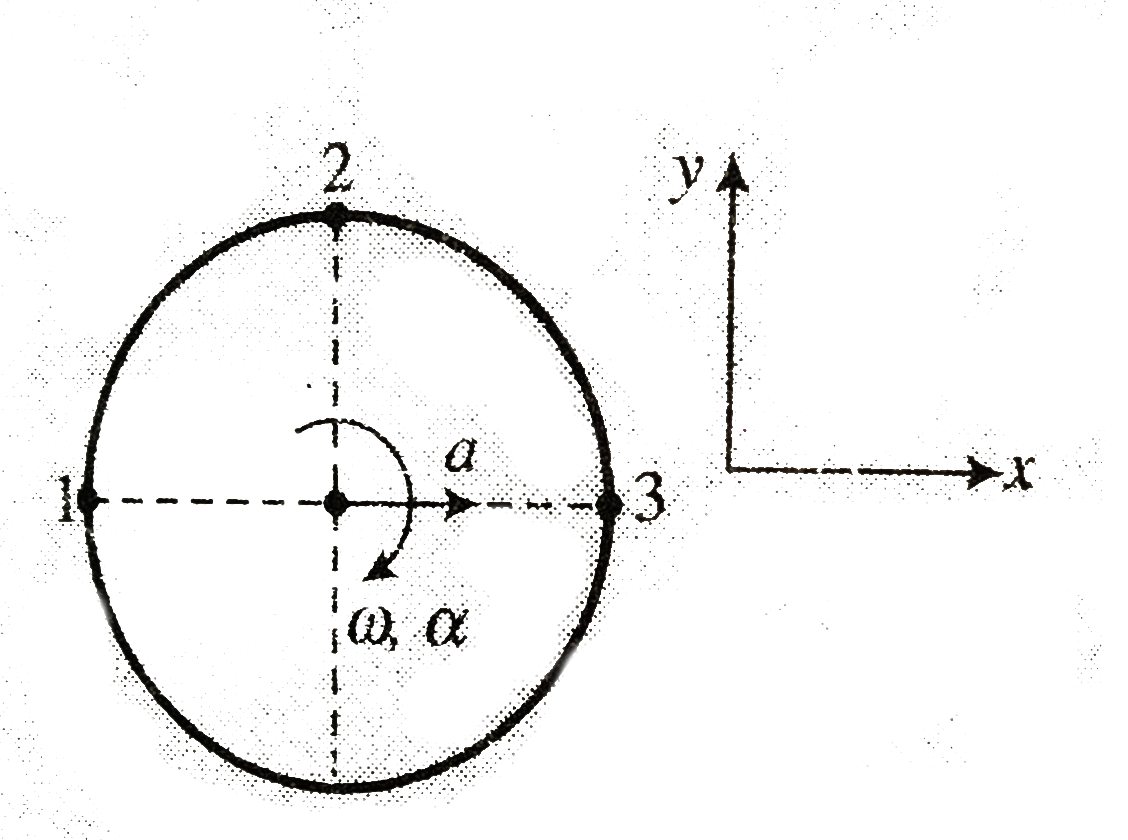
View SolutionIf I, α and τ are the moment of inertia, angular acceleration and torque respectively of a body rotating about an axis with angular velocity ω then,
View SolutionFind the dimensional formula of the following question :
(a) Surface tension T
(b) Universal constant of gravitation , G
(c ) Impulse ,J
(d) Torque τ
The equation involving these equations are :
T=FilF=Gm1m2r2,J=F×t and τ=F×1
View SolutionFind time period of the function, y=sinωt+sin2ωt+sin3ωt
View Solutionthe angular velocity omega of a particle varies with time t as ω=5t2+25rads. the angular acceleration of the particle att=1s is
View SolutionThe equations of two waves given as x=acos(ωt=δ) and y=acos(ωt+α), where δ=α+π2, then resultant wave represent:
View Solution
