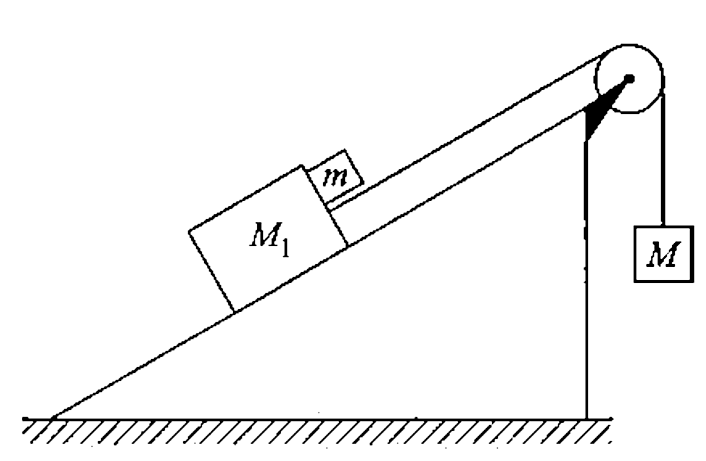
Find the mass M in the situation shown in figure-2.212 such that m remains at rest on the front surface of . The coefficient of friction between the front surface of and that of m is .
The correct Answer is:
Step by step video, text & image solution for Find the mass M in the situation shown in figure-2.212 such that m remains at rest on the front surface of M_(1). The coefficient of friction between the front surface of M_(1) and that of m is mu. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Advance MCQs
17 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - exercise 1.3
3 Videos
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Unsolved Numerical
63 Videos
Similar Questions
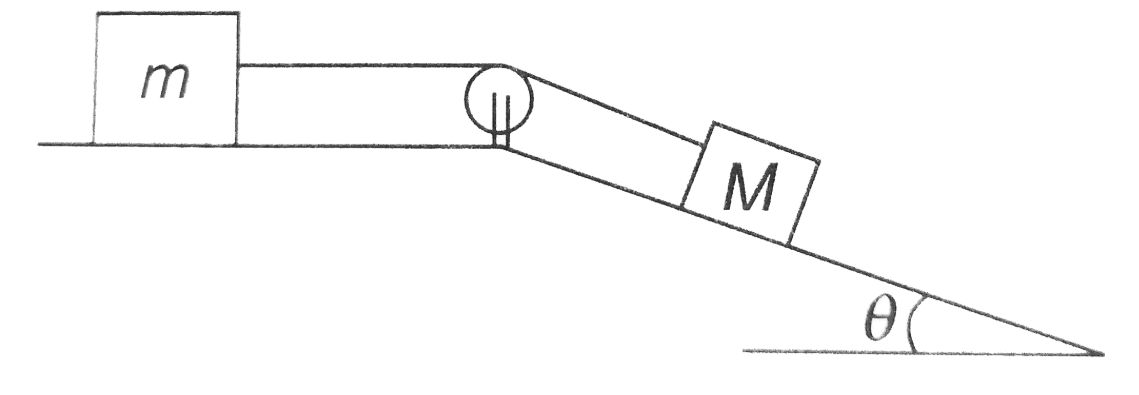
Find the maximum value of M//m in the situation shown in figure so that the system remains at rest. Friction coefficient at both the contacts μ. Discuss the situation when tanθ>μ
View SolutionFind the minimum value of horizontal force (F) required on the block of mass m to keep it at rest on the wall. Given the coefficient of friction between the surfaces is μ.

View Solution
Knowledge Check
A force F accelerates a block of mass m on horizontal surface. The coefficient of friction between the contact surface is . The acceleration of m will be -
AF−μmgMBzeroCmay be (A) or (B)DNone of theseA mass m is at rest under the action of a force F. as shown in the figure, on a horizontal surface. The coefficient of friction between mass and surface is u. Then, find the correct choice(s)
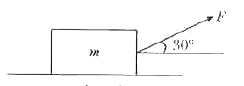 AThe force of friction between the mass and surface is F√3/2BThe force of friction between the mass and surface is μmg.CNormal force is (mg +F)DNormal force is (mg - F/2)
AThe force of friction between the mass and surface is F√3/2BThe force of friction between the mass and surface is μmg.CNormal force is (mg +F)DNormal force is (mg - F/2)A mass m rests under the action of a force F as shown in the figure-2.158 on a horizontal surface. The coefficient of friction between the mass and the surface is μ. The force of friction between the mass and the surface is :
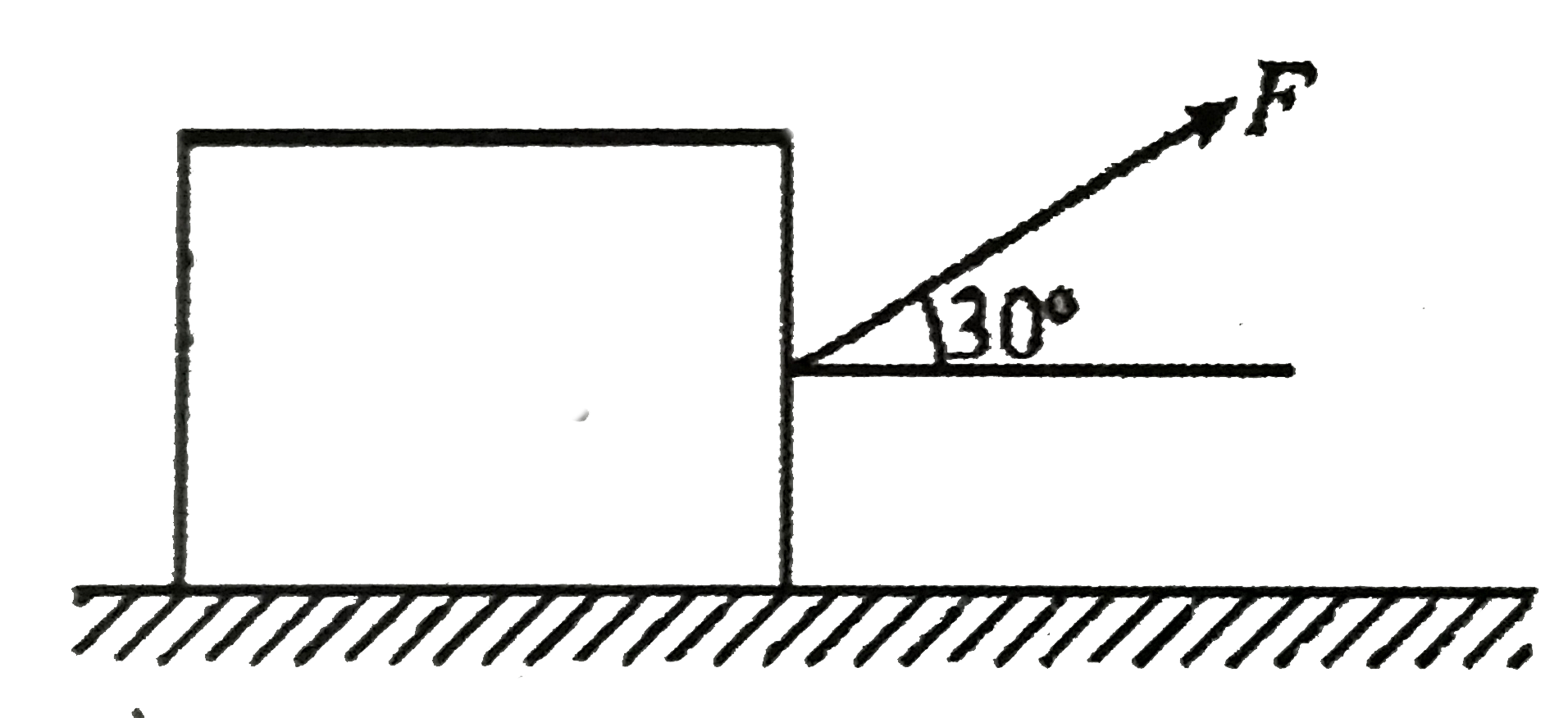 AμmgBμ[mg+F2]CF√32Dμ[mg−F2]
AμmgBμ[mg+F2]CF√32Dμ[mg−F2]
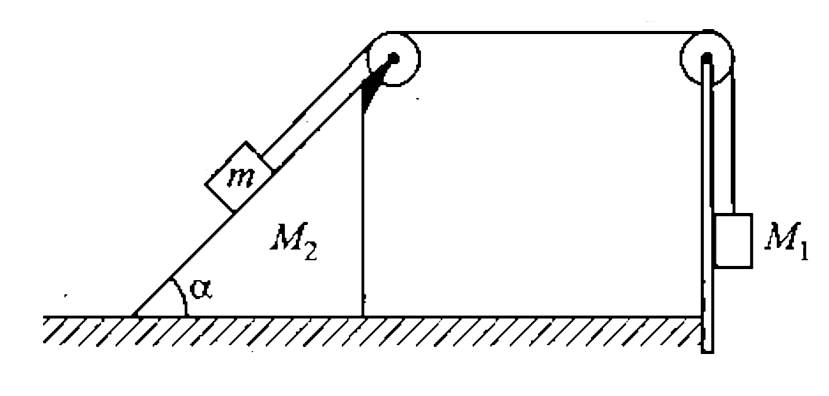
Find out the value(s) of θ of the inclined plane such that the mass m remains at rest on the wedge of mass M2 as shown in figure-2.215. Friction between the small block and the wedge plane is μ and all other surface are smooth.
View SolutionA block of mass m1 connected with another of mass m2 by a light spring of natural length l0 and stiiffness k is kept stationary on a rough horizontal surface . The coefficient of friction between m1 and surface is μ and the block m2 is smooth .The block m2 is moved with certain the block m1 in horizontal plane Find the (a) maximum to m1 and (b) acceleration of m2 in part(a)

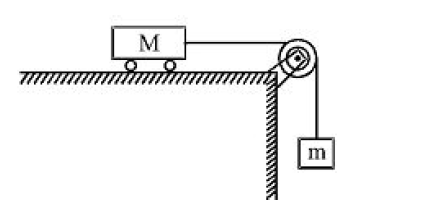
View SolutionA trolley of mass M is attached to a block of mass m by a string passing over a frictionless pulley as shown in the figure. If the coefficient of friction between the horizontal surface and wheels of trolley is μ, then acceleration of the block m and that of trolley M on releasing the system is :-
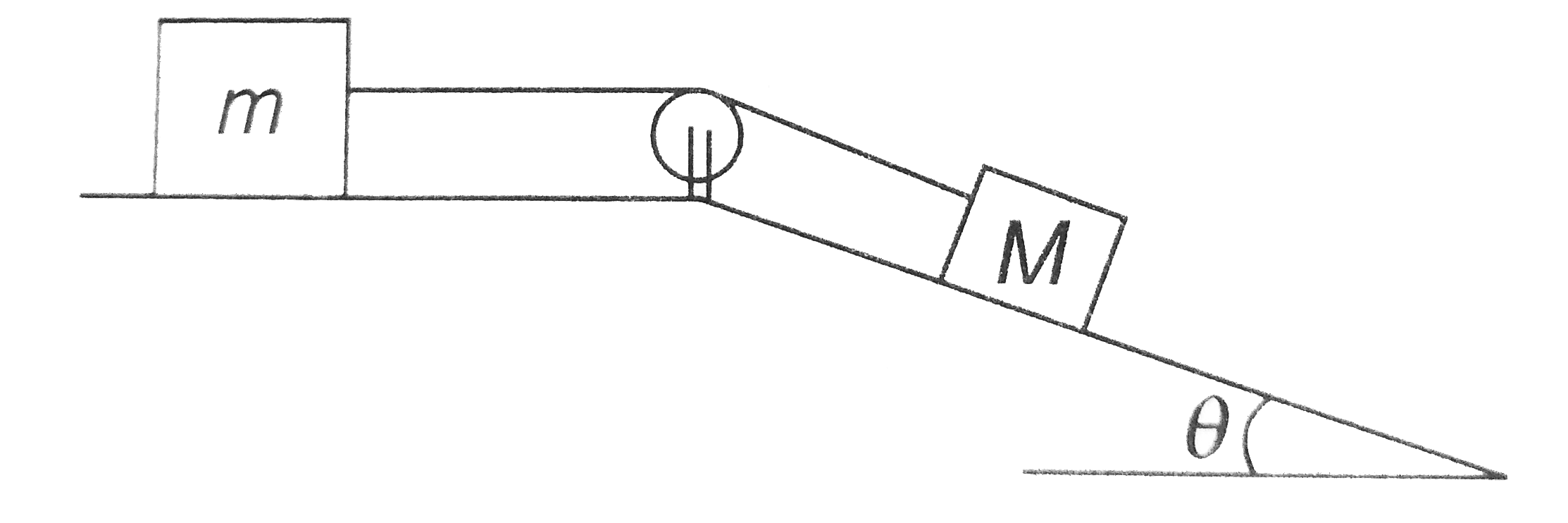
View SolutionFind the maximum value of (m//m) in the situation shown in figure so that the system remains at rest. Friction coefficient of both the contacts is μ , string is massless an pulley is friction less.
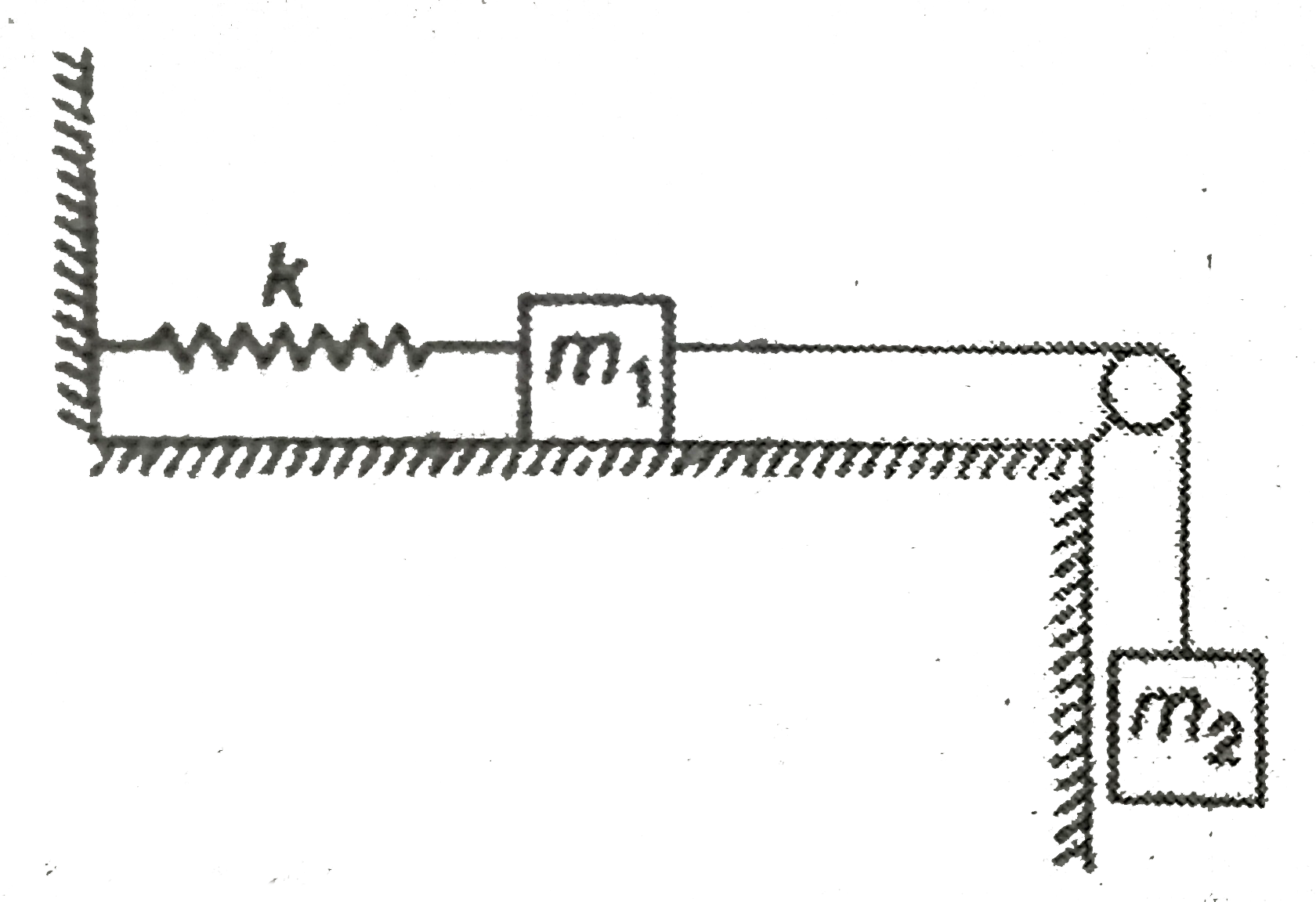
View SolutionIn the system as shown in figure, the blocks have masses m1 and m2, the spring constant is k, coefficient of friction between the block of mass m1 and the surface is μ. The system is released with zero initial speed from the position where the spring is in its natural length.
View Solution
