Find the relation between the group velocity u and phase velocity υ for the following disperison laws:
(a) υ∝1/√λ1,
(b) υ∝k,
(c ) υ∝1/ω2,
Here λ,k, and ω are the wavelength, wave number, and angular frequancy.
(a) v=a/√λ,a= constant
Then u=v−λdvdλ
=a√λ−λ(−12aλ−3/2)=32.a√λ=32v.
(b) v=bk=ωk,b= constant
so ω=bk2 and u=dωdk=2bk=2v.
(c) v=cω2,c= constant =ωk.
so ω3=ck or ω=c1/3k1/3
Thus u=dωdk=c1/313k−2/3=13ωk=12v
Topper's Solved these Questions
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Optics Of Moving Sources
22 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Thermal Equation
47 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Polarization Of Light
43 Videos
- MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems
92 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
Similar Questions
Find the resultant amplitude and the phase difference between the resultant wave and the first wave , in the event the following waves interfere at a point , y1=(3cm)sinωt,
y2=(4cm)sin(ωt+π2),y3=(5cm)sin(ωt+π)
View SolutionCheck by the method of dimensions, the formula uψlo=1λ√Kd, where υ is velocity of longitudinal waves, λs is wavelength of wave, K is coefficient of volume elasticity and d is density of the medium.
View Solution
Knowledge Check
The velocity υ of a particle as a function of its position (x) is expressed as υ=√c1−c2x, where c1 and c2 are positive constants. The acceleration of the particle is
Ac2B−c22Cc1−c2Dc1+c22Which of the following does not have the dimensions of velocity ? ( Given ε0 is the permittivity of free space , μ0 is the permittivity of free space , visequency,λ is wavelength , P is the pressure , and ρ is density , k is wave number , ω is the the angular frequency)
AωkBvλC1√ε0μ0D√PρStatement-1 : A body released from a height equal to the radius (R) of the earth. The velocity of the body when it strikes the surface of the earth will be √2gR.
Statement -2 : As υ2=u2+2 as.AStatement -1 is true , Statement -2 is true , Statement-2 is a correct explanation of Statement -1.BStatement -1 is true , Statement-2 is true , Statement-2 is a correct explanation of Statement-1.CStatement-1 is true , Statement-2 is false.DStatement-1 is false , Statement-2 is true.
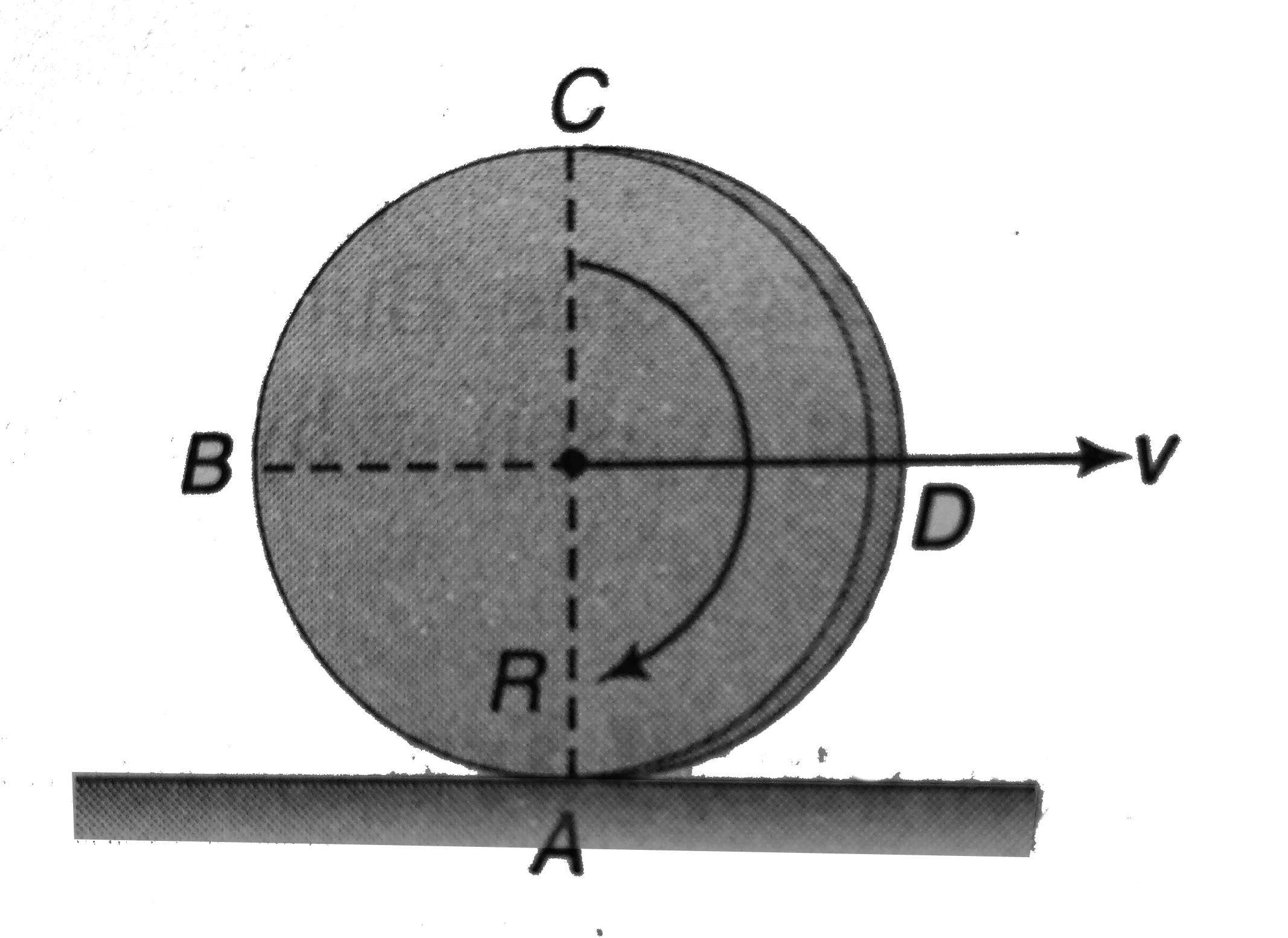
A disc of radius R has linear velocity v and angular velocity ω as shown in the figure. Given υ=rω find velocity of point A, B, C and D on the disc.
View SolutionUsing dimensional analysis, check the correctness of the following relations : (i) Snth=u+a2(2n−1) (ii) λ=h/mυ (ii) =mc2 where the symbole have their usual menaings.]
View SolutionA metallic ring of field. If z is the radius l (ring being horizontal is falling under gravity in a region haivng a magnetic field. If z is the vertical direction, the z-component of magnetic field is Bz=B0(1+λz). If R the resistance of the ring and if the ring falls with a velocity υ, find the energy lost in the resistance If the ring has reached a constant velocity, use the conservation of energy to determine υ in terms of m, B, λ and acceleration due to gravity g.
View SolutionIn a certain medium the relationship between the group and phase velocities of an electromagnetic wave has the form uv=c2, where c is the velocity of light in vaccume. Find the dependence of permittivity of that medium on wave frequency, ε(ω).
View SolutionK.E. of photo electron is E when incident frequency is λ1. It is 2E when incident frequency is λ2. The relation between the wavelength is
View Solution
