Find the total heat produced in the loop of the previous problem during the interval 0 to 30 s if the resistance of the loop is 4.5 mOmega.Solution in Gujrati
As heat product is a scalar property:
so, net heat produced
=Ha+Hb+Hc+Hd
R=42mΩ=405×10−3Ω
(a)e=3×10−4V
i=eR=3×10−44.5×10−3
=6.7×10−2Amp.
Ha=(6.7×10−2^2)×4.5×10−3×5
Hb=Hd=0
[Sinceemfis∈ducedfor5sec]
Hc=(6.7×10−2)2×4.5×10−2×5
So,→talheat
=Ha+Hc
=2×(6.7×10−2)2×4.5×10−3×5
=2×10−4J.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 2
10 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Exercises
75 Videos
- ELECTROMAGNETIC WAVESBOOK - HC VERMACHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercises
9 Videos
Similar Questions
Shown a metallic square frame of edga a in a vertical plane. A uniform magnetic field B exists in the space in a directon perpendicular to the plane of the figure. Two boys pull the opposite corners of the square ot deform it into a rhombus. They start pulling the corners t t=0 and sisplace the corners at a uniform speed u. (a) find the induced emf in the frame at the instant when the angles at these corners reduce to 60∘. (b) find the induced current in the frame at this instatnt if the total resistance of the frame is R. (c) Find the total charge which flows through a side of the frame by the time the square is deformed into a straight line.
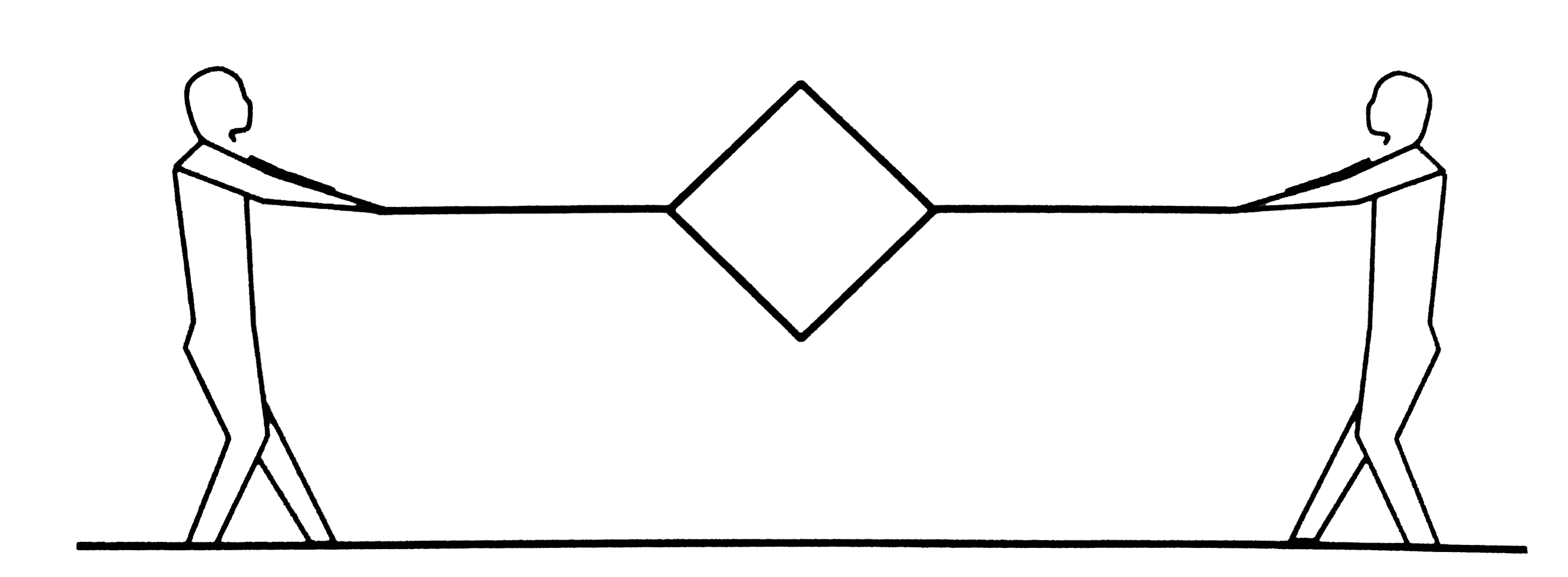
View SolutionA bar magnet is moved along the axis of a copper ring placed far away from the magnet. Looking from the side of the magnet, an anticlockwise current is found to be induced in the ring. Which of the following may be true?
View SolutionFind the dimensional formula of ε0 .
View SolutionShow that the dimensions of the displacement current (ε0dΦEdt) are that of an electric current.
View SolutionConsider the situation described in the previous problem. Suppose the current I enters the loop at the point A and leaves it at the point B. Find the magnetic field at the centre of the loop.
View SolutionFind the total heat produced in the loop of the previous problem during the interval 0 to 30 s if the resistance of the loop is 4.5 mOmega.
View SolutionA magnetic flux through a stationary loop with a resistance R varies during the time interval tau as phi=at(tau-t) . Find the amount of the generated in the loop during that time
View SolutionA stationary circular loop of radius a is located in a magnetic field which varies with time from t=0→t=T according to law B=B0t(T−t). If plane of loop is normal to the direction of field and resistance of the loop is R, calculate
amount of heat generated in the loop during this interval.
View SolutionA magentic flux through a statinary loop with a resistance R varies during the tiem interval τ as Φ=at(τ−t). Find the amount of heat generated in the loop during that time. The inductance of the loop is to be neglected.
View SolutionIn the previous problem, fin dthe total heat produced in the loop.
View SolutionFind the total heat produced in the loop of the previous problem during the interval 0 to 5s
View SolutionA triangle loop as shown in the figure is started to being pulled out at t=0 from a uniform magnetic field with a constant velocity v.Total resistance of the loop is constant and equals to R.Then the variation of power produced in the loop with time will be:
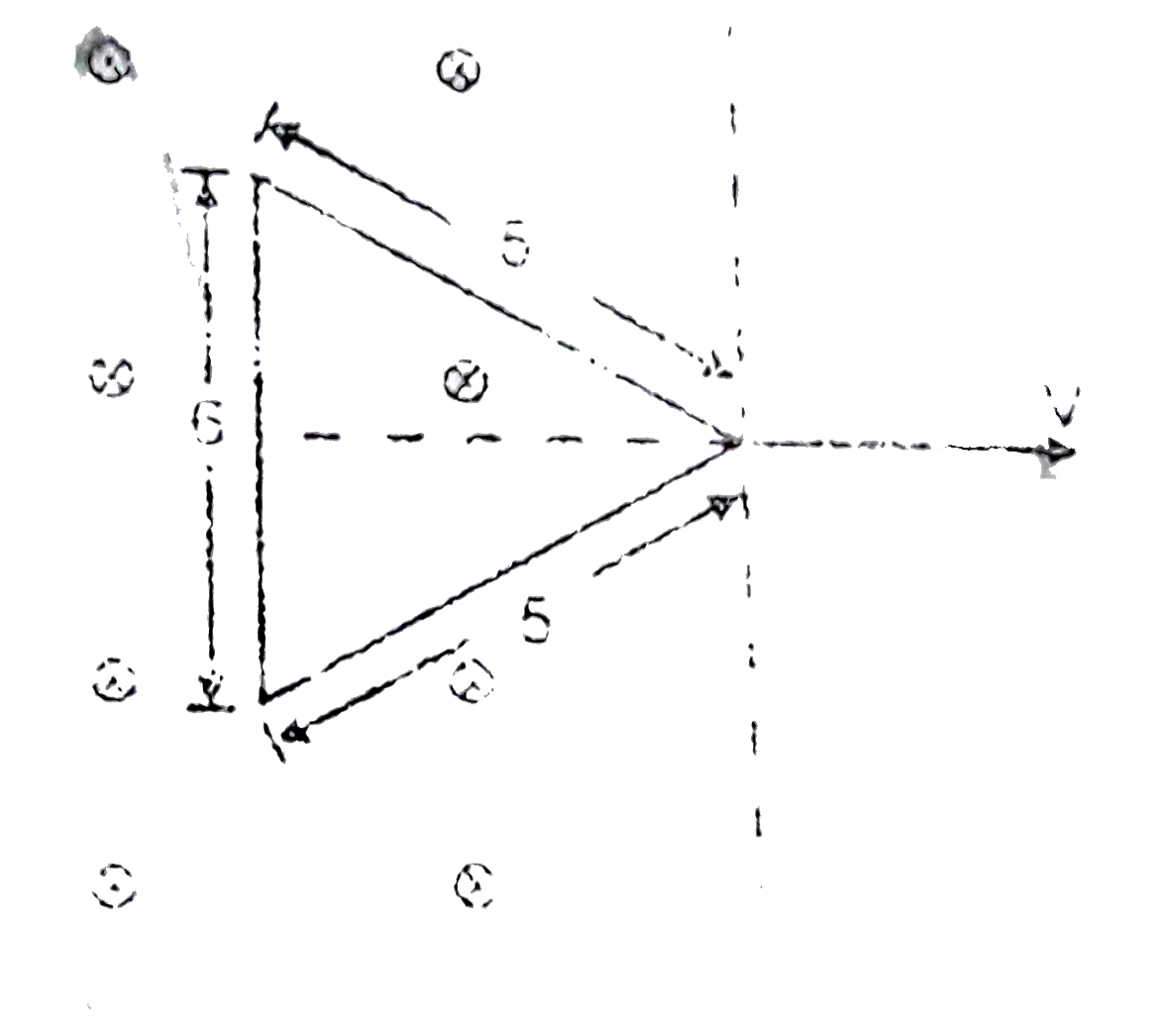
View SolutionFind the total heat produced in the loop of the previous problem during the interval 0 to 5s
View SolutionMagnetic flux through a stationary loop with a resistance R varies during the time interval tau" as "phi=alphat(tau-t) where alpha is a constant. Calculate the amount of heat generated in the loop during the time interval tau .
View SolutionThe magnetic flux through a stationary loop with resistance R varies during interval of time T as phi = at (T – t). The heat generated during this time neglecting the inductance of loop will be
View SolutionR प्रतिरोध वाले स्थायी लूप में से चुम्बकीय फ्लक्स ϕ=at(τ−t) के रूप में समय अन्तराल τ के दौरान परिवर्तित होता है। उस समय के दौरान लूप में उत्पन्न ऊष्मा की मात्रा क्या है?
View Solution
